filmov
tv
Constant Coefficient ODEs: Real & Distinct vs Real & Repeated vs Complex Pair
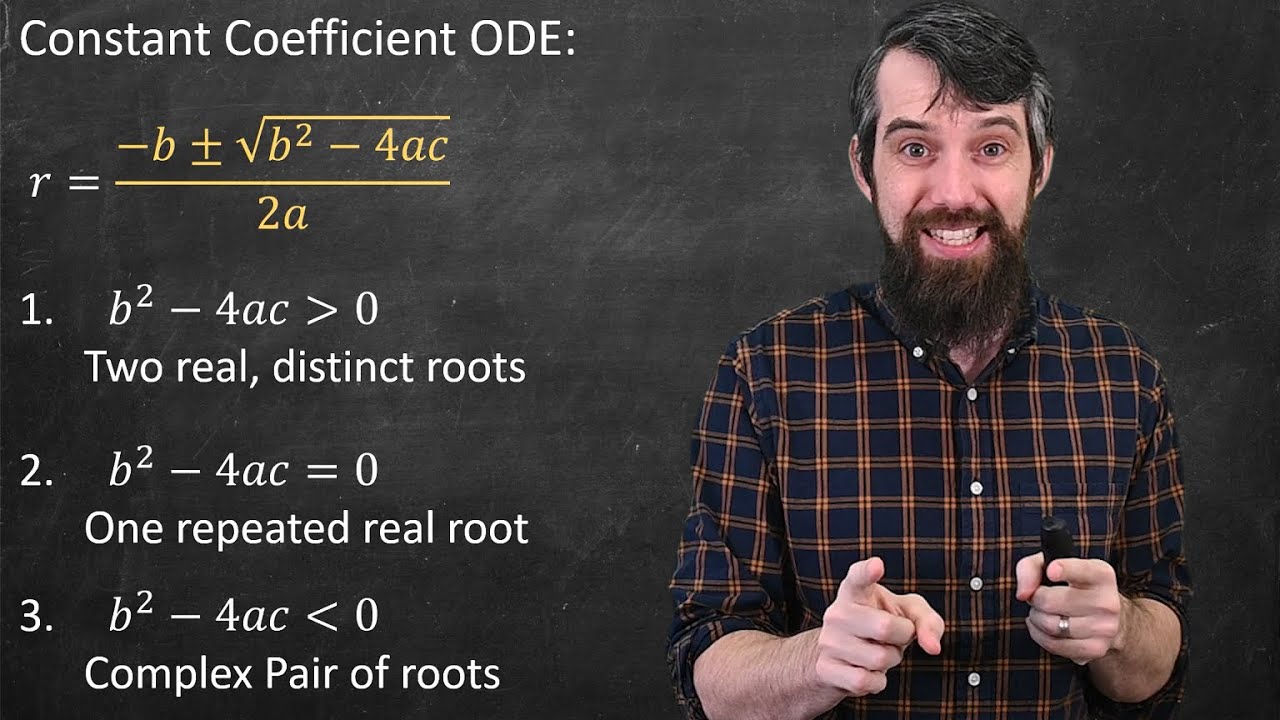
Показать описание
While we saw a specific example of Constant Coefficient Homogeneous ODEs in my previous video in the playlist, in this video we're going to solve it in generality and really elaborate on the three main cases. The characteristic equation aka auxiliary equation can have either real and distinct root, real and repeated roots, or complex conjugates. Each of these three cases has a specific form of the solution to the differential equation.
0:00 Characteristic Equation
1:50 Three Cases
4:00 Real Distinct Roots
4:24 Real Repeated Roots
6:19 Complex Roots
OTHER COURSE PLAYLISTS:
OTHER PLAYLISTS:
► Learning Math Series
►Cool Math Series:
BECOME A MEMBER:
MATH BOOKS & MERCH I LOVE:
SOCIALS:
How to Solve Constant Coefficient Homogeneous Differential Equations
Constant Coefficient ODEs: Real & Distinct vs Real & Repeated vs Complex Pair
Constant Coefficient ODEs: Example 1 with Real and Complex Roots
Constant Coefficient ODEs with Distinct Real Roots
Constant Coefficient ODEs: Example 1 with Distinct Real Roots
Constant Coefficient ODEs: Example 1 with Repeated Real Roots
Constant Coefficient ODEs Example 2 with Distinct Real Roots
Second order homogeneous linear differential equations with constant coefficients
Higher Order Constant Coefficient Differential Equations: y'''+y'=0 and y'&...
Homogeneous, second-order, constant coefficient ode with two real roots
Linear ODE with Constant Coefficients: Complex Roots with Nonzero Real Parts
Second Order Linear Differential Equations
Second-order, linear, homogeneous odes with constant coefficients (Part II: real, distinct roots)
Constant Coefficient ODEs: Example 1 with Distinct Complex Roots
Differential Equations | Homogeneous 2nd Order ODEs with constant coefficients| Distinct real roots
Undetermined Coefficients: Solving non-homogeneous ODEs
Constant Coefficient ODEs: Example 1 with Repeated Complex Roots
Differential Equations - 24 - 2nd Order - Complex Roots (r=a+bi)
2nd Order ODEs - Constant Coefficient | Definition & Worked examples: Real, Repeated & Compl...
Constant Coefficient ODEs with the Rational Root Theorem: Example 1
Constant Coefficient ODEs: Example 2 with Repeated Real Roots
Second Order Linear Constant Coefficient ODEs Part 1
Linear ODEs with Constant Coefficients: Repeated Roots
Homogeneous, second-order, constant-coefficient ode with complex roots
Комментарии
 0:06:41
0:06:41
 0:11:50
0:11:50
 0:04:22
0:04:22
 0:07:49
0:07:49
 0:05:02
0:05:02
 0:06:05
0:06:05
 0:02:59
0:02:59
 0:11:44
0:11:44
 0:11:19
0:11:19
 0:05:35
0:05:35
 0:07:39
0:07:39
 0:25:17
0:25:17
 0:05:06
0:05:06
 0:03:02
0:03:02
 0:05:41
0:05:41
 0:12:44
0:12:44
 0:04:17
0:04:17
 0:09:49
0:09:49
 0:06:46
0:06:46
 0:07:21
0:07:21
 0:04:39
0:04:39
 0:45:11
0:45:11
 0:08:03
0:08:03
 0:05:44
0:05:44