filmov
tv
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (8 of 35) Eigenvector=? of a 3x3 Matrix

Показать описание
In this video I will find eigenvector=? given a 3x3 matrix and an eigenvalue.
Next video in this series can be seen at:
Linear transformations and matrices | Chapter 3, Essence of linear algebra
Linear Algebra 3.1.1 Introduction to Determinants
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (1 of 35) What Are Eigenvalues? (Part 1)
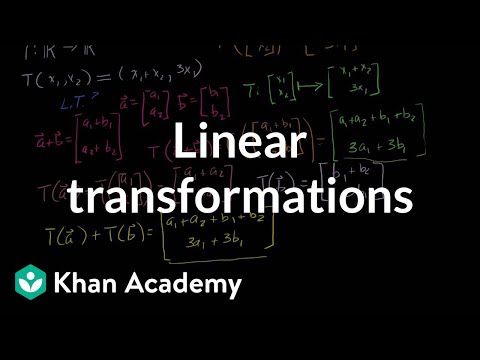
Linear transformations | Matrix transformations | Linear Algebra | Khan Academy
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (8 of 35) Eigenvector=? of a 3x3 Matrix
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (3 of 35) What Are Eigenvalues? (Example 1)
Linear algebra Ex 3.1(lecture 1)Part a Ba/ Bsc 6th sem
Vectors | Chapter 1, Essence of linear algebra
Ch 3 Linear Regression | Exercise 3.3 (Q6 - Q.14) | Class 12 HSC Commerce | Maths 2 @GalaxyofMaths
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (7 of 35) Given the Eigenvector, Eigenvalues=?
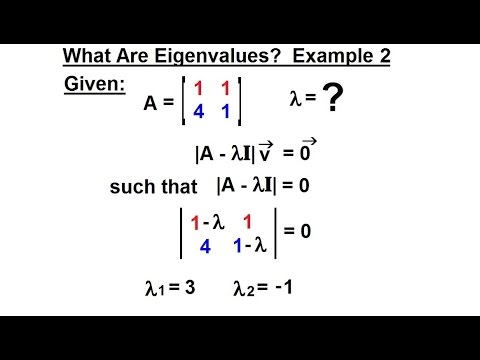
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (4 of 35) What Are Eigenvalues? (Example 2)
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (13 of 35) Basis=? for a 3x3 Matrix: Ex. 2
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (29 of 35) Power Method A^3
Three-dimensional linear transformations | Chapter 5, Essence of linear algebra
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (30 of 35) Power Method A^n, A^5
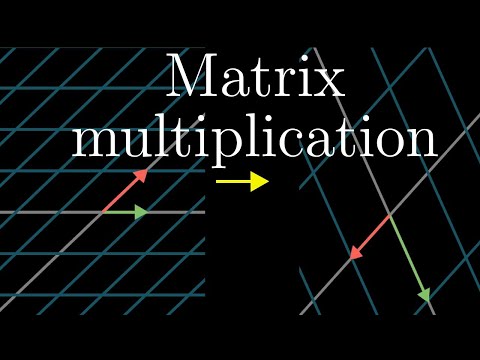
Matrix multiplication as composition | Chapter 4, Essence of linear algebra
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (10 of 35) Bases and Eigenvalues: 2
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (31 of 35) Power Method A^n, General Eqn.***
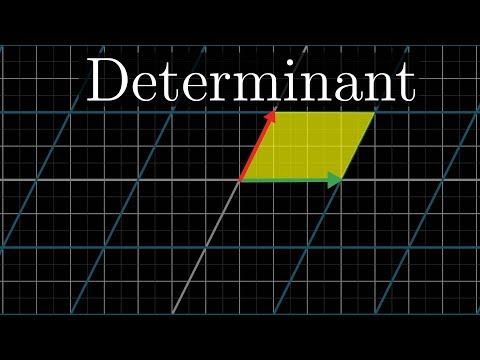
The determinant | Chapter 6, Essence of linear algebra
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (2 of 35) What Are Eigenvalues? (Part 2)
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (12 of 35) Basis=? for a 3x3 Matrix: Ex. 1
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (27 of 35) Diagonalization (Part 3/3)
Linear Algebra: Ch 3 - Eigenvalues and Eigenvectors (25 of 35) Diagonalization (Part 1/3)
Inverse matrices, column space and null space | Chapter 7, Essence of linear algebra
Комментарии
 0:10:59
0:10:59
 0:12:52
0:12:52
 0:05:20
0:05:20
 0:13:52
0:13:52
 0:08:44
0:08:44
 0:02:42
0:02:42
 0:21:24
0:21:24
 0:09:52
0:09:52
 1:01:44
1:01:44
 0:03:53
0:03:53
 0:02:51
0:02:51
 0:04:04
0:04:04
 0:04:33
0:04:33
 0:04:46
0:04:46
 0:06:42
0:06:42
 0:10:04
0:10:04
 0:05:23
0:05:23
 0:05:07
0:05:07
 0:10:03
0:10:03
 0:03:56
0:03:56
 0:05:16
0:05:16
 0:03:36
0:03:36
 0:07:02
0:07:02
 0:12:09
0:12:09