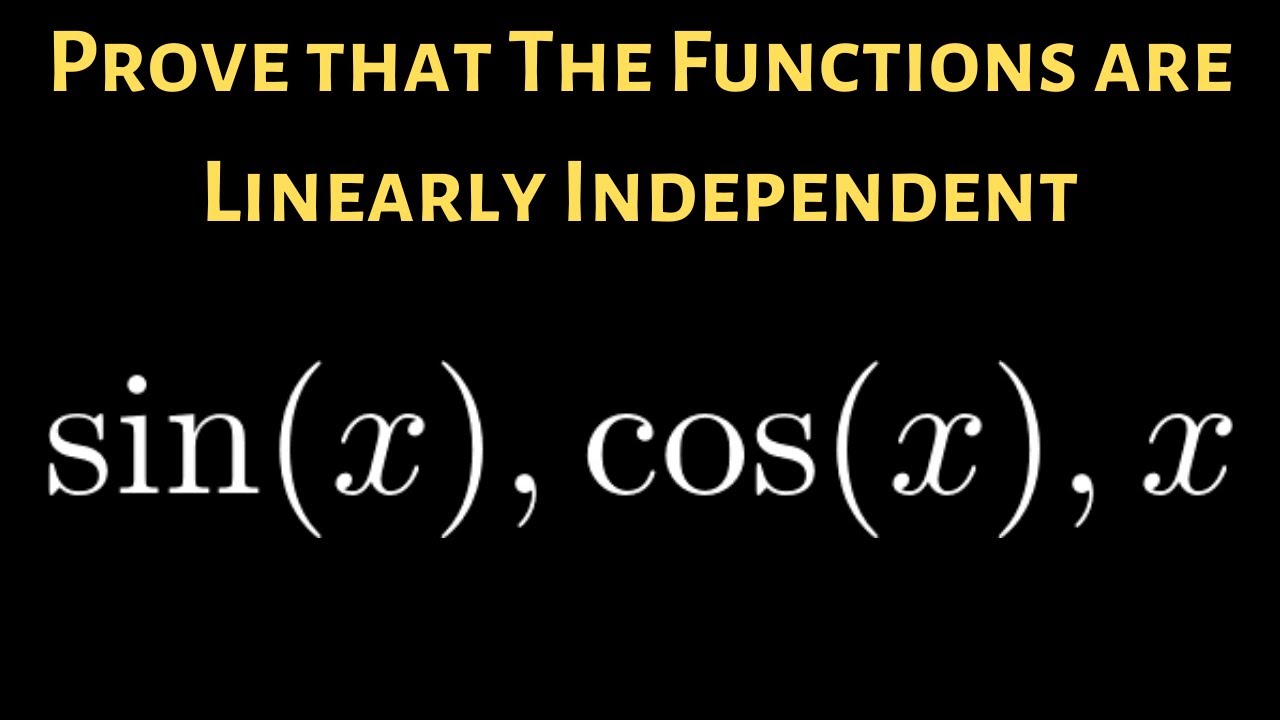
Prove that the Functions sin(x), cos(x), and x are Linearly Independent over the set of Real Numbers

preview_player
Показать описание
Prove that the Functions sin(x), cos(x), and x are Linearly Independent over the set of Real Numbers

If you enjoyed this video please consider liking, sharing, and subscribing.

There are several ways that you can help support my channel:)

************Udemy Courses(Please Use These Links If You Sign Up!)*************
Abstract Algebra Course

Advanced Calculus Course

Calculus 1 Course

Calculus 2 Course

Calculus 3 Course

Calculus Integration Insanity

Differential Equations Course

College Algebra Course

How to Write Proofs with Sets Course

How to Write Proofs with Functions Course

Statistics with StatCrunch Course

Math Graduate Programs, Applying, Advice, Motivation

Daily Devotionals for Motivation with The Math Sorcerer

Thank you:)
  1. The Math Sorcerer
  2. linearly independent
  3. prove the functions are linearly independent
  4. prove linearly independent over years
  5. use the definition of linear independence
  6. proof
Рекомендации по теме
Proof of the 0:05:52

Proof of the derivative of sin(x) | Derivatives introduction | AP Calculus AB | Khan Academy

Prove that the 0:05:42

Prove that the Functions sin(x), cos(x), and x are Linearly Independent over the set of Real Numbers

Proof of the 0:04:57

Proof of the Derivative of Sinx

prove that sinx 0:04:30

prove that sinx is continuous function

Derivative of sin(x) 0:09:39

Derivative of sin(x) from First Principles

Derivative of sin(x) 0:09:18

Derivative of sin(x) and cos(x), PROOF

Derivative of Sine 0:10:30

Derivative of Sine and Cosine Functions | Calculus

The most important 0:11:54

The most important limit in Calculus // Geometric Proof & Applications

Integral of arcsin(x) 0:03:22

Integral of arcsin(x) with Integration by Parts | Calculus 2 Exercises

Differentiating sin(x) from 0:09:02

Differentiating sin(x) from First Principles

Visualizing the derivative 0:00:59

Visualizing the derivative of sin(x)

Proof of angle 0:08:26

Proof of angle addition formula for sine | Trigonometry | Khan Academy

Proof: Derivative of 0:09:39

Proof: Derivative of Sin is Cos (Version 2)

derivative of sin(x) 0:07:32

derivative of sin(x) by using the definition of derivative

Establishing the Derivatives 0:17:08

Establishing the Derivatives of sin x, cos x & tan x

Limit of sin(x)/x: 0:05:27

Limit of sin(x)/x: Detailed Explanation and Proof

Derivative of Sine 0:07:49

Derivative of Sine X = Cos X || Differentiate Sin X || Proof in Calculus Math

❖ Taylor / 0:05:51

❖ Taylor / Maclaurin Series for Sin (x) ❖

Derivative of Sin(x) 0:05:20

Derivative of Sin(x) Proof (Using the Limit Definition)

Visual Calculus: Derivative 0:03:08

Visual Calculus: Derivative of sin(θ) is cos(θ)

Limit of sin(x)/x 0:09:16

Limit of sin(x)/x as x approaches 0 | Derivative rules | AP Calculus AB | Khan Academy

Integral of sin(x) 0:00:23

Integral of sin(x)

Complex definitions of 0:05:16

Complex definitions of sine and cosine

Does sin¯¹(sin x) 0:07:34

Does sin¯¹(sin x) = x?

Комментарии
Автор

Thank you so much... I was looking everywhere for an explanation online and I found the best one here, right on your channel!

CoolRockstar
Автор

What will happen if we put h(x)= tanx in the set of these given functions.
Is it L.D. or L.I. ?

jalajshah
Автор

Not a proof by any measure..you have to verify for any general value of x over the set of real numbers

pranjalagarwal
Автор

I'm confused on this style of proof. How does this prove that c1 = c2 = c3 = 0 for ALL x in R, when you only selected a few values of x for the example? There are infinitely many more possible values of x to be chosen? If that makes sense ...

jonesThePirate_huker
Автор

Helo sir sinx, cosx, sinx-cosx which mean linearly depent or lineraly independent sir pls clarification

gunaharish
Автор

hey can u solve it with the help of matrix??

arnavchauhan
Автор

Sir for your kind knowledge I wanna tell you that cos pie is not equal to zero. It's -1 . Practice first then upload.

sagarpundir
Автор

The value of cosπ=-1 . U have put wrong value.

deepakmodi