filmov
tv
Lagrange Multipliers PART 2/2 (KristaKingMath)
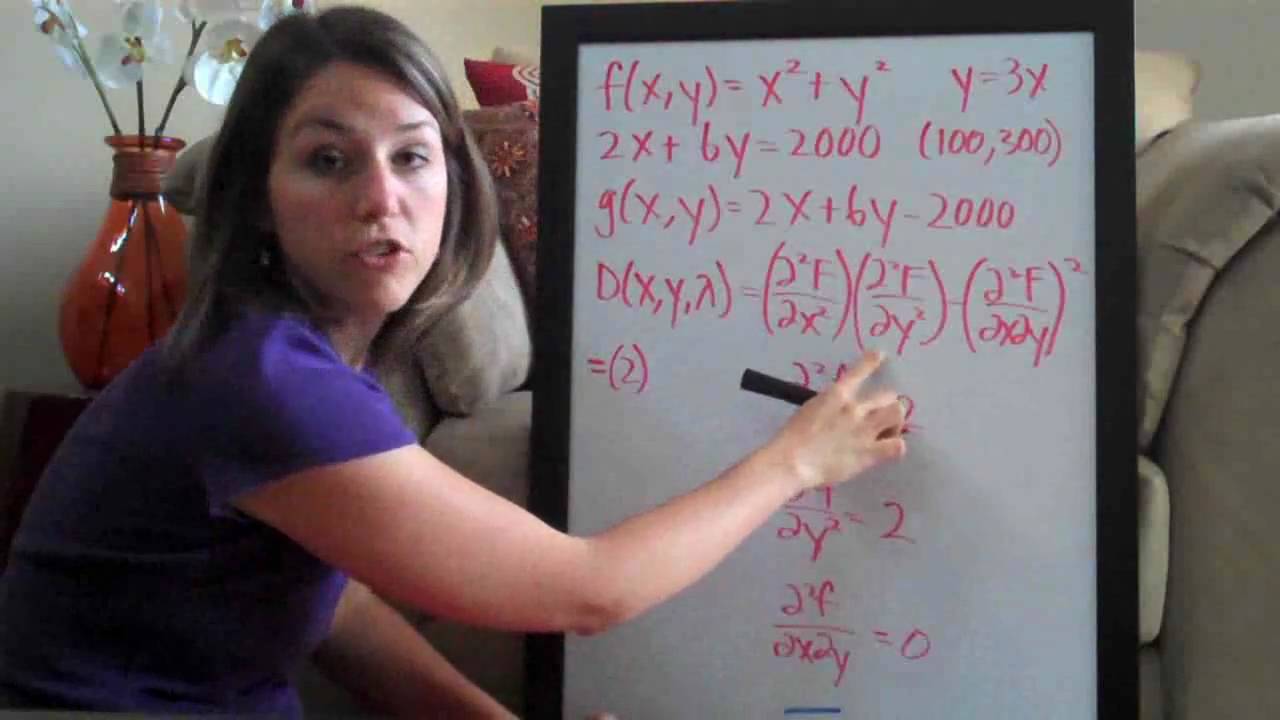
Показать описание
Lagrange Multipliers calculus example.
● ● ● GET EXTRA HELP ● ● ●
● ● ● CONNECT WITH KRISTA ● ● ●
Hi, I’m Krista! I make math courses to keep you from banging your head against the wall. ;)
Math class was always so frustrating for me. I’d go to a class, spend hours on homework, and three days later have an “Ah-ha!” moment about how the problems worked that could have slashed my homework time in half. I’d think, “WHY didn’t my teacher just tell me this in the first place?!”
Lagrange Multipliers PART 2/2 (KristaKingMath)
Lagrange Multipliers Example 2 PART 2/2 (KristaKingMath)
Lagrange Multipliers Example 2 PART 1/2 (KristaKingMath)
Lagrange Multipliers PART 1/2 (KristaKingMath)
Lagrange Multipliers, example 2
Constrained Optimization - Lagrange Multipliers Part 2 of 2
Lagrange multiplier example, part 2
Lagrange Multipliers - Part 2
Lagrange multipliers in three dimensions with two constraints (KristaKingMath)
Multivariable Calculus: Lagrange Multipliers, Part 2
Lagrange Multipliers - Two Constraints - Part 2
Lagrange Multipliers Part 2
Lagrange Multipliers with TWO constraints | Multivariable Optimization
lagrange multipliers, three dimensions one constraint (KristaKingMath)
12.9 Lagrange Multipliers, Part 2
Lagrange Multipliers (Example 2)
Lagrange Multipliers, Part Two
Lagrange Multipliers
UPenn Math 114 Lagrange Multiplier Method Example #2
LM5: Lagrange Multipliers with Two Constraints
Multivariable calculus 2.6.2: Lagrange multipliers
Lagrange Multipliers Example 2
'Lagrange Multipliers, Part 2' | Multivariable Calculus with Educator.com
An Introduction to Lagrange Multipliers Example 2 Optimize f(x,y)=3x+y constraint to x^2+y^2=10
Комментарии
 0:07:27
0:07:27
 0:08:05
0:08:05
 0:09:49
0:09:49
 0:09:26
0:09:26
 0:06:57
0:06:57
 0:11:36
0:11:36
 0:06:51
0:06:51
 0:05:02
0:05:02
 0:14:54
0:14:54
 0:11:36
0:11:36
 0:02:16
0:02:16
 0:18:52
0:18:52
 0:16:29
0:16:29
 0:08:33
0:08:33
 0:20:24
0:20:24
 0:07:14
0:07:14
 0:09:27
0:09:27
 0:33:46
0:33:46
 0:12:41
0:12:41
 0:10:24
0:10:24
 0:12:14
0:12:14
 0:06:23
0:06:23
 0:11:36
0:11:36
 0:06:44
0:06:44