Lagrange Multipliers with TWO constraints | Multivariable Optimization

Now we are upgrading to the case of optimizing with two constraints. We will look at how to interpret the lagrange multiplier method geometrically for two constrains, and then see a full example. We will also look at the geometry of the special case of optimization function: the distance.

0:00 Intro
0:38 Lagrange Multiplier Method
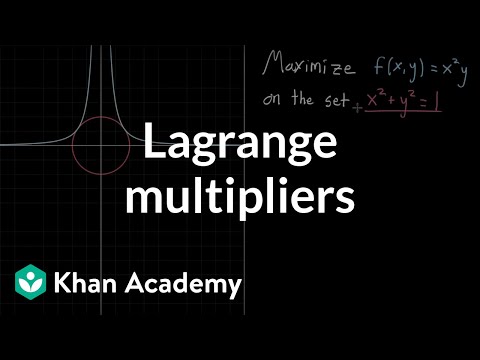
4:50 Example
12:30 Visulization

Click Multivariable Calculus playlist below for the rest of the series.

****************************************************
COURSE PLAYLISTS:

***************************************************

► Want to learn math effectively? Check out my "Learning Math" Series:

►Want some cool math? Check out my "Cool Math" Series:

****************************************************

*****************************************************

This video was created by Dr. Trefor Bazett. I'm an Assistant Teaching Professor at the University of Victoria.

BECOME A MEMBER:

MATH BOOKS & MERCH I LOVE:

[SolaceAndBane]

The only guy on YouTube who gives an explanation for the expanded Lagrange Multiplier, even my professor just threw the formula out and told us to use it

[tasninnewaz]

I love Trefor for Math and his personality.

[joaomattos]

I've watched many classes on youtube and I can say that Professor Trefor's classes stand out. Simply awesome!

[atakan]

i wish all classes were like this, all we need is just a touch of intuition and visualization to set the concepts clear in our mind!

[davidcooper]

Wikipedia is great for 1 constraint, but I absolutely needed Dr. Bazett for 2 constraints. Thanks so much.

[arrow]

This course/playlist is extremely great, wish I found it earlier, now my exam is tomorrow itself 😕

[devashishshah]

Your explanation, math, handwriting, 3d graphs.... all are super good

[nguyentuanminh]

What an awesome explainations and cool visualization. Thanks you Prof, keep doing.

[fernandojackson]

Excelent, as usual. Why not just find the intersection of the two constraints and use the standard method on that intersection?

[briandwi]

That was beautiful.
I suddenly noticed while watching the video that I too was wearing a checked shirt! Morphing into Dr Trefor!

[rajat]

Thanks man ...you just made my life easier...gr8 work..

[arinoba]

Nice energy and even better teaching! I also found that website and seeing it here makes me happy :D

[cesarnunezrios]

Dr. you are amazing! You just earned a new follower. This video really helped me

[FranFerioli]

11:50 How satisfying when you catch the Professor making a clerical sign mistake.
11:58 How disappointing when such clerical sign mistake gets squared off leaving the correct result 😁
Great video as usual!

[srikanthk]

Hi Trefor, you made it look easy. Thank you👍 I didn't understand why grad f is a linear combination of gradients of the two constraints. Shouldn't grad f be perpendicular to the line of intersection of constraints? Can't one find the gradient of the intersection line and then proceed the same way as Lagrange multiplier case for a single constraint?

[josecarlosferreira]

Thank you man! You are very helpful =D

[crimfan]

Zed's dead, baby, Zed's dead. ;)

Nicely done. I really like the visualizations, too. I'll have to check out the software you mentioned in one of these vids.

[nirajgujarathi]

thanks professor, it is really great explanation !

[NubaPrincigalli]

Thanks for the excelent content! Found a small typo: at 11:50, it should be f(-3, 0, -3), not f(-3, 0, 3), as z was squared the typo went unnoticed.. :)

[sayanjitb]

Wow, this kinda interpretation is pretty handsome.
Dear sir
I had a question, i have seen in some places circle is indicated as S1 and sphere in 3d as S2. What do they mean anyway?
TIA