Matrix Determinant Properties Example #2 - Linear Algebra Example Problems -

Показать описание

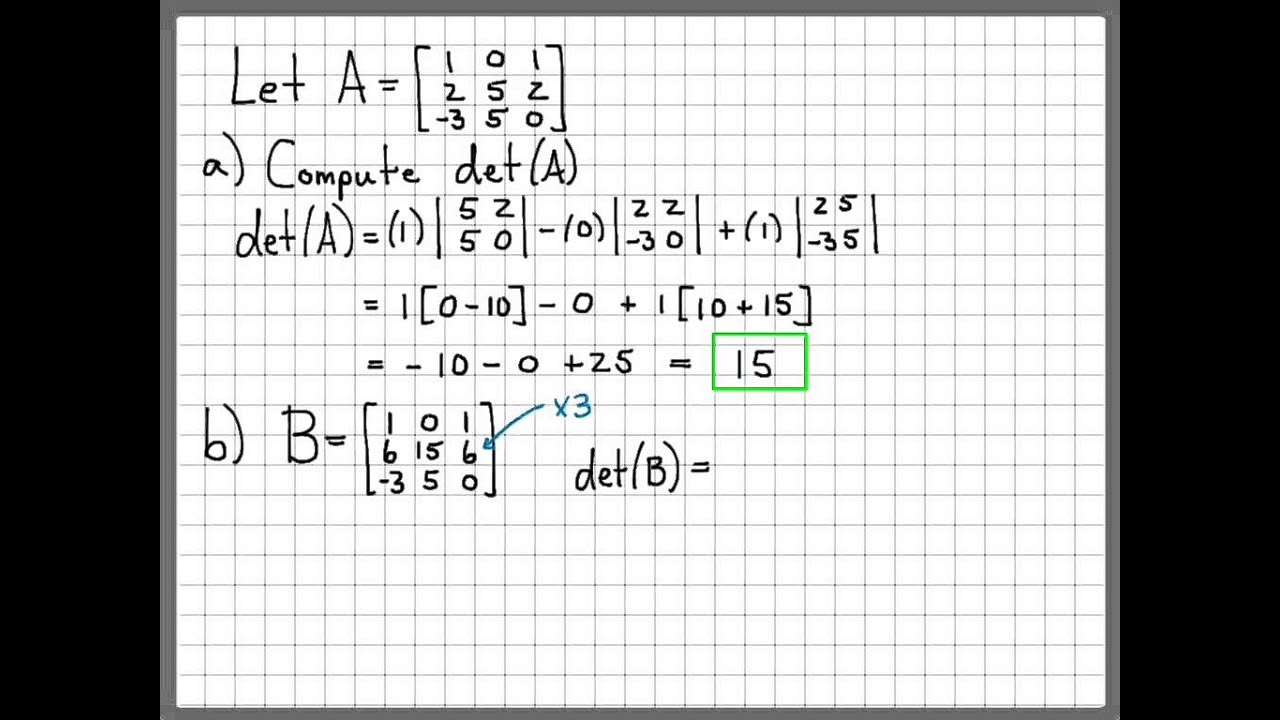
Consider the square matrices A and B, where B can be obtained from A by replacing one row of A with k times the row. If det(A) is known, det(B) can be easily computed by just multiplying by k, i.e. det(B) = k*det(A). Each time a row is multiplied by a constant k, the det(A) changes by a factor of k.

This videos shows a computational example that demonstrate this matrix determinant property. This video does not PROVE this general result, it just demonstrates the property.