filmov
tv
Matrix Determinant Properties Example #3 - Linear Algebra Example Problems

Показать описание
Consider the square matrices A and B, where B is the same as A except one row has been been replaced with a linear combination of rows from A. In this case, we have that det(A) = det(B). In general, replacing a row of a matrix with a linear combination of other rows does not change the value of the matrix determinant.
This video does not prove this result, but demonstrates the property with a simple example.
 0:03:44
0:03:44
 0:04:56
0:04:56
 0:05:53
0:05:53
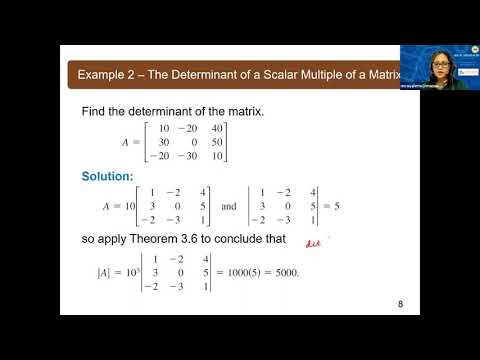 0:08:13
0:08:13
 0:12:15
0:12:15
 0:07:10
0:07:10
 0:03:24
0:03:24
 0:05:44
0:05:44
 2:12:40
2:12:40
 0:01:13
0:01:13
![[Linear Algebra] Properties](https://i.ytimg.com/vi/IjMxFP8AtpA/hqdefault.jpg) 0:11:58
0:11:58
 0:10:17
0:10:17
 0:02:07
0:02:07
 0:11:36
0:11:36
 0:01:01
0:01:01
 0:05:31
0:05:31
 0:00:18
0:00:18
 0:08:00
0:08:00
![8]Properties of Determinant](https://i.ytimg.com/vi/vLkQmdT45N8/hqdefault.jpg) 0:07:19
0:07:19
 0:22:06
0:22:06
 0:02:12
0:02:12
 0:10:03
0:10:03
 0:01:00
0:01:00
 0:25:16
0:25:16