filmov
tv
Properties of Determinants - Linear Algebra

Показать описание
In this video we will learn some properties of determinant of a matrix.
Properties of Determinants of Matrices:
Determinant evaluated across any column or row is same.
If all the elements of a row (or column) are zeros, then the value of the determinant is zero.
Determinant of a Identity matrix is 1.
If rows and columns are interchanged then value of determinant remains same (value is the same). Therefore, det(A) = det(A^T), here A^T is transpose of matrix A.
If any two row (or two column) of a determinant are interchanged the value of the determinant is multiplied by -1.
If all elements of a row (or column) of a determinant are multiplied by some scalar number k, the value of the new determinant is k times of the given determinant.
If two rows (or columns) of a determinant are identical the value of the determinant is zero.
Let A and B be two matrix, then det(AB) = det(A)*det(B).
Determinant of Inverse of matrix is equal to inverse of the original matrix
Properties of Determinants of Matrices:
Determinant evaluated across any column or row is same.
If all the elements of a row (or column) are zeros, then the value of the determinant is zero.
Determinant of a Identity matrix is 1.
If rows and columns are interchanged then value of determinant remains same (value is the same). Therefore, det(A) = det(A^T), here A^T is transpose of matrix A.
If any two row (or two column) of a determinant are interchanged the value of the determinant is multiplied by -1.
If all elements of a row (or column) of a determinant are multiplied by some scalar number k, the value of the new determinant is k times of the given determinant.
If two rows (or columns) of a determinant are identical the value of the determinant is zero.
Let A and B be two matrix, then det(AB) = det(A)*det(B).
Determinant of Inverse of matrix is equal to inverse of the original matrix
Properties of determinants of matrices | Lecture 31 | Matrix Algebra for Engineers
[Linear Algebra] Properties of Determinants
Properties of Determinants - Linear Algebra
The determinant | Chapter 6, Essence of linear algebra
Linear Algebra 3.2.1 Properties of Determinants
Properties of Determinants
Properties of Determinants (Linear Algebra)
Properties of Determinants 1
✅▶ DETERMINANT of a MATRIX by applying PROPERTIES
Linear Algebra Ch 3.2 Properties of Determinants # 1
Determinant of 3x3 Matrices, 2x2 Matrix, Precalculus Video Tutorial
3.2 - Properties of Determinants
Properties of Determinants
Lec-7: Properties Of Determinants | Linear Algebra
Properties of Determinants (Row Operations)
Linear Algebra Lecture 12 | Properties of Determinants
Linear Algebra: Properties of Determinants (Sec. 3.3)
Properties of Determinants - Linear Algebra - Part 3
What is a determinant?
Properties of Determinants 2
Linear Algebra 6.3 - Properties of Determinants (1 of 5)
Linear Algebra 2.3 Properties of Determinants; Cramer’s Rule
Properties of Determinants | Mathematics
2.3 - Properties of Determinants; Cramer’s Rule (Part 1)
Комментарии
 0:15:21
0:15:21
![[Linear Algebra] Properties](https://i.ytimg.com/vi/IjMxFP8AtpA/hqdefault.jpg) 0:11:58
0:11:58
 0:12:15
0:12:15
 0:10:03
0:10:03
 0:25:16
0:25:16
 0:09:56
0:09:56
 0:11:26
0:11:26
 0:02:12
0:02:12
 0:04:56
0:04:56
 0:14:37
0:14:37
 0:10:17
0:10:17
 0:19:10
0:19:10
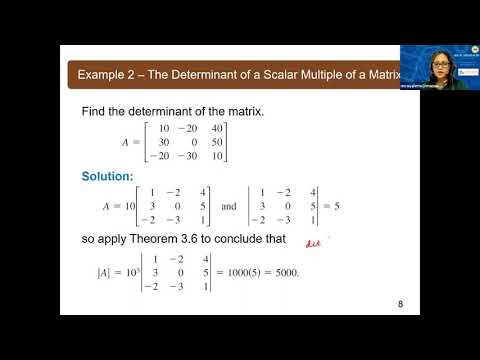 0:08:13
0:08:13
 0:07:53
0:07:53
 0:07:50
0:07:50
 0:10:48
0:10:48
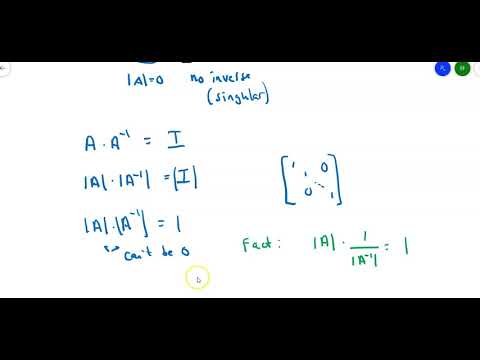 0:14:59
0:14:59
 0:11:36
0:11:36
 0:02:51
0:02:51
 0:02:20
0:02:20
 0:07:55
0:07:55
 0:31:51
0:31:51
 0:15:40
0:15:40
 0:10:31
0:10:31