filmov
tv
Riemann Curvature Tensor - 1
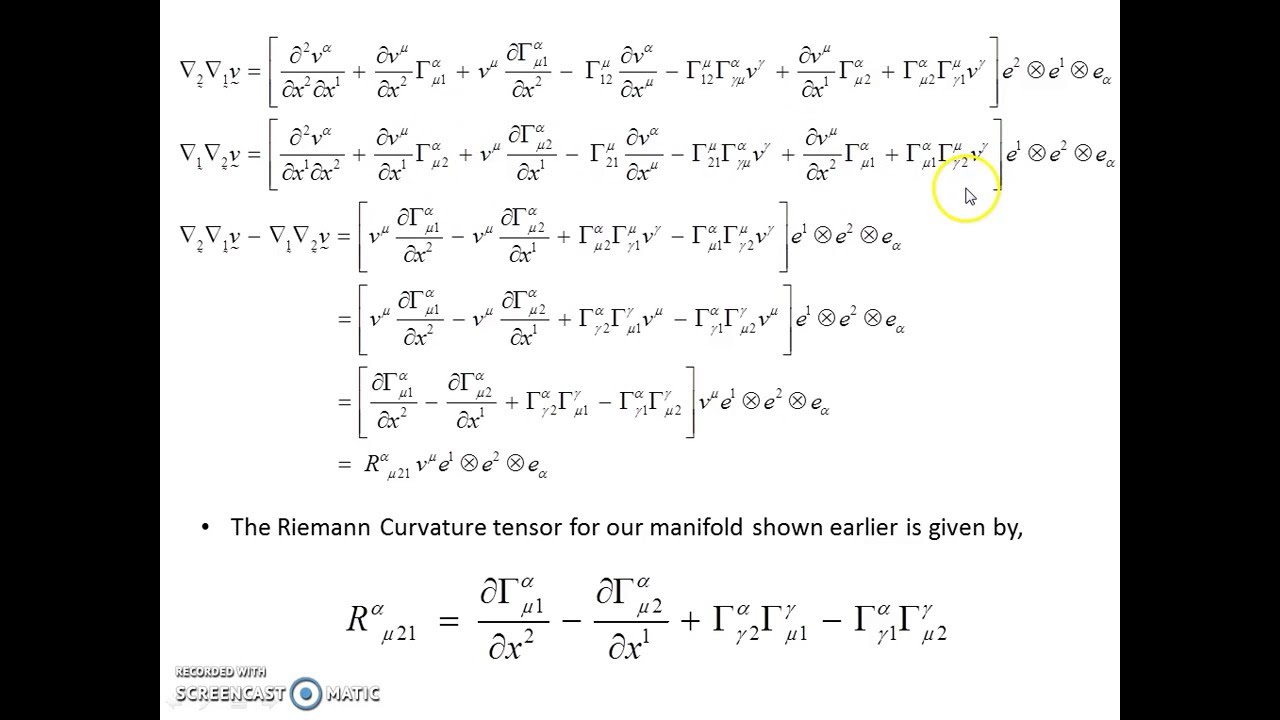
Показать описание
This video looks at one method for deriving the Riemann Curvature tensor using covariant differentiation along different directions on a manifold.
Riemann Curvature Tensor - 1
Relativity 107c: General Relativity Basics - Curvature, Riemann Tensor, Ricci Tensor, Ricci Scalar
Tensor Calculus 22: Riemann Curvature Tensor Geometric Meaning (Holonomy + Geodesic Deviation)
Classroom Aid - Riemannian Curvature Tensor
Riemann & Ricci Tensors & The Curvature Scalar
Classroom Aid - Riemannian Curvature Tensor xy
Lecture 1 | Introduction to Riemannian geometry, curvature and Ricci flow | John W. Morgan
Riemann Curvature Tensor
015- Riemann Curvature Tensor, Nonlinear Equations, Multidimensional Array for Tensor, Christoffel
Tensor Calculus Ep. 15 | Riemann Curvature Tensor
GRSS 055 Riemann curvature tensor part one
Lecture 12: Introduction to Riemann Curvature Tensor.
The Maths of General Relativity (5/8) - Curvature
The Metric Tensor in 20 Glorious Minutes
The Riemann Curvature Tensor and its Derivation.
Lecture 25: Riemannian Curvature
Riemann Christoffel Tensor
17. Riemann Curvature (General Relativity)
The Riemann Curvature Tensor (Lecture #17a of a course on Relativity & Cosmology)
Tensor Calculus 23: Riemann Curvature Tensor Components and Symmetries
riemann curvature tensor
Differential Geometry #1 - Riemann Curvature Tensor
Introduction to the Riemann tensor
Tensor Calculus 24: Ricci Tensor Geometric Meaning (Sectional Curvature)
Комментарии
 0:07:21
0:07:21
 0:34:31
0:34:31
 0:29:13
0:29:13
 0:06:14
0:06:14
 1:08:12
1:08:12
 0:06:14
0:06:14
 0:58:06
0:58:06
 0:09:01
0:09:01
 1:17:29
1:17:29
 0:42:25
0:42:25
 0:09:39
0:09:39
 1:06:28
1:06:28
 0:10:39
0:10:39
 0:19:43
0:19:43
 0:05:58
0:05:58
 1:35:58
1:35:58
 0:00:16
0:00:16
 0:49:41
0:49:41
 0:22:21
0:22:21
 0:25:31
0:25:31
 0:17:18
0:17:18
 0:24:41
0:24:41
 0:26:45
0:26:45
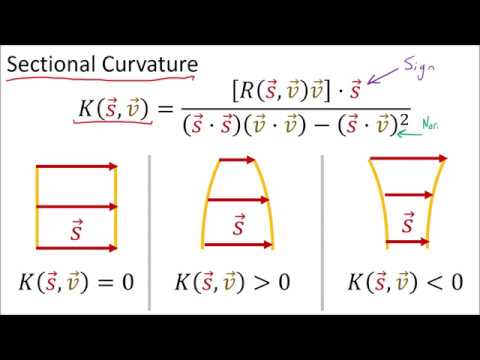 0:28:54
0:28:54