filmov
tv
Relativity 107c: General Relativity Basics - Curvature, Riemann Tensor, Ricci Tensor, Ricci Scalar
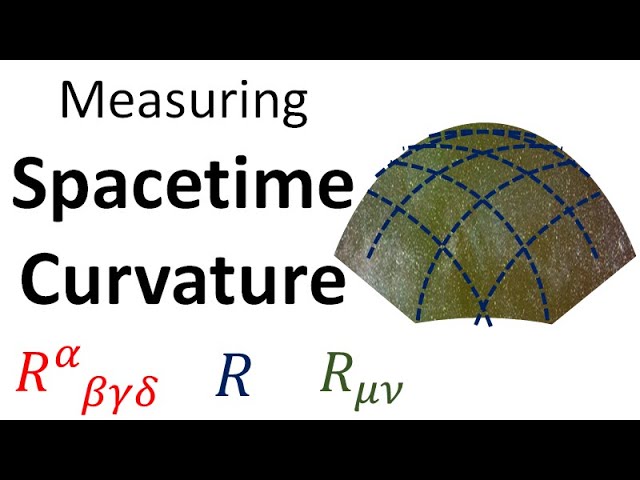
Показать описание
You are free to continue watching to the next video, but if you feel you are getting confused, here are some other videos on curvature here:
0:00 Introduction
1:56 Riemann Curvature Tensor
8:12 Riemann Tensor Components + Symmetries
15:29 Riemann Tensor - Geodesic Deviation
19:04 Ricci Curvature Tensor
23:46 Ricci Curvature Scalar
25:46 Curvature of Rindler Metric
33:19 Summary
Relativity 107c: General Relativity Basics - Curvature, Riemann Tensor, Ricci Tensor, Ricci Scalar
Relativity 107a: General Relativity Basics - Equivalence Principle and Proper Acceleration
Relativity 107f: General Relativity Basics - Einstein Field Equation Derivation (w/ sign convention)
Relativity 107b: General Relativity Basics - Manifolds, Covariant Derivative, Geodesics
Relativity 107e: General Relativity Basics - Stress-Energy-Momentum Tensor
Relativity 107d: General Relativity Basics - Curved Spacetime for Newtonian Gravity (Newton Cartan)
Demystifying The Metric Tensor in General Relativity
Einstein's Universe: Understand Theory of General Relativity
Why The Theory of Relativity Doesn't Add Up (In Einstein's Own Words)
The Meaning of the Metric Tensor
How Einstein derives the Riemann tensor in his paper on General Relativity
Relativity 108a: Schwarzschild Metric - Derivation
The Maths of General Relativity (5/8) - Curvature
1. Gravity is Geometry (General Relativity)
General relativity | General relativity lecture | General relativity explained | Field equations
General Theory of Relativity | General Relativity Lecture | General Theory of Relativity Lecture
What is General Relativity? Lesson 68: The Einstein Tensor
General relativity | General relativity lecture | Einstein field equations | Special relativity
Relativity 102a: Keys to Mathematics of Relativity - Invariance
How Einstein Uncovered the Path a Particle Traces Through Spacetime!
Intro to General Relativity - 13 - Non-inertial frames in Special Relativity and Rindler coordinates
Relativity 105a: Acceleration - Hyperbolic Motion and Rindler Horizon
Relativity 109c: Gravitational Waves - Wave Derivation (The Lorenz Gauge)
General Relativity, Lecture 17: solving Einstein's equations for FLRW
Комментарии
 0:34:31
0:34:31
 0:25:03
0:25:03
 0:36:51
0:36:51
 0:36:21
0:36:21
 0:34:07
0:34:07
 0:25:13
0:25:13
 0:14:29
0:14:29
 1:57:09
1:57:09
 0:17:01
0:17:01
 0:19:22
0:19:22
 0:11:10
0:11:10
 0:30:48
0:30:48
 0:10:39
0:10:39
 0:15:49
0:15:49
 1:20:48
1:20:48
 0:31:25
0:31:25
 0:46:56
0:46:56
 1:02:50
1:02:50
 0:08:53
0:08:53
 0:22:36
0:22:36
 0:49:12
0:49:12
 0:31:37
0:31:37
 0:23:55
0:23:55
 0:20:54
0:20:54