filmov
tv
Tensor Calculus 23: Riemann Curvature Tensor Components and Symmetries
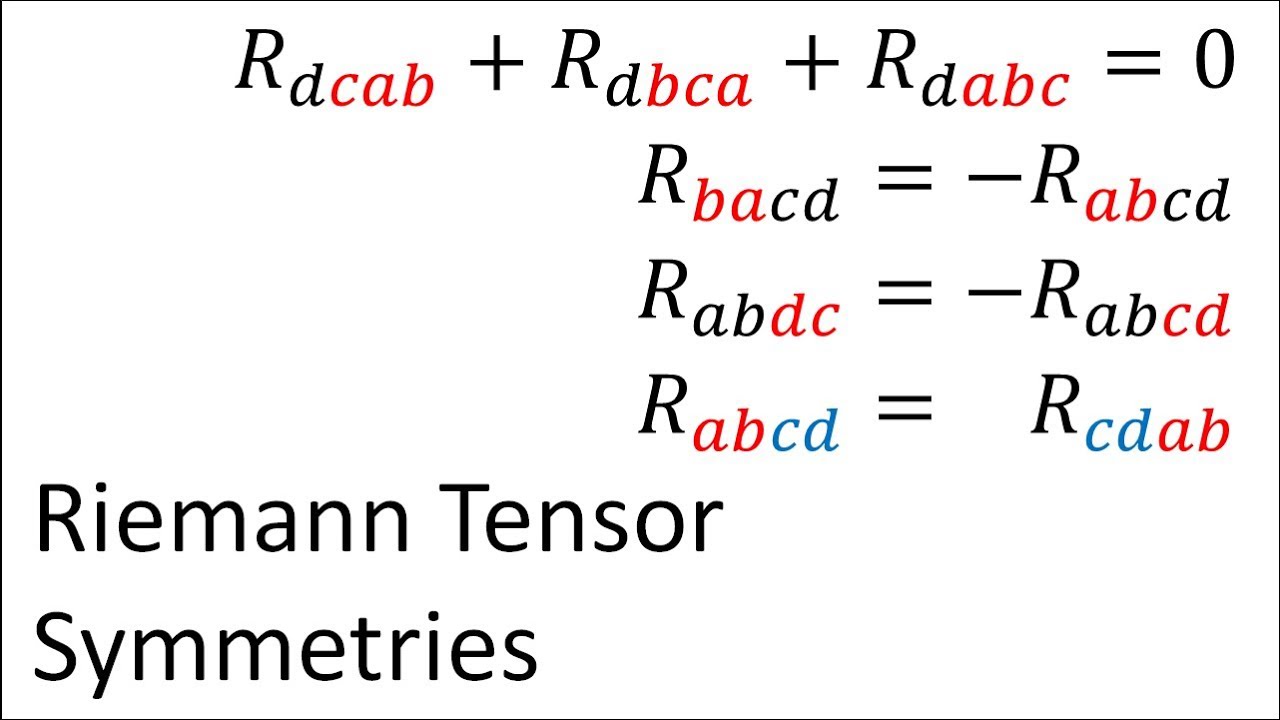
Показать описание
Tensor Calculus 23: Riemann Curvature Tensor Components and Symmetries
Tensor Calculus 22: Riemann Curvature Tensor Geometric Meaning (Holonomy + Geodesic Deviation)
Tensor Calculus 24: Ricci Tensor Geometric Meaning (Sectional Curvature)
Riemann & Ricci Tensors & The Curvature Scalar
Tensor Calculus Ep. 15 | Riemann Curvature Tensor
Riemann Christoffel Tensor
CAT REACTS TO RICCI TENSOR FOR THE FIRST TIME!! | Tensor Calculus 24: Ricci Tensor Geometric Meaning
Classroom Aid - Riemannian Curvature Tensor xy
riemann curvature tensor
Relativity 107c: General Relativity Basics - Curvature, Riemann Tensor, Ricci Tensor, Ricci Scalar
Tensor Calculus 26 - Ricci Tensor/Scalar Properties
Introduction to the Riemann tensor
Understanding Tensor Calculus | Symmetries of the Riemann Tensor
GRSS 123 Ricci tensor
3.An easy Mathematical Derivation of The Riemann Tensor
[Locality] 20. Riemann curvature tensor
Curvature Tensor
Graduate Course: Introduction to the Riemannian Curvature Dimension condition - 9
The Riemann Curvature Tensor (Lecture #17a of a course on Relativity & Cosmology)
The Riemann Curvature Tensor and its Derivation.
Lecture 12: Introduction to Riemann Curvature Tensor.
Riemann Curvature Tensor - 2
Ricci Tensor & Ricci Scalar: Gravity & General Relativity #23 | ZC OCW
Tensor Calculus 25 - Geometric Meaning Ricci Tensor/Scalar (Volume Form)
Комментарии
 0:25:31
0:25:31
 0:29:13
0:29:13
 0:28:54
0:28:54
 1:08:12
1:08:12
 0:42:25
0:42:25
 0:00:16
0:00:16
 0:06:13
0:06:13
 0:06:14
0:06:14
 0:17:18
0:17:18
 0:34:31
0:34:31
 0:28:59
0:28:59
 0:26:45
0:26:45
 0:23:39
0:23:39
 0:08:09
0:08:09
 0:14:54
0:14:54
![[Locality] 20. Riemann](https://i.ytimg.com/vi/0dIkbTQGHGo/hqdefault.jpg) 0:56:19
0:56:19
 0:33:24
0:33:24
 1:33:14
1:33:14
 0:22:21
0:22:21
 0:05:58
0:05:58
 1:06:28
1:06:28
 0:11:29
0:11:29
 1:09:54
1:09:54
 0:32:12
0:32:12