filmov
tv
Tensor Calculus 24: Ricci Tensor Geometric Meaning (Sectional Curvature)
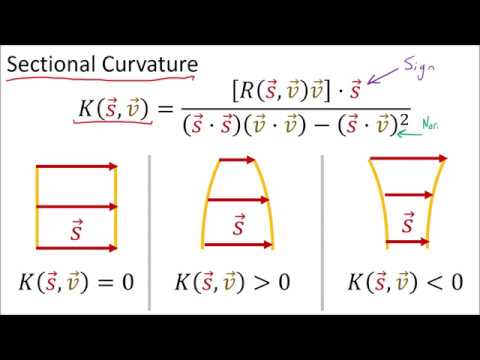
Показать описание
Previous videos on Riemann Curvature Tensor:
Tensor Calculus 24: Ricci Tensor Geometric Meaning (Sectional Curvature)
CAT REACTS TO RICCI TENSOR FOR THE FIRST TIME!! | Tensor Calculus 24: Ricci Tensor Geometric Meaning
Tensor Calculus 25 - Geometric Meaning Ricci Tensor/Scalar (Volume Form)
Tensor Calculus 26 - Ricci Tensor/Scalar Properties
Ricci Tensor
Ricci's Tensor
Ricci Tensor and Scalar
Riemann & Ricci Tensors & The Curvature Scalar
Ricci Tensor Derivation
Einstein space and Ricci tensor
Ricci tensor, ricci scaler, Einstein tensor
Tensor Calculus 9: Integration with Differential Forms
Tensor Analysis, Lec-24 (Ricci Tensor), by Dr.D.N.Garain, For B.Sc/M.Sc Maths & Physics
Classroom Aid - Riemannian Curvature Tensor
Independent components of the Riemann tensor
GRSS 123 Ricci tensor
Classroom Aid - Riemannian Curvature Tensor xy
Ricci tensor for sphere
Relativity 107c: General Relativity Basics - Curvature, Riemann Tensor, Ricci Tensor, Ricci Scalar
Tensor Calculus Ep. 15 | Riemann Curvature Tensor
Tensor Calculus 22: Riemann Curvature Tensor Geometric Meaning (Holonomy + Geodesic Deviation)
Weyl Tensor
An application of the Riemann tensor - corrected
Riemann Curvature Tensor - 2 (corrected)
Комментарии
 0:06:13
0:06:13
 0:32:12
0:32:12
 0:28:59
0:28:59
 0:08:17
0:08:17
 0:17:09
0:17:09
 0:11:48
0:11:48
 1:08:12
1:08:12
 0:23:22
0:23:22
 0:00:16
0:00:16
 0:01:01
0:01:01
 0:18:34
0:18:34
 0:15:19
0:15:19
 0:06:14
0:06:14
 0:15:50
0:15:50
 0:08:09
0:08:09
 0:06:14
0:06:14
 0:13:01
0:13:01
 0:34:31
0:34:31
 0:42:25
0:42:25
 0:29:13
0:29:13
 0:05:10
0:05:10
 0:26:51
0:26:51
 0:13:41
0:13:41