Deriving the Dirac Equation

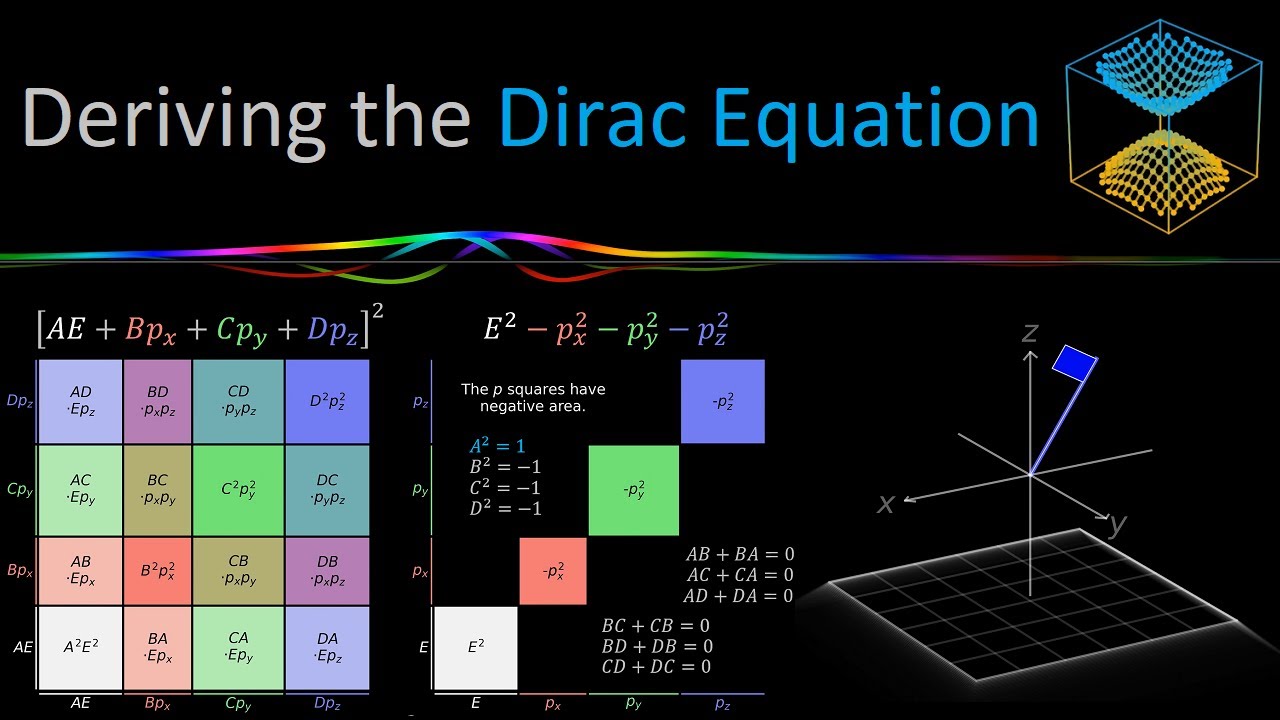
In this video, we'll derive the Dirac equation, and see where it comes from! :)

Recommended reading: Introduction to Elementary Particles, by David Griffiths, Chapter 7.

Equations from the videos are available as downloadable PDFs on my Patreon. I'll also be on there to answer any questions you might have.

Chapters:

0:00 Intro
0:38 Three Principles for the Dirac Equation
3:12 Square Root of the Mass Shell
7:30 Anticommutation Relations
9:50 The Dirac Matrices
10:58 The Dirac Equation
13:58 Spinors

#physics #quantum #relativity

[RichBehiel]

Hey all, thanks for checking out this video! :)

While reviewing the video just now, I realized I probably should have been more clear about covariance and contravariance. This affects the sign of the momentum terms (more generally, the space-like terms), and is a perennial source of dropped minus signs 😅 The plus signs at 13:20 are due to using the contravariant Dirac matrices, three of which which absorb the minus signs, see the Wikipedia article “gamma matrices” for more info.

Anyway, the main idea I hope you’ll take away from this video is that the anticommutation relations for the Dirac matrices arise from taking the square root of the mass shell.

[SirTravelMuffin]

The "I'm letting the squares breathe..." was genius for geometrically visualizing variables!

[JakeFace]

A thousand jargon-filled wikipedia articles could not have given me the clarity I now posess thanks to this video. Thanks so much

[jim]

Dirac was known for both the brevity and precision of his speech. At a conference he was lecturing and writing at a blackboard. A member of the audience said at the conclusion of Dirac's presentation, "I don't understand your equation in the upper right." Dirac said nothing and returned to his seat at the table with other lecturers. After an uncomfortable silence, the conference moderator asked, "Mr. Dirac, are you going to answer the man's question?" Dirac: "It wasn't a question. It was a statement."

[bastiaanvanhoorn]

The teacher I had for QFT was one of the best teachers i ever met, and yet, this is so much more insightful then what he ever managed on a blackboard. The visualisation is genius.

[eg]

Straight-up the best physics videos on youtube

[peterhall]

Fun fact. Back in 1972 I was taught some mathematical physics by the late Joe Moyal. Joe was invited by Dirac to Cambridge during the World War 2 to discuss Joe's work on statistical foundatiions of quantim mechanics (there is a 1947 paper with Bartlett and Kendall which also deals with the issues). Joe met Dirac and I can say that I have shaken hands with someone who has shaken hands with Dirac.

[FunkyDexter]

The reason the coefficients don't commute (so the opposite breathing squares cancel out) and why the coefficient squared are negative numbers, is because a spinor is a unit quaternion! In fact, the nature of spin is a bit less mysterious if instead of using Dirac matrices we use quaternions, for rotations on a 4D hypersurface :)

[Sol-En]

Wow this lesson is much better than in Oxford or Harvard. Very interesting to see visualisation of solution of Dirac equation to compare with Klein-Gordon and Schrodinger equations. Also very interesting to see how do the wave functions in the bispinor responsible for spin up, spin down, and antimatter affect each other

[pelegsap]

Absolutely terrific :)

btw, here's a tip for everyone who sees this: geometric (Clifford) algebra really helps understanding these spinors better (and also space-time in general).

[dialgapalkia]

This sort of accessible education will prove to be revolutionary.

[natecoad]

Wow. I have devoted all of my time to getting into GR and have only had a basic rundown of QM but have wanted to get into the more interesting QM stuff for a while. You did a great job and I love hearing your excitement for introducing new ideas. That was perfect and felt so clean. Great stuff!!!!

[evilotis]

eigenchris's "Spinors for Beginners" series is a really good introduction to, well, spinors for beginners!

[Phantores]

I never heard of this way of deriving the equation and now the matrices seem so much more understandable... Congrats dude, you just did the impossible of explaining all of this stuff I tried to understand for months

[mahapeyuw]

I was watching Alexander Fufaev video on the Schrodinger equation (He gave an excellent explanation), and he mentioned Diracs equation as the next thing for relativistic SE.

I'm happy to have found this. It's been years since I finished.
So here I am learning new things and brushing up the old, to enable drawing out something new if I persist just enough.

This is excellent and widened my understanding but raised further questions too.

[TheSandkastenverbot]

You really have a gift for explaining abstract topics!

[thabomsiza]

I know the Dirac equation but never really saw it being derived before. Either I was incredibly blind in my education or my educators hand waved everything and I struggled from there. These videos are opening my eyes more than my entire journey through undergrad and honors.

[ThomasGutierrez]

Brilliant content! Looking forward to the next installment on spinors!

[renscience]

This is fantastic. A secret closely guarded by academics has been simplified so that even I can understand it with only a BS in Engineering. Thank you
I need to watch more on spin as it too is a kept secret usually presented and discussed in baloney classical ways. Just a glimpse here helped already.
I would love for someone to simplify Hilbert space as it too is slung around a lot without explanation.

[michaeljin]

Thank you so much! I was reading the book “The strangest man” by Graham Farmelo, and your video gives an in depth looking into Dirac equation. This let me give the following parallel from a basic math concept to understand Dirac equation.

While Cartesian coordinates, introduced by René Descartes, provide a structured way to map points in space using two or more axes, the Dirac matrices, part of the Dirac equation in quantum mechanics, offer a profound mathematical framework for describing the behavior of particles in spacetime.

Just as Cartesian coordinates allow us to pinpoint locations in space with precision, the Dirac matrices enable us to describe fundamental properties of particles, such as spin and angular momentum, within the framework of quantum mechanics. Both systems provide a means to understand and navigate complex phenomena: Cartesian coordinates in the realm of classical mechanics and geometry, and Dirac matrices in the intricate domain of quantum mechanics.

In essence, while Descartes paved the way for understanding space in terms of ordered pairs of numbers, Dirac expanded this notion to encompass the intricate fabric of spacetime at the quantum level, offering a beautifully intertwined perspective on the mathematical underpinnings of the universe.