Binomial Probability Distribution for tossing coin by Sir Tanveer

A binomial distribution can be thought of as simply the probability of a SUCCESS or FAILURE outcome in an experiment or survey that is repeated multiple times. The binomial is a type of distribution that has two possible outcomes (the prefix “bi” means two, or twice).
For example, a coin toss has only two possible outcomes: heads or tails and taking a test could have two possible outcomes: pass or fail.
Binomial distributions must also meet the following three criteria:

The number of observations or trials is fixed. In other words, you can only figure out the probability of something happening if you do it a certain number of times. This is common sense—if you toss a coin once, your probability of getting a tails is 50%. If you toss a coin a 20 times, your probability of getting a tails is very, very close to 100%.
Each observation or trial is independent. In other words, none of your trials have an effect on the probability of the next trial.
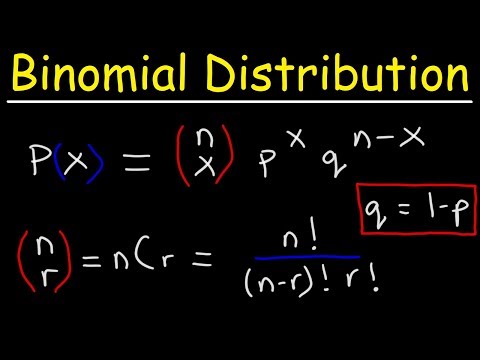
The binomial distribution formula is:

b(x; n, P) = nCx * Px * (1 – P)^n – x
OR
b(x; n, P) = nCx * Px * (q)^n – x
Where:
b = binomial probability
x = total number of “successes” (pass or fail, heads or tails etc.)
P = probability of a success on an individual trial
n = number of trials

The probability of success (tails, heads, fail or pass) is exactly the same from one trial to another.
Step 1: Identify ‘n’ from the given question. Using our sample question, n (the number of randomly selected items) is 5.

Step 2: Identify ‘X’ from the problem. X (the number you are asked to find the probability for) is:-
i) Exactly 3 heads
ii) At least 3 heads
iii)At the most 2 heads .

Step 4: Find p and q. p is the probability of success and q is the probability of failure. Here, p = 0.5. and failure q is also 0.5. Because, if there is no p and q is given in the question, then the p and q automatically becomes 1/2.
Remember:- p+q=1

Subscribe, Share the channel.

Thanking You...!

[MuhammadSajid-pryr]

Acha explain kia Hai sir.
Concept clear hogya

[alinasir]

Sir, kafi useful or understandable video hai ye.
Thanks sir...!

[muhammadsabir]

Really informative sir, keep it up sir.

[abubakarkhan]

Sir, it is very useful to me but, make video on hypergeometric distribution also.
Thank you sir.

[ajayguptaajaygupta]

your explaination is good, easily anyone understood .

[zainul-ab]

Thanks sir ! Thanks a lot! It really helped me

[technicaltaha]

Sir kafi achi'video hae or sir apnea 1/2 coins kae liyae lagaya hae na yea pher kahi per p ki values nahi hogi tou hum 1/2 hi lagaye gae plz sir batae

[sidraqayyum]

thanks sir,
i have a query here, why you do not perform p-q step here?

[khushdeepbhatti]

Sir binomial mean, median, mode, step deviation v smja dao eh wala ta smj a gea 😊

[davidjason]

Sir how to understand the question that we should solve this question by binomial probability distribution or some other method? Is there any hint for that to confirm the question yes I have to use binomial formula ?

[daniyalhaleemvlogs]

Sir what if the limit is infinite, how will we then do atleast 3 heads?

[naziatahir]

Sir please make a video on Random variable please

[ajilashram]

I don't understand the p and q value. It is constant? Or not how will find this
I don't understand hindi plz tell anyone in English

[lcproduction]

Definition of binomial theorem and probability