filmov
tv
Factoring Quadratics WITHOUT Guessing Product & Sum

Показать описание
0:00 x^2+32x+92
7:00 x^2-6x-91
10:10 x^2+9x+20
12:53 2x^2-7x-4
15:42 x^2+8x+4
17:50 x^2+4x+10
Factoring Quadratics WITHOUT Guessing Product & Sum
Factor ANY Quadratic Equation Without Guessing | Outlier.org
Solving Without Factoring or Quadratic Formula
FASTEST way to factor a trinomial! #shorts
NO GUESSWORK Trick to Factor Quadratics!
How to Quickly Factor without Guess and Check
Learn the AC METHOD in 2 MINUTES | Factoring Trinomials | Algebra 2
Quadratic Equations | Solve by factoring | Free Math Videos
Factoring Trinomials
How to Factor Trinomials without Guessing any Factors
The Quadratic Method: Factoring without Guessing and Testing
A Different Way to Solve Quadratic Equations
Factoring trinomials in the form ax^2 + bx + c without guessing and checking
Factoring Trinomials: Direct method with no “guess and check”
Solving Quadratic Equations by Factoring #mathteachergon #quadraticequation #factoring #grade9math
How to factor quadratics quickly without guess and check or mental math
Factoring Quadratics... How? (NancyPi)
Factoring Trinomials Guess and Check
Factoring No Guessing
How to Factor and Solve Quadratics - Sneaky Trick - No Fuss Factoring
Box Method of Factoring Trinomials (In Under 2 Minutes)
Learn How to Solve a Quadratic Equation by Factoring by 'guessing'
Factoring out a variable and using zero product property to solve
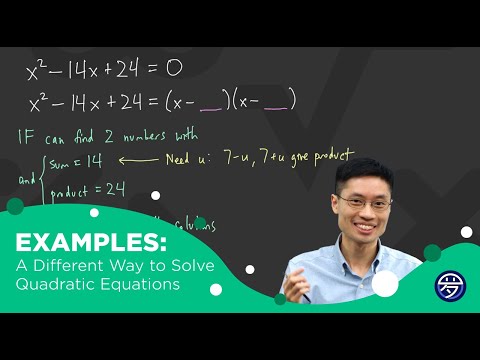
Examples: A Different Way to Solve Quadratic Equations
Комментарии
 0:20:01
0:20:01
 0:14:02
0:14:02
 0:00:58
0:00:58
 0:00:55
0:00:55
 0:00:58
0:00:58
 0:02:51
0:02:51
 0:02:45
0:02:45
 0:02:43
0:02:43
 0:04:17
0:04:17
 0:07:51
0:07:51
 0:11:13
0:11:13
 0:03:49
0:03:49
 0:09:49
0:09:49
 0:09:29
0:09:29
 0:01:00
0:01:00
 0:02:06
0:02:06
 0:09:14
0:09:14
 0:03:40
0:03:40
 0:02:32
0:02:32
 0:05:42
0:05:42
 0:01:36
0:01:36
 0:01:50
0:01:50
 0:03:19
0:03:19
 0:40:05
0:40:05