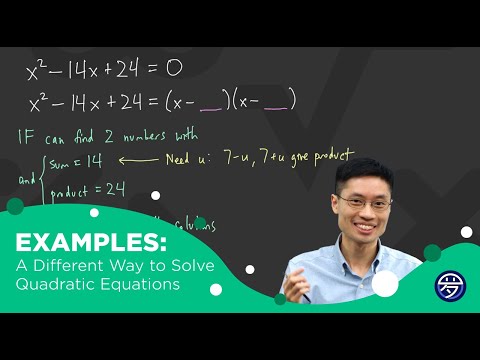
Examples: A Different Way to Solve Quadratic Equations

Want to understand how to solve any quadratic equation, without just memorizing the quadratic formula? In this lesson full of worked examples, Daily Challenge founder, Carnegie Mellon University professor, and U.S. International Math Olympiad coach Po-Shen Loh introduces a simple, different method for solving quadratic equations. Instead of guess-and-check, this method uses students’ existing experience searching for a pair of numbers with a given sum and product to solve quadratics.

Thanks to the Templeton World Charity Foundation for their support of this work.

==========
UNIQUE APPLICATIONS OF THE METHOD
Looking for a specific kind of problem that this method can solve? Check out the timestamps below:

21:24 Coefficients with different signs
25:03 If the coefficient of x is odd
27:28 If x² has a coefficient other than 1
30:41 Deriving a Quadratic Formula
33:14 Deriving the commonly learned Quadratic Formula

HISTORICAL PARTS OF THE SOLUTION
38:18 Viète’s Relations
39:00 Babylonian Mathematics

==========
ABOUT DAILY CHALLENGE

ABOUT EXPII
Expii is a free math and science education platform that embraces what makes us all individuals: our voices, experiences, and cultures. We encourage choice and experimentation by giving students the power to choose how they learn. Through creative, fun, practical, and personal lessons each person can have their own aha moment.

JOIN OUR MAILING LIST

[BravoTango-vtem]

This is the type of math teacher I like. The teacher who actually lets their kids understand the math instead of making them pass the exam so he doesn’t have to worry about it again.

[robertmcfarlane]

This is one of the best lectures on quadratic equations I have seen. Thank you Mr Loh for clear and understandable information presented in a concise manner.

[jimspelman]

I am a math and computer science major and a math teacher (middle school) and, like others have commented, I have never seen this method before. I love how you can easily get non-integer solution sets! Perhaps instead of the "Po-Shen Method" I shall henceforth call this the "Magic Quadratic Po-Shen" (just to add a little wordplay)!

[rajeevmaakan]

I salute you man! This is absolutely amazing....have been teaching math for 35 years, you have just made my job easier for whatever remaining number of years I will be able to teach. That's why they say....it doesn't matter you learned something new so late in life, what matters is you got new knowledge (new, I say, for the learner), new wisdom. And last but not the least, what is the primary objective of Problem solving....in any branch of knowledge or education....it is to achieve the objective in as simple and as uncomplicated a manner as possible....so that it can bring a smile on the faces of our students. Thanks a zillion bro!!!

[RavinaTutorial]

Sir please keep posting such methods so that people start analyzing mathematics and taste the true essence of it, Thank you so much for sharing this🙏.

[beteilniguse]

This is exactly how we learned in my country East Africa. God how did I forget this, and our professor has zero clue and got me confused and spent days to really figure this out. I wish I found you early. I would have saved a lot of time. But thank you so much! You did an amazing job. keep them coming.

[norbertotorbeso]

I have been teaching this method to my high school students back in 1999 and yes, this is another way. Students will definitely learn if a teacher will expose them to different ways of solving the problem. Nice presentation...

[harisomerbasic]

My teacher had me explain this to her and now she's recommending your technique and referencing to you! Thanks from Sweden!

[tamajongmichaelnkeh]

That is the easiest method I have ever used. I can't imagine that I did not know this before. Thank you sir

[espenvang]

What a marvellous lecture in every way! Not just a genius new method for solving quadratic equations, but also clear and precisely presented.

[blockyboxhead]

I watched this entire video without getting bored or wanting to click off. This proves that this video is godly

[g.v.]

I’m reviewing all of my precalculus math that I had 50 years (!) ago. I wish I’d had you for a math teacher back then. Thank you so much for the teaching an old dog a new trick! I’m planning to learn a lot more new tricks. I’ll be watching more of your videos in the future! Thanks so much!!

[gautamghaisas]

I wish we could have learnt all subjects this way in school. So systematically and brilliantly explained

[johankotze]

Dear Prof Loh, I am now retired at 61, and I have worked in IT for most of my working life. I have, however, always dabbled in mathematics. The thrill of discovering this only recently, is one the reasons for my continued love of the subject. If you have not done so, adding the graphical interpretation would help many other students. Thank you very much.

[geraldillo]

Every highschool teacher should learn this method

[dananewborn]

Thank you from my 19 person math class who enjoyed learning this method

[philplante]

The method at 00:46 is just the quadratic formula. - B/2A +- u, where u^2 = B^2/4 - AC. The x-coordinate of the vertex of a parabola is -B/2A. It is also the line of symmetry. If you extend the line of symmetry through the x-axis, you will see that one root is a distance SQRT(B^2 - 4AC) to the right and the other is a distance -SQRT(B^2 - 4AC) to the left. The method is a bit different than just plugging numbers into the quadratic formula, but there is really no new math here, just a different way to apply the quadratic formula.

[sandanadurair]

You are simply great.
I have never seen such a pleasant mathematics teacher during my learning period. I am now 72. Today you taught me a novel technique. I salute you

[keinKlarname]

You're such a friendly person! It's a pleasure to follow your explanations, Mr. Loh.

[wreckim]

As a 4th grade teacher, I can tell you that it would be incredible to have this kind of talent exposed to the kids right from 1st grade on. So much of the math problems we have is because we lack our natural curiosity and stop having fun. Math gets to be all about 'testing' pretty quick once they leave 6th grade. Thanks for posting, great lesson.