filmov
tv
Geometric Algebra in 2D - Fundamentals and Another Look at Complex Numbers

Показать описание
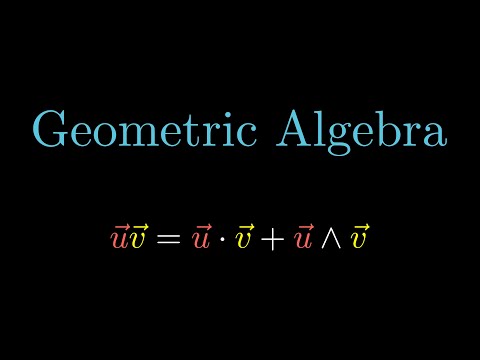
In this video, I introduce some of the concepts of geometric (Clifford) algebra, focusing on two-dimensional space (R^2). We'll talk about the wedge (exterior) product, review the dot product, and introduce the geometric product. We'll see that a new mathematical object, the bivector, arises from considering the wedge product. Furthermore, we're going to see that this bivector behaves like an imaginary unit in that it squares to -1. Since it has this property, it naturally relates to vector rotation in 2D, like the complex numbers.
Recommended video on Clifford algebra:
Recommended video on Clifford algebra:
A Swift Introduction to Geometric Algebra
2D Spacetime from Nilpotents | Intro to Geometric Algebra
Quaternions Are Not Four-Dimensional Objects
2D Rotations in Geometric Algebra
Amazing Things You Can Do in Geometric Algebra - Explained
Constructing Geometric Algebra
Addendum to A Swift Introduction to Geometric Algebra
Geometric Algebra vs. Clifford Algebra
Mathematics Grade 12 Number Patterns , Sequences and Series Exam Term 1 Revision
Geometric Algebra in 2D - Fundamentals and Another Look at Complex Numbers
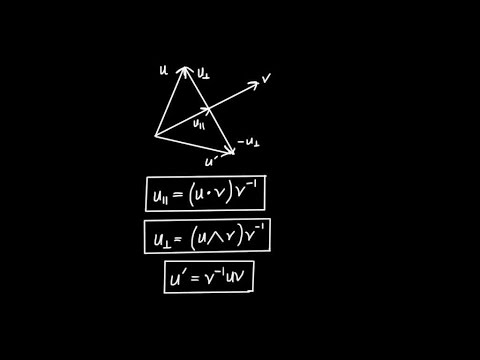
Geometric Algebra in 2D - Vector Projection and Reflection
Let's remove Quaternions from every 3D Engine: Intro to Rotors from Geometric Algebra
Algebro geometric context of Geometric Algebra in 2D symbol
A Swift Introduction to Spacetime Algebra
Geometric Algebra in 2D - Some Trigonometry
The fascinating absurdity of Geometric Algebra
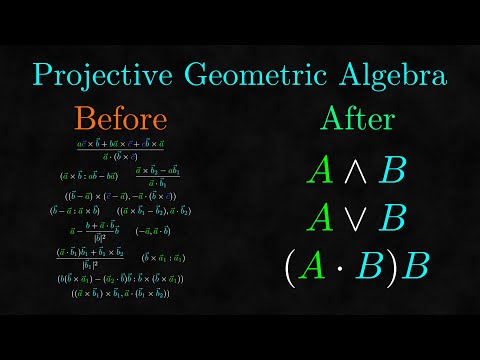
A Swift Introduction to Projective Geometric Algebra
Finding the Area of Arbitrary Polygons With Geometric Algebra
PGA Ep 6 : A new Hope II
Geometric Algebra in 2D - Linear Algebra and Cramer's Rule
Solution of two line intersection using geometric algebra.
An Overview of the Operations in Geometric Algebra
GAME23 David Eelbode - Rotors and Spinors
From Zero to Geo Introduction (Geometric Algebra Series)
Комментарии
 0:44:23
0:44:23
 0:04:39
0:04:39
 0:00:41
0:00:41
 0:00:49
0:00:49
 0:09:34
0:09:34
 0:00:40
0:00:40
 0:23:40
0:23:40
 0:00:54
0:00:54
 0:25:59
0:25:59
 0:35:14
0:35:14
 0:31:23
0:31:23
 0:16:48
0:16:48
 0:04:41
0:04:41
 0:38:59
0:38:59
 0:14:47
0:14:47
 0:01:00
0:01:00
 0:54:38
0:54:38
 0:00:52
0:00:52
 0:12:34
0:12:34
 0:30:00
0:30:00
 0:07:22
0:07:22
 0:40:35
0:40:35
 0:56:55
0:56:55
 0:11:11
0:11:11