A Swift Introduction to Spacetime Algebra

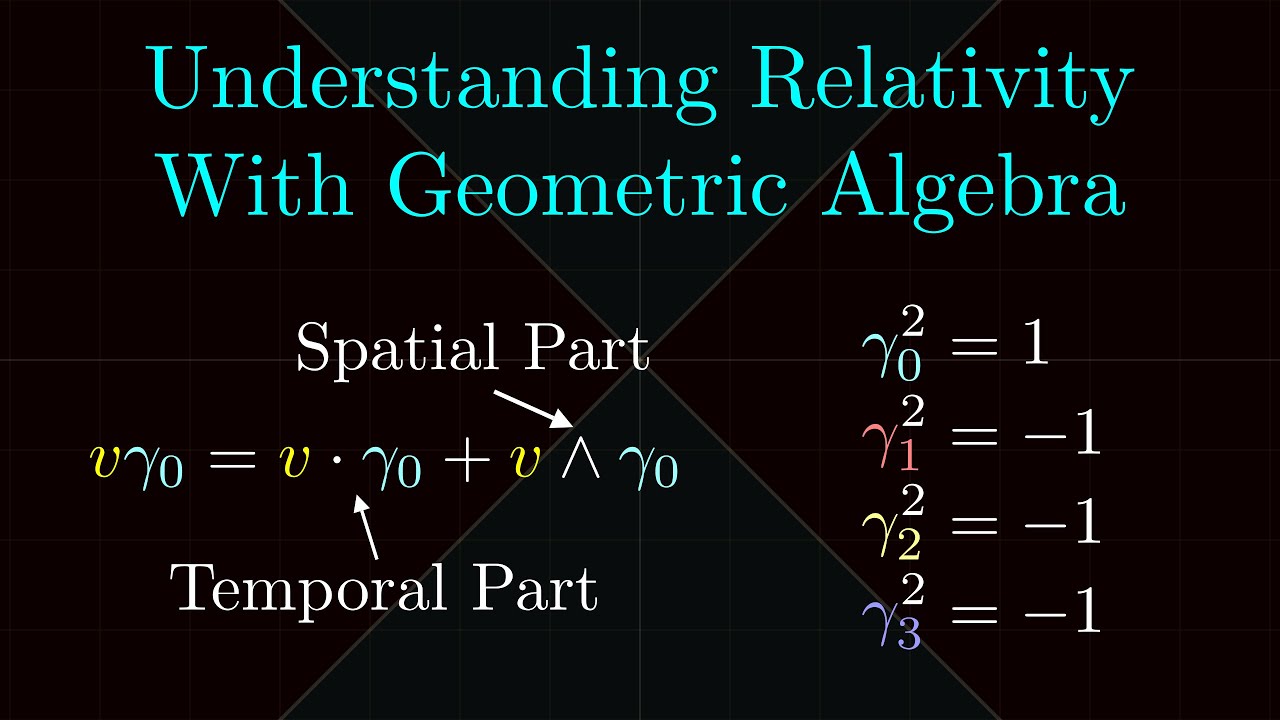
This video is a fast-paced introduction to Spacetime Algebra (STA), which is the geometric algebra of Minkowski space. In it, we figure out what the problems are with the way introductory textbooks usually describe special relativity and how we can solve those problems by using spacetime and redefining length in terms of the spacetime interval. This creates Spacetime Algebra. We then study STA and learn about spacetime splits and Lorentz transformations.

This video is my submission for SoME2, and just in time too! It was probably the hardest video to make that I've ever done because of the length of the video and the difficulty of the material being presented. In the end, I hope it can be of use to you.

When it comes to resources for applications of STA, you can't go wrong with Doran and Lasenby's Geometric Algebra for Physicists. It includes chapters on every topic discussed here. Here's a few other resources:
Relativistic Mechanics: New Foundations for Classical Mechanics by Hestenes, although he actually uses VGA instead of STA
Electrodynamics: Understanding Geometric Algebra for Electromagnetic Theory (starts out in VGA but switches to STA later on)

Patreon Supporters:
AxisAngles
David Johnston
p11
Richard Penner
Rosario

Sections:
00:00 Introduction
00:54 Prerequisites
02:05 Outline
03:18 Symmetry
05:36 Lorentz Boosts
07:03 Problems With Lorentz Boosts
08:02 Lorentz Boosts Mix Space and Time
08:34 Making Time a Vector
10:08 Visualizing Spacetime
12:27 Lorentz Boosts Change Lengths
12:54 Length vs. Square
13:33 Finding an Invariant Square
14:11 Spacetime Vectors as Reference Frames
14:59 Measuring Length in a Vector's Reference Frame
15:29 Derivation of the Spacetime Interval
17:38 Examples of the Square of a Vector
19:37 Negative Length?
20:26 Spacetime Algebra
22:22 Correspondence Between Space and Spacetime
23:38 Converting Between Spacetime and Space
24:51 Spacetime Splits
25:57 Algebraic View of Spacetime Splits
29:00 Return to Lorentz Boosts
29:51 2D Lorentz Boosts
32:17 Lorentz Boosts = Rotations
33:52 Higher-Dimensional Lorentz Boosts
35:06 Lorentz Transformations
35:40 Various Applications

[JackDespero]

As a physicist: Mind=blown.
I am so used to the other set of mathematical tools that it is hard for me to do anything but simple problems with geometric algebra, but I can appreciate how amazing and nice of a tool it is.

[sudgylacmoe]

To my viewers that are wanting more videos in From Zero to Geo: Good news! I'll be getting back to it right away. I just wanted to get this video out there that I think is a better SoME2 submission than the videos in From Zero to Geo.

EDIT: Since people keep asking me and I realize I should have said it in the video, I said "the zitterbewegung interpretation of quantum mechanics" near the end.

[justynpryce]

I feel bad, I had never heard of David Hestenes until watching this video, and after looking him up I found out he works in the physics department of my university! I'll have to pay him a visit it seems.

Edit: It seems he retired before I started but this was not conveyed on the university's website :/

[MaxxTosh]

1. I want you to know that your intro to geo algebra was the greatest math video I’ve ever seen and I was so excited to see this
2. What was the name of the interpretation of QM, and where can I learn more?
3. I would LOVE similar videos for PGA and CGA!
4. No seriously, thank you so dearly much for the effort you put into this niche but stunning topic

[FoxxAngel_]

I took notes during my second watch and omg, I didn't realised how much information there was. Everything just came up so naturally that I just took it in at first. I hope you continue to do videos on more complex notions in GA, It's so engaging !

[diribigal]

This really helps me see how "hyperbolic rotations" are just like the notations I know, and why there's good intuition in doing geometric algebra stuff with the Minkowski basis/quadratic form. Thanks for such clear explanations!

[vyordanov]

I would like to quote David Hestenes: "I have been pursuing the theme of this talk for 25 years, but the road has been a lonely one where I have not met anyone travelling very far in the same direction.'" Looks like his theory is becoming more popular. Thanks for this video it is fantastic! May be some more details how we can do more calculas in STA. Just one note: Your style is very similar to 3Blue1Brown as a talk. I would recommend to select your own style of presentation.

[stephanevernede]

The fact that time-space split can be modeled by a geometric product blew my mind. Geometric algebra will definitely become the mainstream tool to do and teach physics in a close future. keep the good work your videos are an asset for humanity !

[might_e]

hell yeah 5hr video outlining Hestenes' Zitterbewegung structure in electrons and photons when?

seriously through, i love your work in making these concepts surrounding both relativity and STA easy to follow and visually intuitive whenever possible. keep up the good work :)

[michalbotor]

this is mind boggling.
geometric algebra is the most exciting branch of mathematics i have ever encountered.

[nice]

Its so cool how simple some of these equations can get when viewed in the right lens of relativity!

[pomtubes]

Actually underrated... ur zero to geo video textbook series are the works of a good samaritan... keep up the good work!

[FoughtAgaisntSisera]

This chapter on GA4P has always left me quite stumped. so stoked for this video

[liammccreary]

I’ve been working on this topic for one year now and I can’t get over how beautifully simple the algebra is. It should be standard in physics.

[adamdarx]

A lot of THANKS for your wonderful introduction video. Recently I've been coming across a quantum field theory textbook written by Maggiore ("A Modern Introduction to Quantum Field Theory"). In this book, the author introduces some kind of decomposition of Lorentz group generator J^{\mu\nu} and make into two part consisting of "inner-product"-like things and "outer-product"-like things(Levi-Civita symbol). Before I learn about geometric algebra from your video, I believe I'm still in the lack of insights about Lorentz group. However, once after seeing that you decompose the "geometric product" into two parts exactly similar with what Maggiore's done, I finally notice that the decomposition of geometric product is 100% connected with the one of Lorentz group. In that sense, we know spinor actually transform under Lorentz group, too. That explains why we can find that Spacetime algebra can be viewed as Dirac γ-algebra.

[izaret]

Excellent work. Love how you connected VGA to STA. Very clear as always. I know you plan to push on from zero to geo but I must say I found this type of video even more important. GA is so powerful that it takes a lot of time to explore it and the books from Hestenes or Lasenby are quite dense (for good reasons) which makes it hard (time consuming) for people to go through it. With a video like this it is now much easier for me to have people watch it and then have a discussion about the powers of GA. I could see three additional videos on the 3 connections you mention in the end: mechanics, electrodynamics and quantum mechanics. One main issue I found in talking to people about GA is it takes very long to get to powerful applications and many see the elegance but ask: what do I do with this that I can’t do already? There are videos about the details of GA already, they could be improved with Manim but they exist. The three above don’t (to my knowledge). Well done. Congrats.

[TheMindfulCraftsman]

Thanks for the clear and very welcome explanations! In order to propagate the fundamentals and applications of Geometric Algebra, such more advanced videos are desperately needed. Great work and looking forward to more videos on this topic!

[Bolpat]

This is some god-tier math channel. it does not have this appearance because the audio is behind others, but really good microphones are expensive and the audio is good enough.

[rustybolts]

For me, watching your last two videos has liberated much of the known laws of physics from the rubbish bin. Much I have yet to learn and relearn. Thank you so much. I will be back.

[Mr.Nichan]

I just realized that special relativistic spacetime is quite quaternionic. (Also, I think it's funny that the best thing my spell-checker can guess I meant by "quaternionic" is "fraternization".)