filmov
tv
Linear Algebra 1.2.2 Solution Sets and Free Variables

Показать описание
Linear Algebra 1.2.2 Solution Sets and Free Variables
[Linear Algebra] Solution Sets for Systems of Equations
One Solution, No Solution, or Infinitely Many Solutions - Consistent & Inconsistent Systems
Linear Algebra - 27 - Algebraic Systems of Equations with Matrices
Solution Set - Visualizing Algebra
Linear Algebra Ch 1.5 Solution Sets of Linear Systems # 2
Solving Systems of Linear Equations By Graphing │Algebra
What is a 'Solution Set' for an Equation (Algebra)
Linear Algebra: induction review, solution sets and the rref, CCP, 8-21-24
Applied Linear: complex example, structure of solution sets, 1-24-17, part 2
Gaussian Elimination & Row Echelon Form
Solving Systems of Equations By Graphing
How to Solve Linear Equations With Variables on Both Sides : Linear Algebra Education
Linear combinations, span, and basis vectors | Chapter 2, Essence of linear algebra
Solving Matrix Equations
Linear Algebra Example Problems - Spanning Vectors #1
How do we solve a system of linear equations using any method
Simultaneous Equations Matrix Method : ExamSolutions
Linear Equations - Algebra
Linear Algebra Example Problems - Linear Combination of Vectors #2
1.5 Solution Sets of Linear Equations
Linear Algebra 1.5.1 Homogeneous System Solutions
Solving simultaneous equations by substitution
Linear Algebra - Matrix Operations
Комментарии
 0:14:19
0:14:19
![[Linear Algebra] Solution](https://i.ytimg.com/vi/mgyNwAemN7I/hqdefault.jpg) 0:11:25
0:11:25
 0:07:30
0:07:30
 0:07:18
0:07:18
 0:01:19
0:01:19
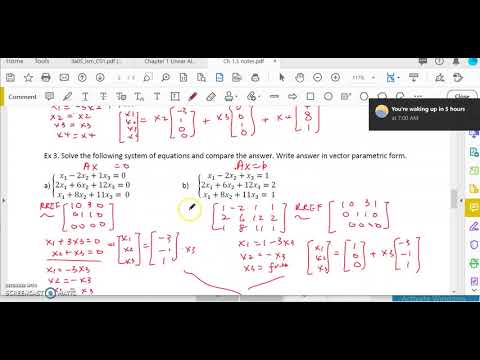 0:15:00
0:15:00
 0:10:52
0:10:52
 0:02:36
0:02:36
 0:53:39
0:53:39
 0:15:31
0:15:31
 0:18:40
0:18:40
 0:05:15
0:05:15
 0:01:54
0:01:54
 0:09:59
0:09:59
 0:06:31
0:06:31
 0:04:31
0:04:31
 0:04:34
0:04:34
 0:09:23
0:09:23
 0:32:05
0:32:05
 0:03:53
0:03:53
 1:01:05
1:01:05
 0:17:05
0:17:05
 0:05:19
0:05:19
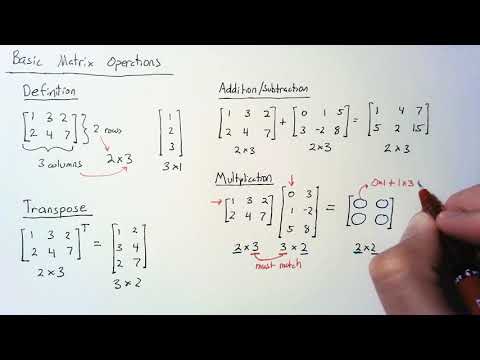 0:07:08
0:07:08