filmov
tv
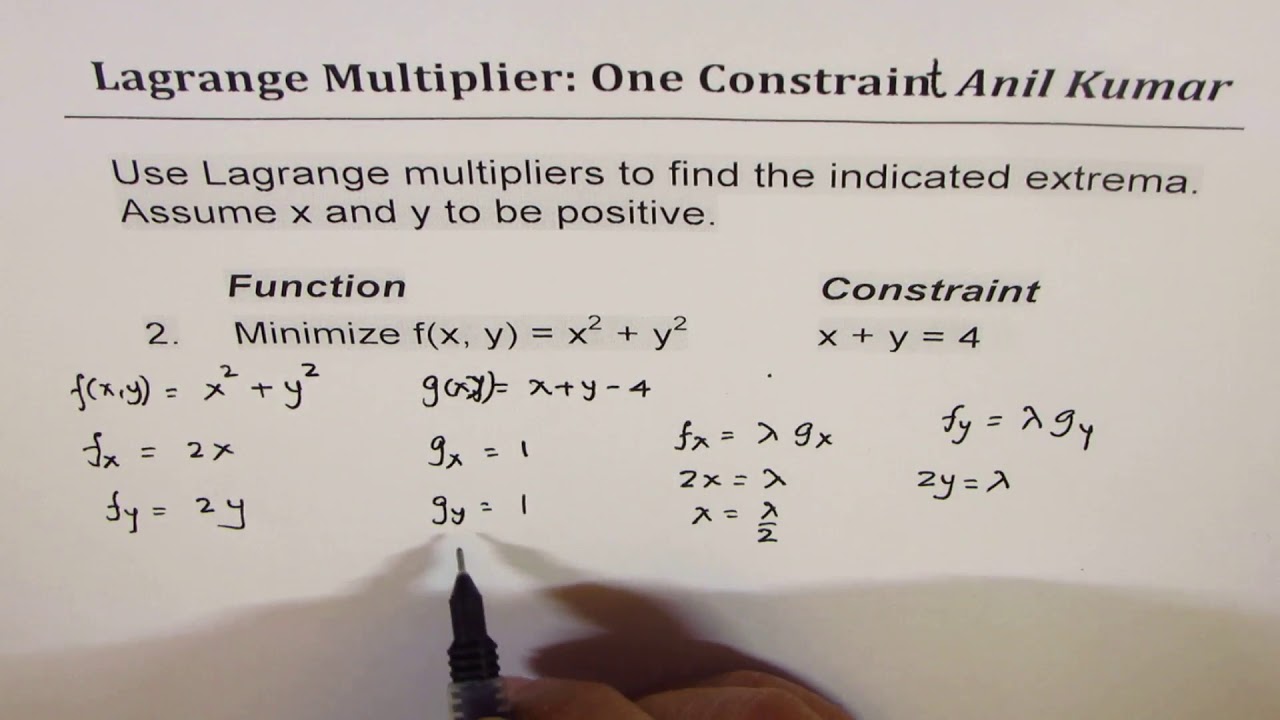
Lagrange Multipliers One Constraint Two Variable Opimization Examples
Показать описание
#Lagrange_Multipliers #multivariablecalculus_Extrema #globalmathinstitute #Calculus_Curve_Sketching_Detials #Increasing_Decreasing_Interval #GCSECalculus #CalculusAnilKumar #ibslmath
Critical number is the point where the derivative is zero or undefined. Maximum occurs where the derivative changes from positive to negative. Minimum is at the point where the first derivative changes from negative to positive increasing to decreasing. Minimum is at a point where the derivative changes from decreasing to increasing. Second derivative can be used to confirm maximum or minimum at the critical point. Positive second derivative means minimum and negative means maximum.
Critical number is the point where the derivative is zero or undefined. Maximum occurs where the derivative changes from positive to negative. Minimum is at the point where the first derivative changes from negative to positive increasing to decreasing. Minimum is at a point where the derivative changes from decreasing to increasing. Second derivative can be used to confirm maximum or minimum at the critical point. Positive second derivative means minimum and negative means maximum.
0:25:52
Lagrange Multipliers One Constraint Two Variable Opimization Examples
0:16:29
Lagrange Multipliers with TWO constraints | Multivariable Optimization
0:08:43
Lagrange multipliers, using tangency to solve constrained optimization
0:33:46
Lagrange Multipliers
0:12:24
Lagrange Multipliers | Geometric Meaning & Full Example
0:17:09
Lagrange Multipliers Practice Problems
0:24:39
🟡15a - Lagrange's Multipliers: One Constraints - Find the maximum and minimum | Ex 1 - 3
0:19:41
🟡15b - Lagrange's Multipliers: Two Constraints - Find the maximum and minimum | Ex 4 & 5
0:15:50
Lagrange Multiplier Method with Two Equality Constraints
0:58:33
Calculus 3 Lecture 13.9: Constrained Optimization with LaGrange Multipliers
0:14:10
Lagrange’s Multipliers (NLPP with 2 Variables and 1 Equality Constraints) Problem
0:11:29
Lagrange Multipliers with Two Constraints
0:14:24
Lagrange multipliers: 2 constraints
0:20:44
Lagrange Multiplier Method with one constraint
0:10:17
lagrangians in economics: constrained optimization
0:07:50
How to Use Lagrange Multipliers with Two Constraints Calculus 3
0:13:18
Understanding Lagrange Multipliers Visually
0:10:24
LM5: Lagrange Multipliers with Two Constraints
0:21:50
Lagrange multiplier two constraints
0:10:49
Constrained Optimization: Intuition behind the Lagrangian
0:09:27
Lagrange multipliers and constrained optimization with two constraints
0:21:36
Lagrange Multipliers - Two Constraints
0:08:33
lagrange multipliers, three dimensions one constraint (KristaKingMath)
0:06:29
Constrained optimization introduction
Комментарии