Lagrange multipliers, using tangency to solve constrained optimization

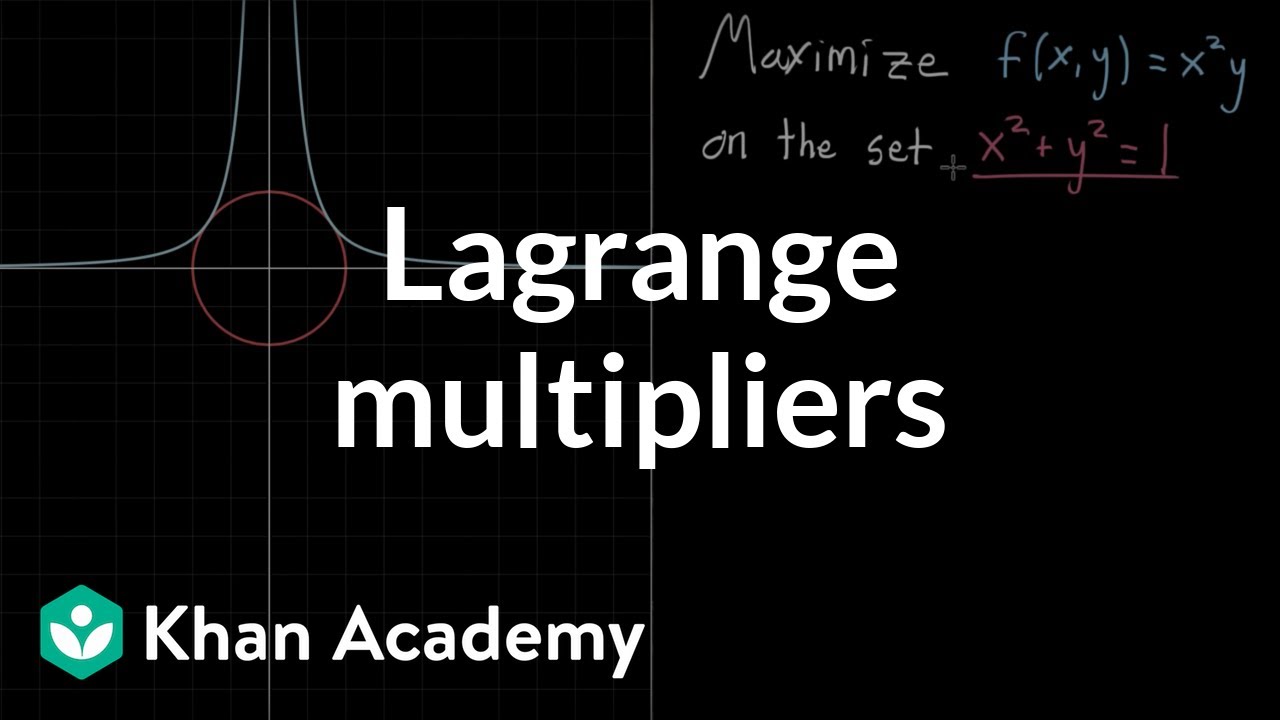
The Lagrange multiplier technique is how we take advantage of the observation made in the last video, that the solution to a constrained optimization problem occurs when the contour lines of the function being maximized are tangent to the constraint curve.

[Burneynator]

Somehow you've managed to compress a 1 hour long lecture into 9 minutes long video with better explanations than my lecturer, thanks a lot! :)

[hoodarrock]

the new guy for khan academy is so mathematical ... I love his explanations so much they are so deep instead of just giving a set of techniques and methods on how to solve exams he gets in the core of things... that's what we always for in Khan Academy

[Cyrusislikeawsome]

This guy is just maths bae. Best maths channel on YouTube and best Khan Academy videos for maths. what a beast.

[garronfish]

I did this in University 2nd year Maths and basically came to the conclusion that it was magic. Now I'm starting to understand it thank you so much!

[eliasminkim]

this is divine. This just cleared my mind up 😭😭 your explanations are so clear and mathematical, yet intuitive! Thanks a lot 😊

[saahilnayyer]

Khan Academy has really revolutionized learning. Today we have so many online learning platforms and all of these are in a way off-springs of Khan Academy. Topic wise learning makes the hour long lecture approach of colleges redundant. Most professors at universities are very knowledgable no doubt but not so great educators. To be able to impart the knowledge you hold is an art. Cheers to Khan Academy.

[hellelo.]

3blue1brown Congratulation, I love the fact you are working with Khan Academy, thats great...

[spencertaylor]

Grant hits that yeet again. What a boss

[ednaT]

With math it's always the same way: When you don't understand it, it's hell but when you got it, it's pretty cool. :)
Thank you for such a nice explanation!

[fjgozzi]

I´ve just contributed pt-br subtitles, please accept them so that this great material is available to a larger audience!

[maxbardelang]

4:27 Though his name may sound French, Lagrange was actually Italian. Actually he was born Italian, his birth name beeing Lagrangia, then migrated to France and changed his name.

[turbopotato]

I havent watched the video yet and have no idea what Lagrange multipliers are, but here is how I'd do it:
1=x^2 +y^2
x=sqrt(1-y^2)

f(x, y)=x^2y
f(y)= (1-y^2)y= y - y^3
f'(y)=1- 3y^2 = 0
y = +-sqrt(1/3)
x = +-sqrt(2/3)

f(+ - sqrt(2/3), + - sqrt(1/3))= + - 2*sqrt(1/3)/3

[MrSkizzzy]

This was so well explained that i'd call it a masterpiece.

[mantacid]

I am literally Watching this the day before my final, and this is way better than how my textbook went about this.

[poiuwnwang]

f = lambda*g is super. I learned that in university, but his explanation is really insightful.

[rfolks]

Lagrange was Italian. I don't know why, but we know him by his French name "Joseph Louis Lagrange" rather than his Italian name: "Giuseppe Luigi Lagrangia".

[kylewolfe_]

Wow, was not expecting to get an explanation from Grant when I clicked on a Khan Academy video. Very cool!

[franciscorivas]

Thank you very much!! this explanation is life-saving. I'm trying to understand Lagrange duality for support vector machines and I've watched many videos but I'm still stuck. Now I have a better taste of what it is about.

[nevgongivuup]

Thanks for saving my life, Grant. You are the best.❤

[nahblue]

While the lagrange method with lambda is great to learn, it is actually a lot less gruel in examples such as these to solve the equations without involving lambda. Take the requirement grad f || grad g and write it as a determinant, det(fx fy; gx gy) == 0 <=> grad f and grad g are parallel; that's one equation and the constraint is another equation -> two equations and two unknowns. :)