filmov
tv
Why is Pi Everywhere? 5 Levels from Basics to the Unexpected
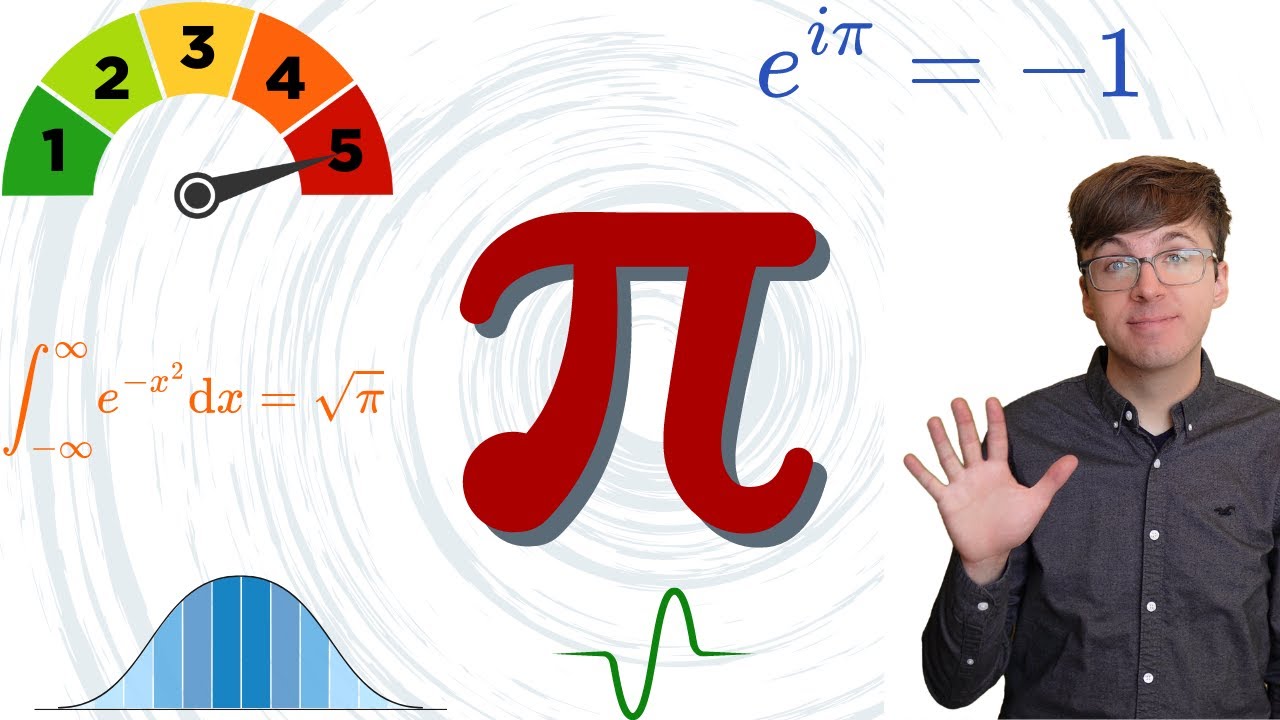
Показать описание
Why does pi show up everywhere, even when there are no circles in sight? Let's explore pi in 5 levels, ranging from geometry to its surprise appearances in complex numbers, calculus, and probability!
This video is sponsored by Squarespace.
00:00 Introduction
00:26 Level 1: Circles & Distance
01:18 Level 2: Trigonometry
03:24 Sponsor Message
04:15 Level 3: Complex Numbers
07:01 Level 4: Gaussian Integral
09:52 Level 5: Central Limit Theorem
Why is Pi Everywhere? 5 Levels from Basics to the Unexpected
Why Pi is Awesome (Vi Hart Rebuttal)
e^(iπ) in 3.14 minutes, using dynamics | DE5
Why is pi here? And why is it squared? A geometric answer to the Basel problem
Why is Pi Number Everywhere? / Pi Number
The most unexpected answer to a counting puzzle
The Most Unusual Ways Pi Shows Up In Mathematics | Can You Explain These?
Math Antics - Circles, What Is PI?
3 Ways Pi Can Explain Almost Everything
Does the number Pi actually exist?
The Pi Alphabet Clock #shorts
Pi is Beautiful - Numberphile
Pi's Secret Twin
What's so special about Euler's number e? | Chapter 5, Essence of calculus
3 Uses of Pi in Daily Life | Why do we need Pi? #Pi #365daysofmath
Why Orange Pi 5 Became a Classic and Earned the Love of Developers Everywhere
The Golden Ratio: Is It Myth or Math?
Why do colliding blocks compute pi?
the Raspberry Pi 5
The magic of Fibonacci numbers | Arthur Benjamin | TED
The Curious Case of Pi Earth: The Planet with a Mathematical Orbit
The mathematical secrets of Pascal’s triangle - Wajdi Mohamed Ratemi
Is math discovered or invented? - Jeff Dekofsky
3-4-5 Triangle and π (Pi)
Комментарии
 0:12:22
0:12:22
 0:07:11
0:07:11
 0:04:08
0:04:08
 0:17:08
0:17:08
 0:06:10
0:06:10
 0:05:13
0:05:13
 0:14:01
0:14:01
 0:07:58
0:07:58
 0:04:54
0:04:54
 0:08:30
0:08:30
 0:00:41
0:00:41
 0:08:24
0:08:24
 0:00:21
0:00:21
 0:13:50
0:13:50
 0:00:33
0:00:33
 0:03:25
0:03:25
 0:22:55
0:22:55
 0:15:16
0:15:16
 0:17:57
0:17:57
 0:06:25
0:06:25
 0:00:56
0:00:56
 0:04:50
0:04:50
 0:05:11
0:05:11
 0:02:07
0:02:07