filmov
tv
sqrt(i)

Показать описание
------------------------------------------------------------------------------------
------------------------------------------------------------------------------------
bprp #shorts
------------------------------------------------------------------------------------
bprp #shorts
sqrt(i)
This Is My New Favorite Number
sqrt(i) | square root of i
sqrt(i+sqrt(i+sqrt(i+...))) = ?
sqrt(i) in polar form
sqrt(i^4)=?
The square root of i || sqrt(i) || root i Explained
sqrt(i)
Coding the self attention mechanism with key, query and value matrices
Square root of i
i-th root of i
A Wonderful Math Problem. Square root i + Square root -i =?
Find the value of sqrt(i)
why sqrt(36) is just positive 6
sqrt(i) as a complex number
5 simple unsolvable equations
Find the square root of i | Sqrt(i)
A beautiful Olympiad Math Problem Sqrt. i | A Nice Olympiad Problem
sqrt (i)
Solving: sqrt(i) + sqrt(-i)
sqrt(i) but ASMR
` sqrt i = `
sqrt(i) | How to find the square root of i
How to find square root of iota || √i=? || sqrt(i)=?
Комментарии
 0:09:02
0:09:02
 0:03:28
0:03:28
 0:01:28
0:01:28
 0:06:30
0:06:30
 0:10:59
0:10:59
 0:01:10
0:01:10
 0:02:57
0:02:57
 0:00:44
0:00:44
 1:19:08
1:19:08
 0:03:10
0:03:10
 0:01:52
0:01:52
 0:03:55
0:03:55
 0:02:28
0:02:28
 0:01:42
0:01:42
 0:04:42
0:04:42
 0:00:50
0:00:50
 0:04:27
0:04:27
 0:10:55
0:10:55
 0:04:20
0:04:20
 0:01:01
0:01:01
 0:00:53
0:00:53
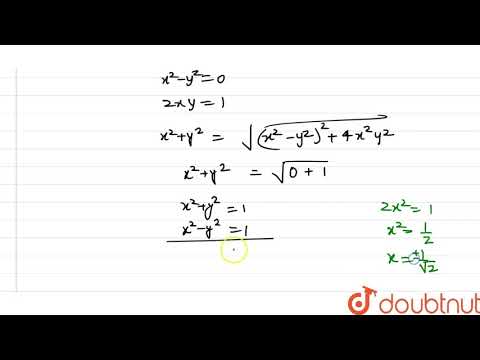 0:02:26
0:02:26
 0:12:06
0:12:06
 0:07:15
0:07:15