filmov
tv
Intro Real Analysis, Lec 11: Continuity and the Intermediate Value Theorem (climbing Monk Story)
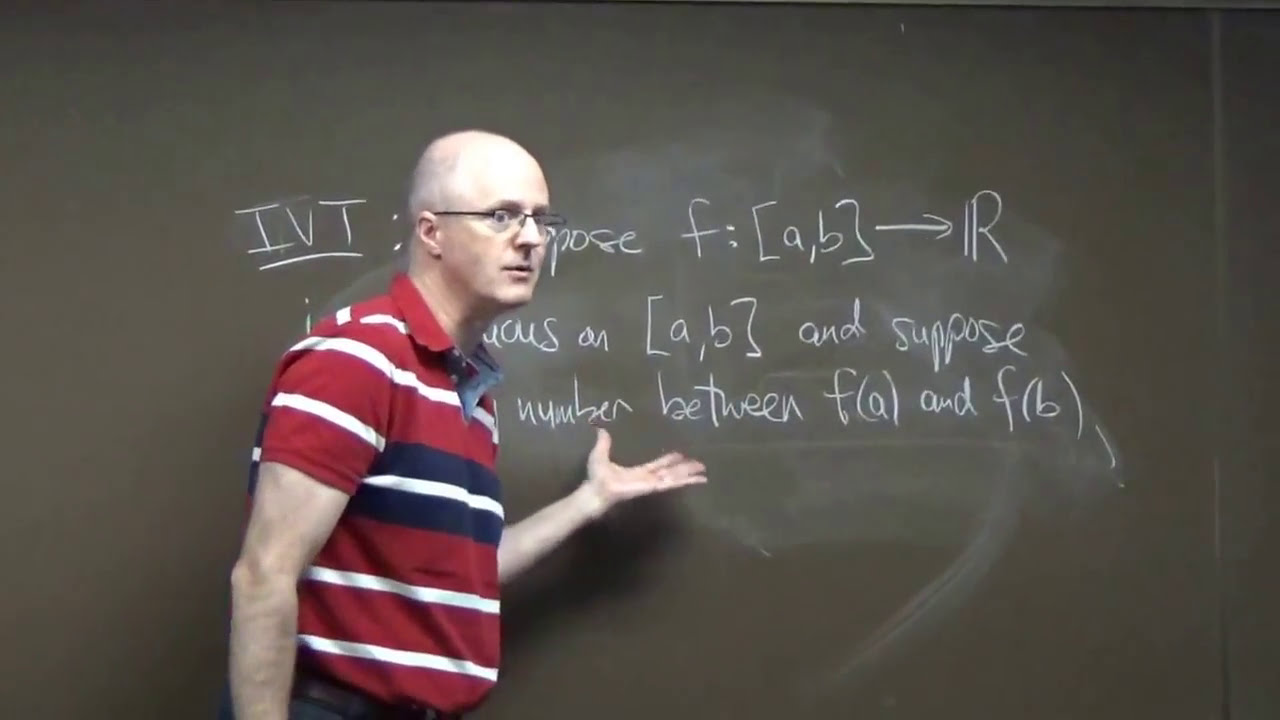
Показать описание
Lecture 11.
(0:00) Announcements.
(0:24) The Story of a Monk on a Mountain (will be at exactly the same point at the same time on two different days, by continuity and the IVT).
(5:23) The Intermediate Value Theorem statement.
(11:13) Outline of how to apply it to proving a certain polynomial has at least three real roots.
(18:32) Scratchwork to prove a fact about a limit of a rational function (it ends up being harder than I thought it would be at first and I end up just thinking about a special case).
(33:57) Epsilon delta definition of continuity at a point.
(38:25) The essence of continuity and ways a function can fail to be continuous at a point.
(43:12) Functions with holes come up in calculus.
(46:08) Continuity of arithmetic combinations of functions.
(48:06) Continuity of a composition (best proved using the relationship between limits of sequences and limits of functions).
(52:54) Theorems as work-saving devices for complicated situations.
Bill Kinney, Bethel University Department of Mathematics and Computer Science. St. Paul, MN.
AMAZON ASSOCIATE
As an Amazon Associate I earn from qualifying purchases.
Intro Real Analysis, Lec 11: Continuity and the Intermediate Value Theorem (climbing Monk Story)
Intro Real Analysis, Lec 12: Limits Involving Infinity, Continuity, Intermediate and Extreme Values
RA1.1. Real Analysis: Introduction
Intro Real Analysis, Lec 19, Part 3: Review for Real Analysis Exam 2
Intro Real Analysis, Lec 24: Convergence of Series, Geometric Series, p-Series Test, Divergence Test
Intro Real Analysis, Lec 29: The Most Beautiful Equation in the World, Taylor Series Calculations
Intro Real Analysis, Lec 19, Part 1: Conditions for Riemann Integrability
Intro Real Analysis, Lec 17: Mean Value Theorem Corollaries, Definition of Riemann Integral
Intro Real Analysis, Lec 37, Part 1: Taylor Series, Cantor Sets & Logistic Map, Review Topology
Introductory Calculus: Oxford Mathematics 1st Year Student Lecture
Intro Complex Analysis, Lec 11, Areas of Images, Differentiability, Analyticity, Cauchy-Riemann Eqs
Intro Real Analysis, Lec 22: Review Properties of Integrals and Fundamental Theorem of Calculus
Intro Real Analysis, Lec 33: Euclidean Metric, Triangle Inequality, Metric Spaces, Compact Sets
Intro Real Analysis, Lec 16, Part 2: Summary of Corollaries of the Mean Value Theorem
HP20BM3RA-11 | B.Sc. Maths | Real Analysis | Lec - 11
Intro Real Analysis, Lec 21: Convergence of Riemann Sums, Fundamental Theorem of Calculus
Intro Real Analysis, Lec 35: Sup Norm and Metric on C[a,b], Sequence Space, Open & Closed Sets
Intro Real Analysis, Lec 8: Subsequences, Bolzano-Weierstrass, Cauchy Criterion, Limsup & Liminf
Intro Real Analysis, Lec 36: Chaos, Logistic Map, Period Doubling, Symbol Space, Shift Map
Mod-11 Lec-32 Introduction to Marty's Theorem
Intro Real Analysis, Lec 5: Archimedean Property of R, Cantor's Theorem, Sequences, Crazy Funct...
Intro Real Analysis, Lec 18: Optimization Exs, Step Functions are Riemann Integrable
Mod-11 Lec-33 Proof of one direction of Marty's Theorem
Necessity of complex numbers
Комментарии
 0:56:28
0:56:28
 0:56:35
0:56:35
 0:10:41
0:10:41
 0:10:34
0:10:34
 0:53:40
0:53:40
 0:55:40
0:55:40
 0:23:59
0:23:59
 0:56:27
0:56:27
 0:21:54
0:21:54
 0:58:03
0:58:03
 0:59:16
0:59:16
 0:54:54
0:54:54
 0:57:32
0:57:32
 0:20:54
0:20:54
 1:00:58
1:00:58
 0:56:16
0:56:16
 0:58:40
0:58:40
 0:57:23
0:57:23
 0:59:46
0:59:46
 0:44:07
0:44:07
 0:55:02
0:55:02
 0:56:52
0:56:52
 0:44:47
0:44:47
 0:07:39
0:07:39