filmov
tv
Intro Real Analysis, Lec 17: Mean Value Theorem Corollaries, Definition of Riemann Integral

Показать описание
Introduction to Real Analysis, Lecture 17.
(0:00) Announcements.
(1:23) Lecture plan.
(1:41) Warnings related to the Increasing Function Theorem: 1) the converse of the Increasing Function Theorem is false (f(x) = x^3 is strictly increasing over an interval but f'(0) = 0), 2) just because f'(c) is positive at some number c, does NOT mean that f is increasing on some open interval containing c (example: f(x) = x/2 + x^2*sin(1/x) when x is nonzero and f(0) = 0, f'(0) = 1/2 but f is not increasing on any open neighborhood of the origin).
(7:50) Constant Function Theorem (CFT). This is not contradicted by the floor function. You should be able to prove the CFT with the Mean Value Theorem (MVT).
(11:43) Any two antiderivatives of the same function over some interval differ by a constant.
(13:10) Other applications: 1) first derivative test, 2) and 3) general solutions of differential equations (do a proof related to this), 4) Fundamental Theorem of Calculus.
(26:57) Prove (1 + x)^(1/3) is less than or equal to 1 + x/3 for all positive x with the Mean Value Theorem (and give statement of a more general case).
(31:30) Be able to use L'Hopital's Rule (do a couple examples).
(38:35) Derivatives satisfy the intermediate value property and various other definitions and facts (related to, for example, concave up and concave down).
(42:52) Definition of Riemann integrability (what it means for a function to be Riemann integrable over a closed and bounded interval, using the idea of a tagged partition and corresponding Riemann sum).
Bill Kinney, Bethel University Department of Mathematics and Computer Science. St. Paul, MN.
AMAZON ASSOCIATE
As an Amazon Associate I earn from qualifying purchases.
Комментарии
 0:56:27
0:56:27
 0:10:34
0:10:34
 0:08:32
0:08:32
 0:23:59
0:23:59
 0:34:24
0:34:24
 0:55:40
0:55:40
 0:23:59
0:23:59
 0:56:52
0:56:52
 0:54:44
0:54:44
 0:53:40
0:53:40
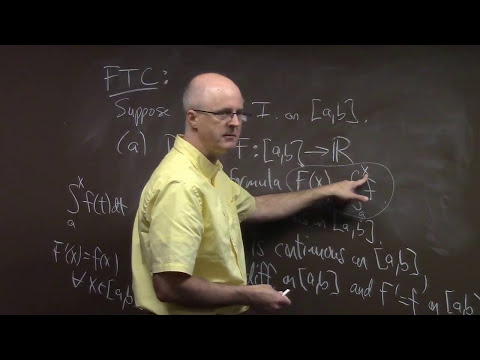 0:56:16
0:56:16
 0:54:06
0:54:06
 0:54:54
0:54:54
 0:56:28
0:56:28
 0:20:54
0:20:54
 0:12:35
0:12:35
 0:43:05
0:43:05
 0:06:48
0:06:48
 0:51:38
0:51:38
 0:35:26
0:35:26
 0:48:09
0:48:09
 0:56:16
0:56:16
 0:57:24
0:57:24
 0:16:10
0:16:10