filmov
tv
Fourier's Proof that e is Irrational

Показать описание
We prove that e is irrational by examining its series expansion and asking for a little bit of help from our friend Joseph Fourier.
Your support is a heartfelt source of encouragement that propels the channel forward.
Please consider taking a second to subscribe in order to express your valuable support and receive notifications for the latest videos!
Any likes, subscriptions, comments, constructive criticisms, etc., are wholeheartedly appreciated.
Your support is a heartfelt source of encouragement that propels the channel forward.
Please consider taking a second to subscribe in order to express your valuable support and receive notifications for the latest videos!
Any likes, subscriptions, comments, constructive criticisms, etc., are wholeheartedly appreciated.
Fourier's Proof that e is Irrational
A proof that e is irrational - Numberphile
What's so special about Euler's number e? | Chapter 5, Essence of calculus
But what is the Fourier Transform? A visual introduction.
e (Euler's Number) - Numberphile
e^(iπ) in 3.14 minutes, using dynamics | DE5
Fourier Transform Equation Explained ('Best explanation of the Fourier Transform on all of YouT...
how to get the Fourier series coefficients (fourier series engineering mathematics)
Parseval's Identity _ Example 4 I Fourier Transform
Eulers formula
Taylor series | Chapter 11, Essence of calculus
Complex Fourier Series (fourier series engineering mathematics)
Fourier Series introduction
Properties of Fourier Series
The Short Time Fourier Transform
The more general uncertainty principle, regarding Fourier transforms
Fourier Series and their Convergence (Proof!) (Theory of Machine Learning)
But what is a Fourier series? From heat flow to drawing with circles | DE4
Using Fourier integral representation, show that
What is Fourier Transform? Proof of Fourier Integral theorems
Proof of Convolution Theorem of Fourier Transform - Advanced #Calculus by #Moein
Find the function using inverse Fourier transform where f(s)= e^-|s|y
Fourier Transform of Basic Signals (Cosω₀t)
Fourier Transform of Basic Signals (Impulse Signal)
Комментарии
 0:03:04
0:03:04
 0:16:29
0:16:29
 0:13:50
0:13:50
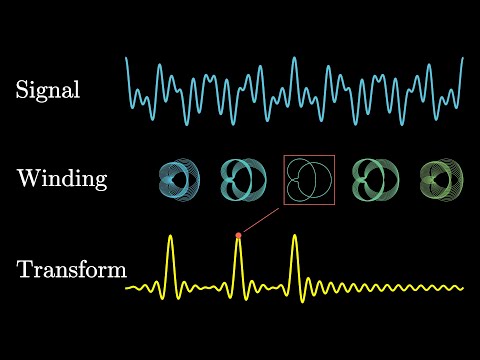 0:20:57
0:20:57
 0:10:42
0:10:42
 0:04:08
0:04:08
 0:06:26
0:06:26
 0:20:17
0:20:17
 0:10:42
0:10:42
 0:08:42
0:08:42
 0:22:20
0:22:20
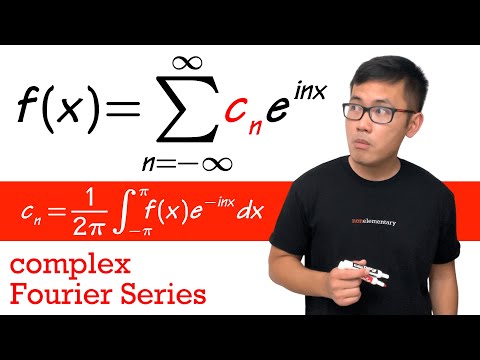 0:12:57
0:12:57
 0:05:12
0:05:12
 0:10:43
0:10:43
 0:00:58
0:00:58
 0:19:21
0:19:21
 0:21:41
0:21:41
 0:24:47
0:24:47
 0:17:53
0:17:53
 0:29:53
0:29:53
 0:07:39
0:07:39
 0:08:28
0:08:28
 0:03:36
0:03:36
 0:03:16
0:03:16