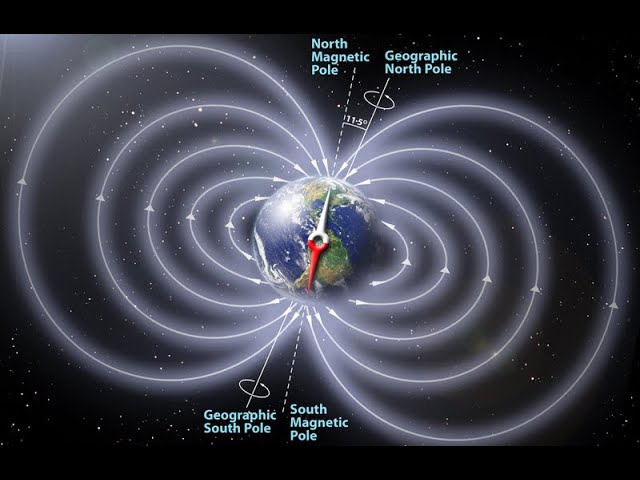
Will Earth's magnetic reversal cause catastrophe? (Part 2 of 'Did the CIA classify...?)

Part one of this video is here:

TO SUPPORT THIS CHANNEL -- PLEASE SUPPORT THIS CHARITY:

CORRECTIONS:

1) 13:01 – I said ‘kilometers an hour’ instead of ‘miles an hour.’

2) As expected, someone corrected my understanding of the torque required to stop the Earth's rotation. The formulaa I used is for a uniform sphere, whereas the Earth has a much denser center and a lighter crust. So the torque would be slightly less than I calculated. But in non-scientific terms, still a shitload.

3) Another poster wrote: "The unit of torque is Nm [Newton-meter] not Nm/s."

4) @anttibra, a physics teacher from Finland, corrected my calculation. On the basis that he knows better then me, I'm happy to lay it out here. He wrote:
Torque is calculated by this equation: M=F x r, where x is vector product. If force is plumb compared to distance of rotating axis, then you can use simple multiplication.
Angular momentum is L=J*w, where J=moment of inertia (similar to mass in rotation) and w=angular velocity.
Assuming Earth is uniform sphere (it's not, but this is simplified math), then J=2/5*Mr^2=9,721052869650016e37 kgm^2
Since Earth rotate once every 23h 56min 4,10 seconds, then angular velocity w=2*pi/(86164,1 s)=7,292*10^-5 1/s.
Now we get Earth's angular momentum L=7,08870359707824214497e33 kgm^2/s.
When we want to stop the Earth, we must apply torque over time.
delta L=M*delta t, delta t = time we apply torque. If the Earth stops in 10 hours, then delta t= 36000 seconds.
M=L/t=7,08870359707824214497e33 kgm^2/s / (36000 s) = 1,96908433252173392916e29 Nm.
How much is this? Well, if we apply force on the equator, the required force would be:
M=F*r
F = M/r = 1,96908433252173392916e29 Nm / (6 378 140 m) = 3,08723912068680513309e22 = 31e21 N = 31 ZN = 31 000 000 000 000 000 000 000 N.

Another poster added: "You have this line as part of your corrections: 'Since Earth rotate once every 23h 56min 4,10 seconds, then angular velocity w=2*pi/(86164,1 s)=7,292*10^-5 1/s.' The units are in radians per second, but the unit written have been written as rotations per second (Hz or 1/s).


SOURCES:

Earth Catastrophe Cycle | Pole Shift
Suspicious0bservers

An overview of Earth’s magnetic field

Strength of field falling:

Magnetic field over 9,000 years:

Equations of inertia;

The Earth’s magnetic field – an overview

How Does A Magnetic Reversal Affect Life On Earth?
RapidChangesinStrengthandDirectionofEarth'sMagneticFieldOverthePast100,000Years

[potholer]

MICRONOVA -- I got a couple of posts asking about Ben Davidson’s ‘micronova’ claim.
@Princip666 wrote:
“I just wonder why did you leave out the sun going [micro]nova part from Ben's claims, as that is supposedly the event that unlocks the crust. Is it intentional, or just an oversight?”

@besticudcumupwith202 wrote:
“if you're debunking Ben Davidson you're doing a poor job of it. The micro nova causes the crustal shift. And it's not every time there's a pole shift. We have lots of those compared to this cyclical solar thing. 12k & 6k years, with the 12k being the micro nova.”

Yes, I did miss it out because it has nothing to do with our solar system. But if you want a complete debunk of every bit of crap Ben spouted in those clips I showed, I’m more than happy to oblige:

A micronova is an event that occurs on white dwarves in binary systems . A white dwarf is a star that has exploded and shrunk to a small size 1 million times more dense than our sun. If it is in a binary system with another star, it can suck hydrogen out of that star. When the hydrogen reaches the surface of a white dwarf, it fuses into helium, causing a short, intensive burst of energy called a micronova.
This was discovered in 2022, and here are a few references:
1) "Astronomers discover micronovae, a new kind of stellar explosion, " by the European Southern Observatory.
2) "Localised thermonuclear bursts from accreting magnetic white dwarfs" Scaringi et al.,
3) "Triggering micronovae through magnetically confined accretion flows in accreting white dwarfs, " by Scaringi et. al.

It’s hard to tell from the video what Ben is claiming these distant micronovae can affect the Earth, but if he thinks thermonuclear explosions hundred of trillions of miles from Earth and not visible to the naked eye can affect the crust, that fallacy speaks for itself. Or if he thinks the sun can produce a micronova, in which case he doesn’t understand what a micronova is.
The reason sun cannot produce a micronova is because it is not a white dwarf. Neither is it part of a binary system. So it can’t produce a micronova.
If you want to ask ‘How do you know?’ the answer is – again – that it is not a white dwarf, and it is not part of a binary system. It cannot such hydrogen out of another star that does not exist.
If you want to argue that it's possible, becaause sscience doesn't know everything, my answer is that it's not possible, and while it's true that science doesn't know everything, we do know that there is only one star in our solar system, not two.
If you want to argue that the sun can produce superflares and great bursts of energy – yes it can, and I already covered that in the video. But they are not micronovae.

[jonw]

I'm a simple man, I see potholer54 and I click.

[HunterYavitz]

The tragedy is that channels like this don't have multi-million subscribers.

[t-rexgamer]

Umm yes... Magneto flipped the poles and nearly ended the world. This isn't a question that hasn't been answered

[scottwills]

"There's no such thing as overdue in a random system" 👍

[bjornfeuerbacher]

The fact that many people think "Suspicious observer" is a reliable source does not speak well for basic science education in the USA. :/

[BerndBausch]

My plumber does a great job fixing my teeth. My car mechanic is a nurse, and guess what, I always reach out to the local florist when my toilet is clogged. Likewise, when I have questions about geology, I get help from my uncle Dave Dunning, whose law degree perfectly equips him to answer them. Alternatively, my colleague Justin Kruger from marketing.

[eyrcuoi]

I find it very depressing that Potholer felt it necessary to make this video - but very glad that he did.

[WentzCraft]

I'm surprised my house doesn't turn upside down every time my AC current switches polarities

[trunkage]

"Come on rock. Come here"
I almost spit my coffee out

[andrewj]

"The crust isn't magnetic" is a fine argument, but even better: Our evidence for prehistoric polar flips is the flipping orientation of magnetic elements *_in the crust!_* Everywhere in the world, rocks that formed at one time all have magnetic orientation in one direction, and rocks formed at a different time are oriented in a different direction. If the crust rotated *_with_* the poles, then the orientation of magnetic elements in rocks would always be the same relative to the crust and wouldn't provide any evidence for the shifting poles.

It's a self-defeating argument. The very fact that we know about the shifting poles proves that the crust doesn't follow those shifts.

[broz]

you really should put your rock on a leash until it is sufficiently trained

[RoyArrowood]

PH-"How much torque would it take?"
Me - a shit ton
PH- "if you put it in non scientific terms, a shitload"
Me - I knew it! Close enough

[williamschacht]

"A tool that started with such promise as a new and exciting learning experience and ended up as the Joe Rogan experience." and "... load of number 2." LOL 🙂

[jamesfarquhar]

I can attest that when I was a Christian and didn’t feel like part of the world I also fantasized about it’s coming destruction and the probability I’d avoid it spiritually and therefore be more clever than all those who perished, now I know my place is to be in the frying pan, in the fire, doing what I can for others for as long as I can, I am not more important than anyone else, while I did not get help from many people, the help I got from just a few got me to where I can do the same for others, Potholer54, being the journalist, poet and Man that he is helped me in ways I could never fully articulate, I’ve donated to his affiliated charity projects many times over the years and I’m about to do it again!
You should too!

[TorqueDonkeyTeethLewith]

Terrance Howard has pushed me over the edge with Rogan. A man with a platform like his bears him the responsibility for not giving a stage for Thanks Mr. PotHoler

[edo]

..."or in non-scientific terms... A Shit load!" hahahaha love it

[desperadox]

"There is a cult of ignorance in the United States, and there has always been. The strain of anti-intellectualism has been a constant thread winding its way through our political and cultural life, nurtured by the false notion that democracy means that my ignorance is just as good as your knowledge."
Isaac Asimov

[simond.]

Buster's eyeroll at the end is a perfect summary. 😆

[johannesschaller]

Jimmy reminds me of another marketing guy who ended up as prime minister of Australia. He had a poor grasp of scientific matters too, and seemed to favour supernatural explanations for just about everything.