filmov
tv
A Nice Radical Equation | 95% Failed To Solve!
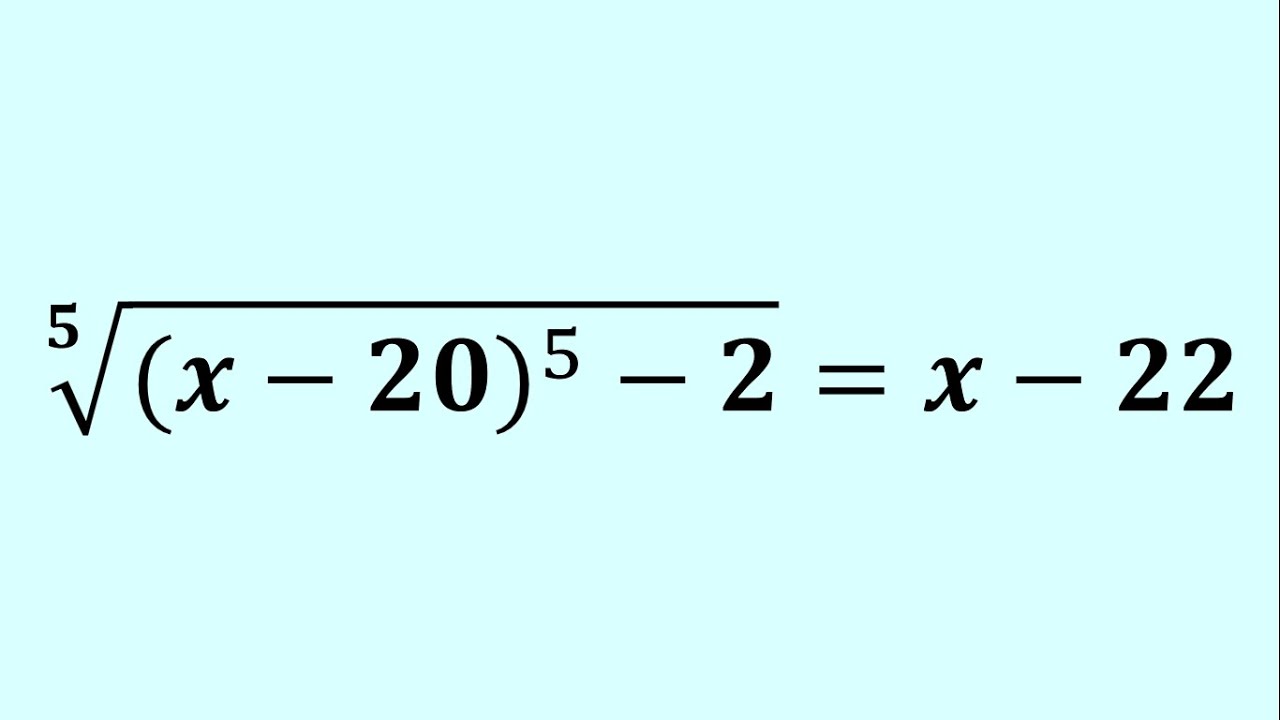
Показать описание
A Nice Radical Equation | 95% Failed To Solve!
Welcome to another exciting algebra challenge! In this video, we solve a fascinating radical equation. Join us as we delve into this intriguing algebraic challenge and explore the elegant solutions. Whether you're a math enthusiast, a student preparing for competitions, or simply love solving problems, this video is for you. Let's solve this together and enhance our problem-solving skills!
Don't forget to like, subscribe, and hit the notification bell to stay updated with more Math Olympiad challenges and math problem-solving content!
🔍 In this video:
Detailed walkthrough of a challenging algebra problem.
Tips and tricks for solving complex algebraic equations.
Encouragement to enhance your problem-solving skills and mathematical thinking.
📣 Call to Action:
Have a go at the problem yourself before watching the solution!
Share your solutions and approaches in the comments below.
If you enjoyed this challenge, give it a thumbs up and subscribe for more intriguing math problems!
#algebra #radical #math #problemsolving #learnmaths #mathematics #matholympiadpreparation
🔗 Useful Links:
Don't forget to like, share, and subscribe for more Math Olympiad content!
Thank You for Watching!!
Welcome to another exciting algebra challenge! In this video, we solve a fascinating radical equation. Join us as we delve into this intriguing algebraic challenge and explore the elegant solutions. Whether you're a math enthusiast, a student preparing for competitions, or simply love solving problems, this video is for you. Let's solve this together and enhance our problem-solving skills!
Don't forget to like, subscribe, and hit the notification bell to stay updated with more Math Olympiad challenges and math problem-solving content!
🔍 In this video:
Detailed walkthrough of a challenging algebra problem.
Tips and tricks for solving complex algebraic equations.
Encouragement to enhance your problem-solving skills and mathematical thinking.
📣 Call to Action:
Have a go at the problem yourself before watching the solution!
Share your solutions and approaches in the comments below.
If you enjoyed this challenge, give it a thumbs up and subscribe for more intriguing math problems!
#algebra #radical #math #problemsolving #learnmaths #mathematics #matholympiadpreparation
🔗 Useful Links:
Don't forget to like, share, and subscribe for more Math Olympiad content!
Thank You for Watching!!
Комментарии
 0:09:49
0:09:49
 0:06:26
0:06:26
 0:17:11
0:17:11
 0:13:59
0:13:59
 0:08:51
0:08:51
 0:02:15
0:02:15
 0:18:05
0:18:05
 0:08:49
0:08:49
 0:07:37
0:07:37
 0:12:26
0:12:26
 0:00:53
0:00:53
 0:11:29
0:11:29
 0:09:16
0:09:16
 0:08:53
0:08:53
 0:19:51
0:19:51
 0:13:35
0:13:35
 0:00:47
0:00:47
 0:00:46
0:00:46
 0:11:59
0:11:59
 0:00:33
0:00:33
 0:13:08
0:13:08
 0:03:10
0:03:10
 0:10:25
0:10:25
 0:00:39
0:00:39