filmov
tv
A Nice Radical Equation with Fifth Root

Показать описание
🤩 Hello everyone, I'm very excited to bring you a new channel (SyberMath Shorts)
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #RadicalEquations #Radicals
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #RadicalEquations #Radicals
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
A Nice Radical Equation | You Should Try it! | Math Olympiad Preparation
India | A Nice Radical Equation
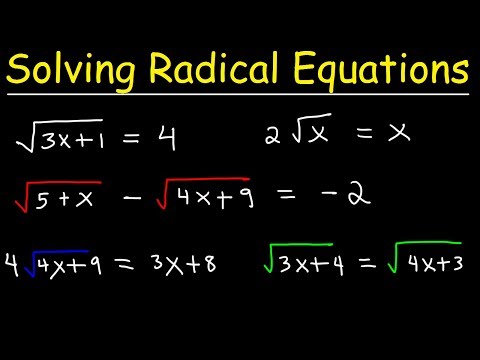
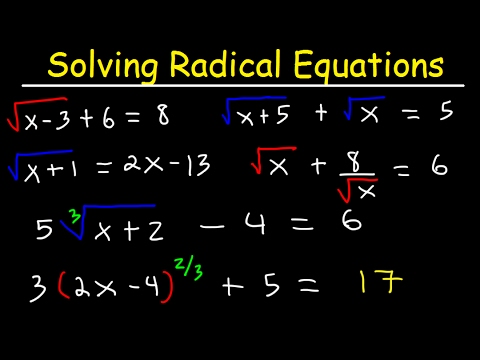
Solving Radical Equations
A Nice Radical Equation | Algebra | Math Olympiad Prep
A Nice Radical Equation with Fifth Root
A Nice Radical Equation Solved in Three Ways
Solving radical equations | Exponent expressions and equations | Algebra I | Khan Academy
A Nice Radical Equation
Nested square roots: why? when? how? USA Nice Root Simplification Radical Equation | find x
A Nice Radical Equation from Finnish Math Olympiads
Solving a Nice Radical Equation
A Nice Radical Equation
Solving A Nice Radical Equation | (√x+5)—(√4x+9)=–2 | Solution To Radical Equations | Math Tricks....
Solving A Nice Radical Equation
05 - Solving Radical Equations & Identifying Extraneous Solutions - Part 1
a nice radical equation
A nice Radical Equation | Math Olympiad | Algebra Problem | Find Real Value of 'x' ?
A nice radical equation|Find square root of product of consecutive numbers in seconds|√70*71*72*73+1...
Solving Radical Equations With Square Roots, Cube Roots, Two Radicals, Fractions, Rational Exponents
#9. Solve the Radical Equation with Two Square Roots
Can You Solve a Radical Equation?
Solving a Radical Equation Using Factoring and Checking Your Answers
Solving a Nice Radical Equation in Two Ways
Solving a nice radical equation
Комментарии
 0:00:00
0:00:00
 0:11:35
0:11:35
 0:17:11
0:17:11
 0:13:59
0:13:59
 0:08:49
0:08:49
 0:08:51
0:08:51
 0:03:11
0:03:11
 0:06:26
0:06:26
 0:03:34
0:03:34
 0:06:03
0:06:03
 0:03:24
0:03:24
 0:11:29
0:11:29
 0:13:15
0:13:15
 0:00:38
0:00:38
 0:27:27
0:27:27
 0:01:00
0:01:00
 0:14:38
0:14:38
 0:02:15
0:02:15
 0:18:05
0:18:05
 0:05:17
0:05:17
 0:00:31
0:00:31
 0:04:25
0:04:25
 0:11:59
0:11:59
 0:09:04
0:09:04