filmov
tv
Square Roots of Complex Numbers (2 of 2: Introductory example)

Показать описание
HSC Maths Ext2 - Complex Numbers - Finding Square Roots of Complex Numbers
Complex numbers : Finding Square roots of : ExamSolutions
sqrt(a+bi), how to get the square roots of a complex number
Square Roots of Complex Numbers
Finding square root of a complex number
How To Take The Square Root Of A Complex Number
How to find Square Root of Complex Number
Find Square root of Complex numbers | Scientific Calculator fx 991 es
Square Roots When Normalizing Rotors
How to Find the nth Roots of a Complex Number with the Formula
sqrt(i)
Square Roots of Complex Numbers (1 of 2: Establishing their nature)
Imaginary roots of negative numbers | Imaginary and complex numbers | Precalculus | Khan Academy
How To Find Square Root Of Complex Number. Square roots of 3-4i =?
The Maths Prof: Square Roots of Complex Numbers
Algebra 2 - How to simplify the a radical with imaginary numbers, root(-45)
Ex: Find the Square Root of a Complex Number (DeMoivre's Theorem)
Square Root of Complex Number (Part 1) | Maths Class 11
How to find the principal square root of a complex number
Square Roots of a Complex Number (2 of 3: Principal square root)
Simplify Square Roots Using Complex Numbers: Rational and Imaginary Results
Square roots of Complex numbers | Class 11 Maths
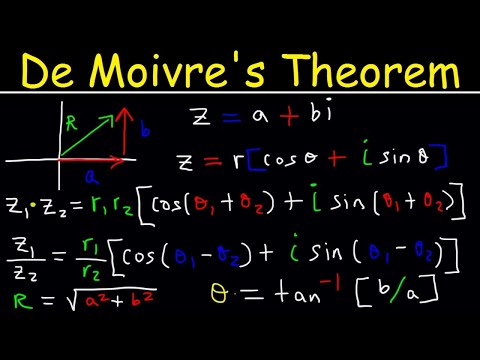
Complex Numbers In Polar - De Moivre's Theorem
How To Find The Square Root of a Negative Number
Комментарии
 0:05:26
0:05:26
 0:12:44
0:12:44
 0:07:54
0:07:54
 0:10:42
0:10:42
 0:02:14
0:02:14
 0:02:38
0:02:38
 0:09:54
0:09:54
 0:03:01
0:03:01
 0:00:50
0:00:50
 0:11:27
0:11:27
 0:09:02
0:09:02
 0:04:56
0:04:56
 0:04:04
0:04:04
 0:06:08
0:06:08
 0:10:21
0:10:21
 0:02:03
0:02:03
 0:07:05
0:07:05
 0:29:26
0:29:26
 0:00:59
0:00:59
 0:09:24
0:09:24
 0:03:45
0:03:45
 0:21:54
0:21:54
 1:04:47
1:04:47
 0:08:04
0:08:04