filmov
tv
The Goat Problem - Numberphile
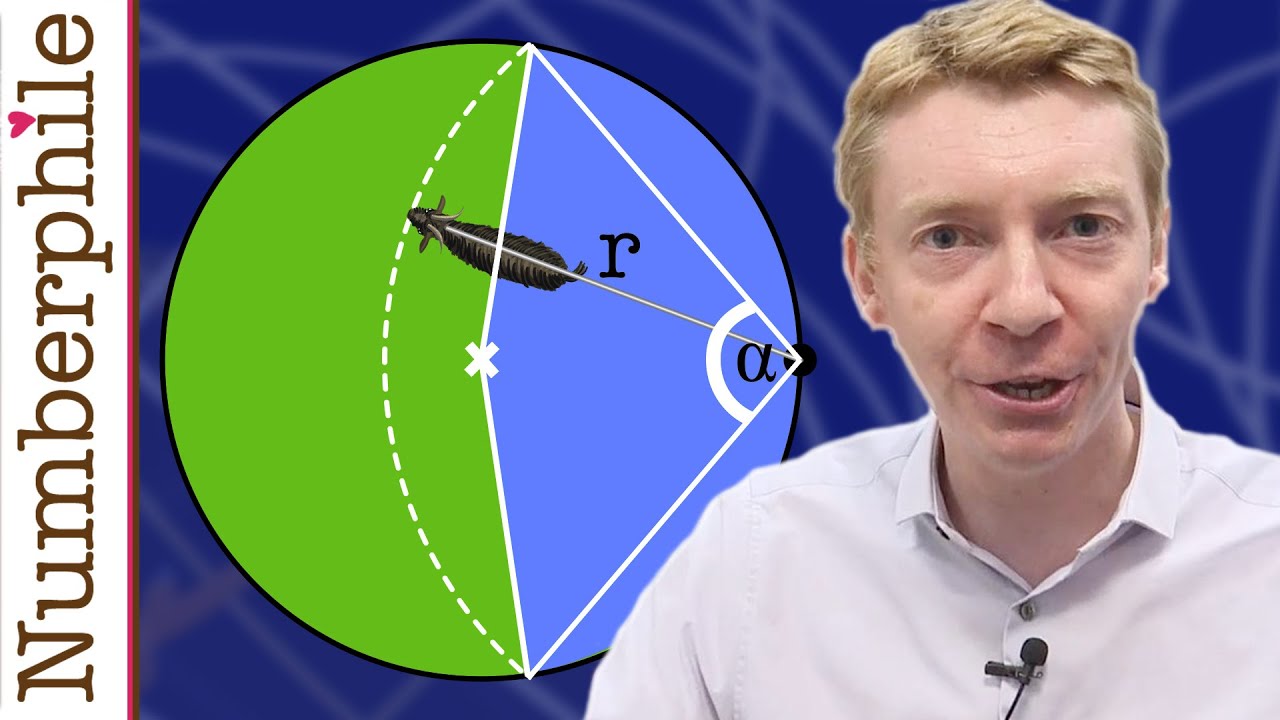
Показать описание
More links & stuff in full description below ↓↓↓
Some papers about the Goat Problem...
NUMBERPHILE
Videos by Brady Haran
Animation by Pete McPartlan
With thanks to our checkers, including János Pallagi
Some papers about the Goat Problem...
NUMBERPHILE
Videos by Brady Haran
Animation by Pete McPartlan
With thanks to our checkers, including János Pallagi
The Goat Problem - Numberphile
Monty Hall Problem - Numberphile
Monty Hall Problem (best explanation) - Numberphile
Monty Hall Problem Express Explanation
270-year-old math problem SOLVED! Grazing goat problem
The Josephus Problem - Numberphile
The Monty Hall Problem - Explained
The Monty Hall Problem
The problem in Good Will Hunting - Numberphile
The math problem that stumped thousands of mansplainers
The Light Switch Problem - Numberphile
The Brick Factory Problem - Numberphile
Game Theory Scene | 21(2008) | Now Playing
The Hydra Game - Numberphile
A Problem with the Parallel Postulate - Numberphile
Problems with Powers of Two - Numberphile
Why greatest Mathematicians are not trying to prove Riemann Hypothesis? || #short #terencetao #maths
The Monty Hall Problem: Switch Doors or Not?
The Simple Question that Stumped Everyone Except Marilyn vos Savant
Terrific Toothpick Patterns - Numberphile
The Most Controversial Problem in Philosophy
Millennium Problem-Grigori Perelman #shorts #KnowX
The Doomsday Algorithm - Numberphile
The Lazy Way to Cut Pizza - Numberphile
Комментарии
 0:16:52
0:16:52
 0:05:30
0:05:30
 0:04:18
0:04:18
 0:00:49
0:00:49
 0:01:00
0:01:00
 0:13:58
0:13:58
 0:02:48
0:02:48
 0:14:01
0:14:01
 0:04:54
0:04:54
 0:04:58
0:04:58
 0:18:31
0:18:31
 0:14:51
0:14:51
 0:03:39
0:03:39
 0:21:54
0:21:54
 0:13:45
0:13:45
 0:10:57
0:10:57
 0:00:38
0:00:38
 0:00:50
0:00:50
 0:07:06
0:07:06
 0:16:07
0:16:07
 0:10:19
0:10:19
 0:00:22
0:00:22
 0:14:33
0:14:33
 0:14:26
0:14:26