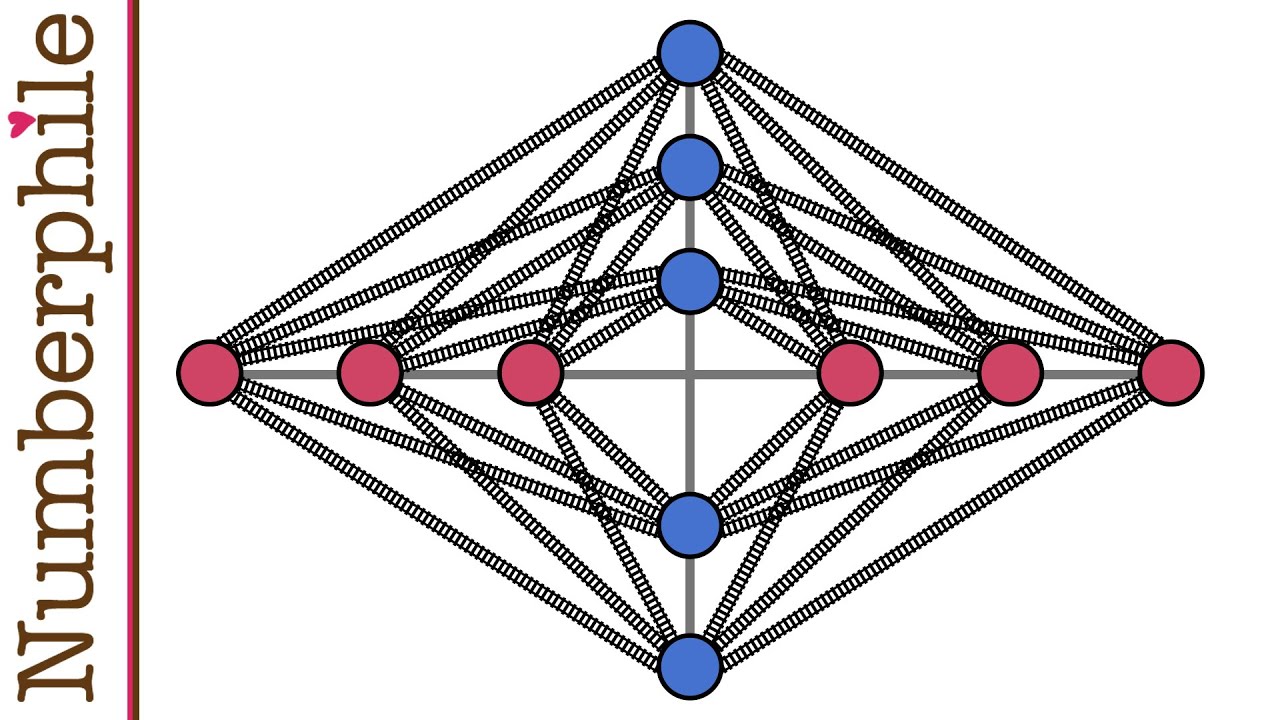
The Brick Factory Problem - Numberphile

More links & stuff in full description below ↓↓↓

NUMBERPHILE

Videos by Brady Haran
Animation by Pete McPartlan
Thanks Michael Colognori from the Numberphile Society for error spotting!

[nguyenbuihamy]

so long since I last watched Numberphile… James was my favourite guest every time!!!! Love that he ages beautifully

[jhonbus]

To me, the obvious solution is to fix the track crossings rather than minimise the amount you use them. But maybe that's why I'm an engineer rather than a mathematician...

[nicokuhne]

i think it is outrageous that they didnt use x(n) for the minimum number of crosses. Absolute tragedy!

[FrankHarwald]

For those who don't know: these graphs that are split in two parts with mutual edges between their vertices but not within same part are called "bipartite graphs".

[gregreynolds]

To anyone who thinks this kind of maths is a bit abstract - I used to use a lot of these graph algorithms in the EDA (electronic design automation) industry - when you get down to really low level problems, this sort of stuff is invaluable and using it is the only way to make many things realistic and/or feasible.

[archivist]

The story of working mathematically in such adverse conditions is inspiring.

[chonkycat]

Three kilns and three storage units is literally the three utilities problem!

[Heshla_Biea]

If a track merger was a viable intersection for this problem, I think you could bring it down a lot by having them all merge down to one and then split back up.

[prosfilaes]

Another obvious solution is to utilize the fact we're working in three dimensions, and have a bridge over the track.

[MathPickle]

There is a mistake in the problem description or a better solution is possible. At 2:39 we see that multiple crossings over the same point are only counted once. At 3:17 we see that curved tracks are possible. Given these two precedents, the partial "optimal" solution at 5:45 can be beaten by (for example) getting rid of intersection #7 by running the 4-5-6 track through intersection #2 instead of creating a new intersection.

[alexgabel]

Can't believe I've been listening to James explain maths curiosities for well over a decade now (since high school until PhD)! And this man seemingly doesn't age. Legend!

[Hambonillo]

The obvious solution is to place each brick onto a blockchain and 3D print it on location.

[michaeldunkerton]

The premise for this one reminds me of Futurama, when Hermes was in a prison camp and optimazed the labor so that it could all be done by one Australian man.

[alecbader]

He looked so happy when he got to name the variables after (k)ilns and (s)torage units

[austynhughes]

Another fantastic Numberphile video. I am so glad I found this channel 6 years ago.

[PunmasterSTP]

You’re kiln-ing me with these extremely fascinating videos!

[Ganerrr]

So there's a (proposed) formula for doing it where all type A must connect to type B, and a general one where all must connect to all, but is there a general one where all type A must connect to type C, all C to type D, etc.?

[-.._.-_...-_.._-..__..._.-.-.-]

Why is it dotted?
The line stands apart,
a whimsical stroke,
a work of art.

Why does my heart
dance for that line,
so delicately dotted,
so mystique and fine.

[AntonAdelson]

Am I the only one who feels that the story of the proofs will even be MORE interesting? Why 6? Why 12? What was the mistake that no one noticed for more than 10 years?

[AlwinMao]

Put all kilns/storage in a circle. Tracks as spokes to the center. Stop all tracks before they overlap. 0 overlaps, but you have a nightmare region in the center where you have to wheel your barrow without a track. Assign a number of forced laborers to the center region to assist.