filmov
tv
What is the Poincare Conjecture?

Показать описание
Is it possible to deduce the shape of the universe without stepping outside of it? Henri Poincaré thought so. Similar to how the Greeks were able to discern the spherical nature of the earth (and even its rough diameter) using mathematics, he proposed that we should be able to make conclusions about our universe. Stanford University mathematician Keith Devlin explains the Poincaré Conjecture, which became a legendary problem in mathematics, with the help of a short animation.
Original Program Date: June 3, 2011
The World Science Festival gathers great minds in science and the arts to produce live and digital content that allows a broad general audience to engage with scientific discoveries. Our mission is to cultivate a general public informed by science, inspired by its wonder, convinced of its value, and prepared to engage with its implications for the future.
Subscribe to our YouTube Channel for all the latest from WSF.
Original Program Date: June 3, 2011
The World Science Festival gathers great minds in science and the arts to produce live and digital content that allows a broad general audience to engage with scientific discoveries. Our mission is to cultivate a general public informed by science, inspired by its wonder, convinced of its value, and prepared to engage with its implications for the future.
Subscribe to our YouTube Channel for all the latest from WSF.
Poincaré Conjecture - Numberphile
Poincare Conjecture and Ricci Flow | A Million Dollar Problem in Topology
What is the Poincare Conjecture?
What is The Poincare Conjecture all about? #shorts
The Poincaré Conjecture - Solving a 100-Year-Old Mystery!
The Poincaré Hypothesis: A Simple Explanation of Perelman's Proof
Grigori Perelman a mathematician who refused to accept a Fields Medal and the $1,000,000 Clay Prize.
The Man Who Solved the $1 Million Math Problem...Then Disappeared
How Grigori Perelman Solved the 100-Year-Old Math Problem and Changed Topology Forever
Deux (deux?) minutes pour la conjecture de Poincaré
Poincaré Conjecture proof overview | Proof by Grigory Perelman and Richard S Hamilton
Grigori Perelman and the Poincare Conjecture | Jordan Ellenberg and Lex Fridman
Die Poincaré-Vermutung | Mathewelten | ARTE
Ricci Flow - Numberphile
Math Genius Solves Million Dollar Problem Then WALKS AWAY
Poincare Conjecture explained | Millennium problems
Millennium Problem-Grigori Perelman #shorts #KnowX
Poincaré Conjecture
GRIGORI PERELMAN the PROVER of the POINCARE CONJECTURE
Grigori Perelman, el genio que resolvió la Conjetura de Poincaré. #shortvideo #grigoriperelman
Grigori Perelman: The Enigmatic Solver of the Poincaré Conjecture
Grigori Perelman: The Reclusive Genius Who Solved the Poincaré Conjecture
Grigori Perelman: The Genius Who Solved a Mathematical Mystery
The Million Dollar Problem that Went Unsolved for a Century - The Poincaré Conjecture
Комментарии
 0:08:52
0:08:52
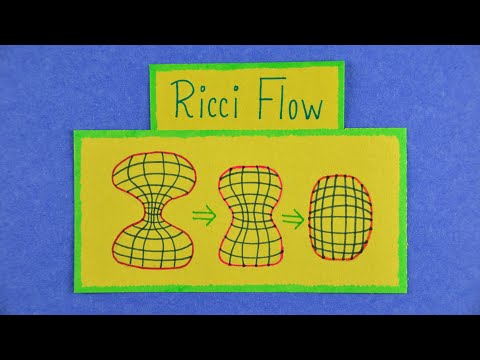 0:08:27
0:08:27
 0:03:27
0:03:27
 0:01:00
0:01:00
 0:01:11
0:01:11
 0:02:28
0:02:28
 0:00:19
0:00:19
 0:10:45
0:10:45
 0:00:49
0:00:49
 0:23:33
0:23:33
 0:03:50
0:03:50
 0:08:56
0:08:56
 0:09:53
0:09:53
 0:14:41
0:14:41
 0:00:55
0:00:55
 0:01:16
0:01:16
 0:00:22
0:00:22
 0:01:19
0:01:19
 0:00:10
0:00:10
 0:00:20
0:00:20
 0:00:13
0:00:13
 0:00:11
0:00:11
 0:00:51
0:00:51
 0:19:25
0:19:25