filmov
tv
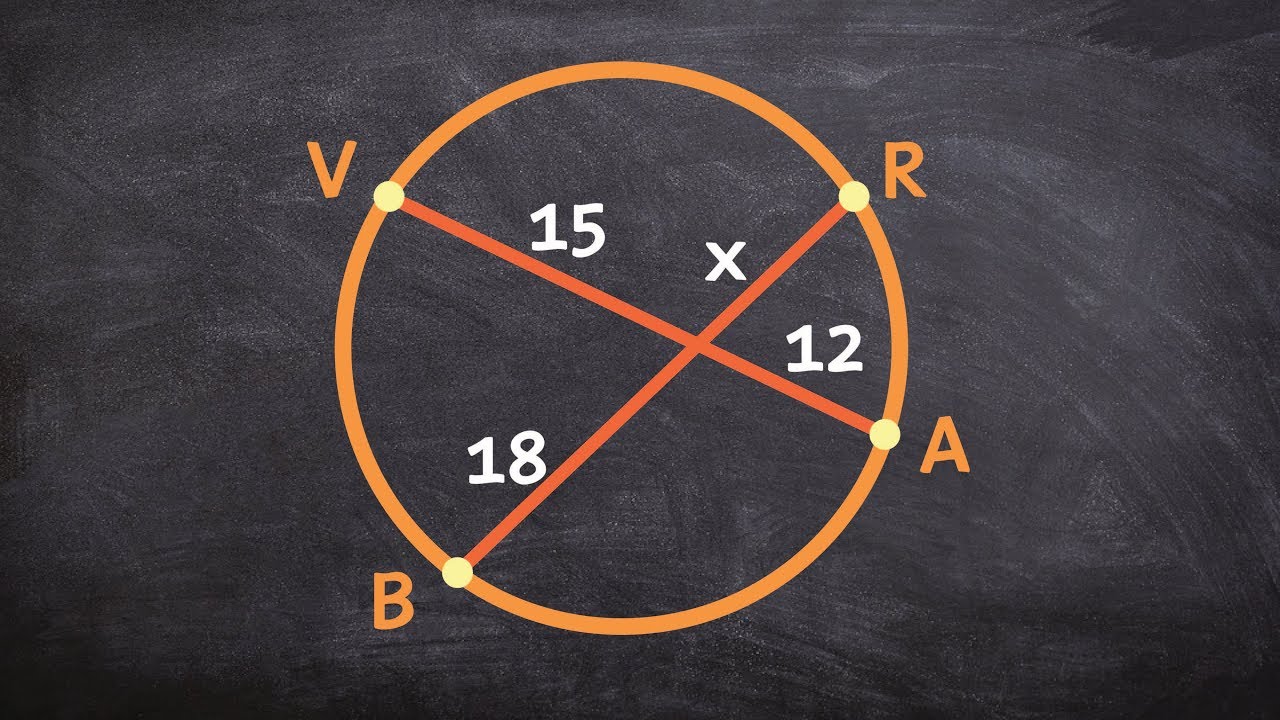
Given the intersection of two chords, find the missing length
Показать описание
Learn how to solve problems with chords. A chord is a line that has its two endpoints on the circle. A chord passing through the center of the circle is refered to as the diameter of the circle. A chord divides the circle into two portions: major and minor segments and also divides the cirumference of the circle into the major and the minor arc.
When two chords intersect inside a circle, the relationship between the two chords is that the product of the lengths from the point of intersection to the two endpoints for one chord is equal to that of the other chord.
#geometry #circles
When two chords intersect inside a circle, the relationship between the two chords is that the product of the lengths from the point of intersection to the two endpoints for one chord is equal to that of the other chord.
#geometry #circles
0:05:23
Finding The Point of Intersection of Two Linear Equations With & Without Graphing
0:02:39
Find the Intersection of Two Line Segments in 2D (Easy Method)
0:07:22
Line of Intersection of Two Planes
0:04:01
How to find the intersection point of two linear equations
0:08:13
Intersection of Two Linked Lists - Leetcode 160 - Python
0:00:48
Given the intersection of two chords, find the missing length
0:04:30
Linear Algebra 2 - 1.5.5 - Basis for an Intersection or a Sum of two Subspaces (Video 1)
0:08:53
How To Find The Intersection and Union of Two Intervals
0:08:16
Finding the intersection of two lines without graphing
0:32:05
L12. Find the intersection point of Y LinkedList
0:05:39
Intersection of Two Planes in a Line Vector
0:05:51
Finding the point of intersection of two lines in vector form.
0:05:42
Vector function for the curve of intersection of two surfaces (KristaKingMath)
0:06:47
How to Find the Intersection Points of Two Circles with Different Centres and Radiuses
0:06:51
Intersection of Two Lines in 3D Space | Intersecting Lines
0:02:00
Find the intersection of two sets
0:03:41
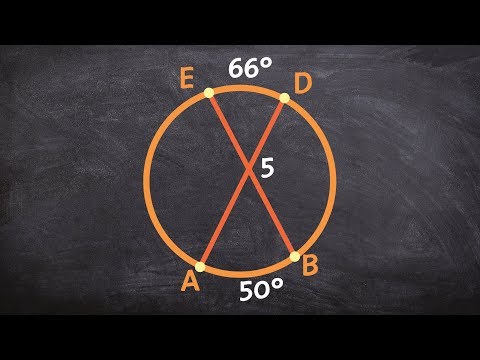
Given the intersection of two chords not at the center find the measure of an arc
0:04:35
How Can We Find The Area of the Intersection of Two Circles? - Solving Problems Together
0:11:58
Vector Equation of Line of Intersection of Two Planes
0:02:25
Finding an Intersection of Two Sets
0:02:40
Solving a system of equations by finding the intersection of two lines
0:12:35
Parametric equations for the line of intersection of two planes (KristaKingMath)
0:04:17
13.1: Another method to parametrize intersection of two surfaces
0:20:49
Intersection of 2 Lines in 3 D Space
Комментарии