filmov
tv
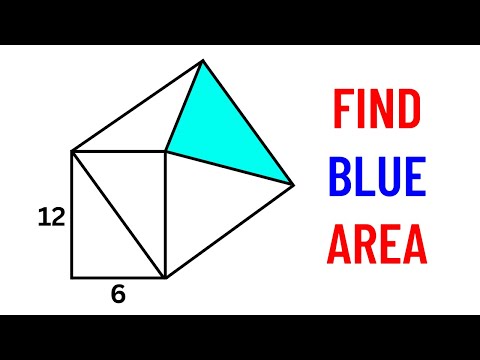
Find the Blue Area
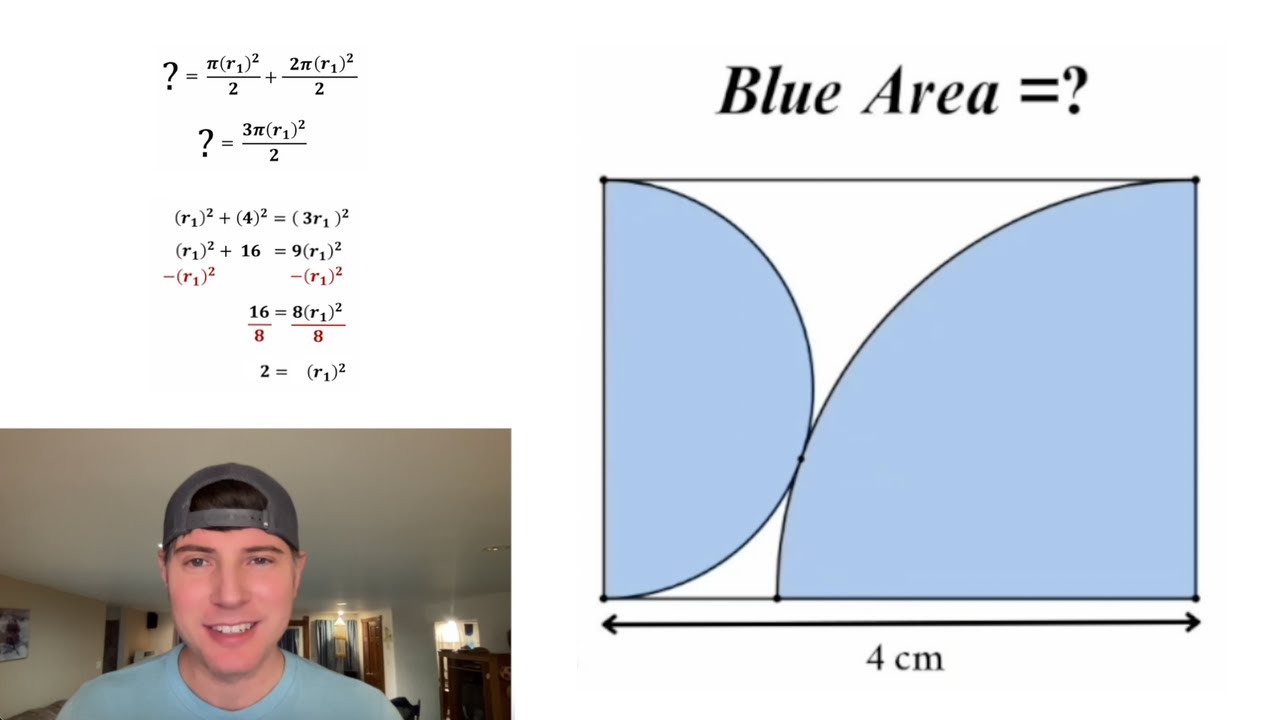
Показать описание
For more geometry challenges check out the above link!
Find the Blue Area
Find Blue Area?
Algebraic Approach to this one
Find the Area of the Blue Region.
How to calculate the Blue Area? | Simple and Easy solution | Semi Circles | Geometry Math Problem
Math Olympiad | Can you find the Blue region area? | (Step-by-step explanation) | #math #maths
Brilliant Blue Area Question
Find The Blue Shaded Area | 3 squares inside a triangle | Important Geometry Skills Explained
Can you solve this puzzle? Find the blue area.
Can you find area of the Blue shaded region? | (Think outside the Box) | #math #maths | #geometry
Find the blue shaded area | Geometry Problem | 2 Different Methods | Very Important Geometry Skills
Find the Area of the Blue Triangle Inside of a Rectangle
Can you find area of the Blue shaded region? | (Semicircle) | #math #maths | #geometry
Geometry Challenge: Find the Area of the Blue Region - 3 Circles inside 1 Big Circle
Find the blue area | Geometry problem | Given a semicircle and a quarter circle inside a rectangle
Can You Find the Blue Area in this Triangle? A Nice Geometry Problem Test Your Math Skills Part 34
Find the blue shaded area | Geometry Problem | 2 Different Methods to Solve
Find the blue shaded area | Given a rectangle and a square | Important Geometry Skills Explained
Find the blue shaded area | Math Olympiad Geometry Problem | Important Geometry and Algebra Skills
Find the Area of the Blue Shaded Region in this Circle | Fast & Easy Explanation
Find area of the Blue shaded region | Two squares | Important Geometry skills explained
A Nice Math Olympiad Geometry Problem | Find the blue area | Important Geometry and Algebra Skills
Can you find area of the Blue shaded region? | (Think outside the Box) | #math #maths | #geometry
Find the Area of the Blue Shaded Region | Step-by-Step Tutorial
Комментарии
 0:02:41
0:02:41
 0:02:49
0:02:49
 0:03:38
0:03:38
 0:07:08
0:07:08
 0:01:54
0:01:54
 0:08:18
0:08:18
 0:03:21
0:03:21
 0:09:10
0:09:10
 0:01:02
0:01:02
 0:05:35
0:05:35
 0:20:10
0:20:10
 0:09:45
0:09:45
 0:08:44
0:08:44
 0:05:53
0:05:53
 0:08:09
0:08:09
 0:10:49
0:10:49
 0:13:55
0:13:55
 0:10:04
0:10:04
 0:22:38
0:22:38
 0:05:42
0:05:42
 0:05:58
0:05:58
 0:15:05
0:15:05
 0:04:50
0:04:50
 0:06:21
0:06:21