Find the blue shaded area | Geometry Problem | 2 Different Methods | Very Important Geometry Skills

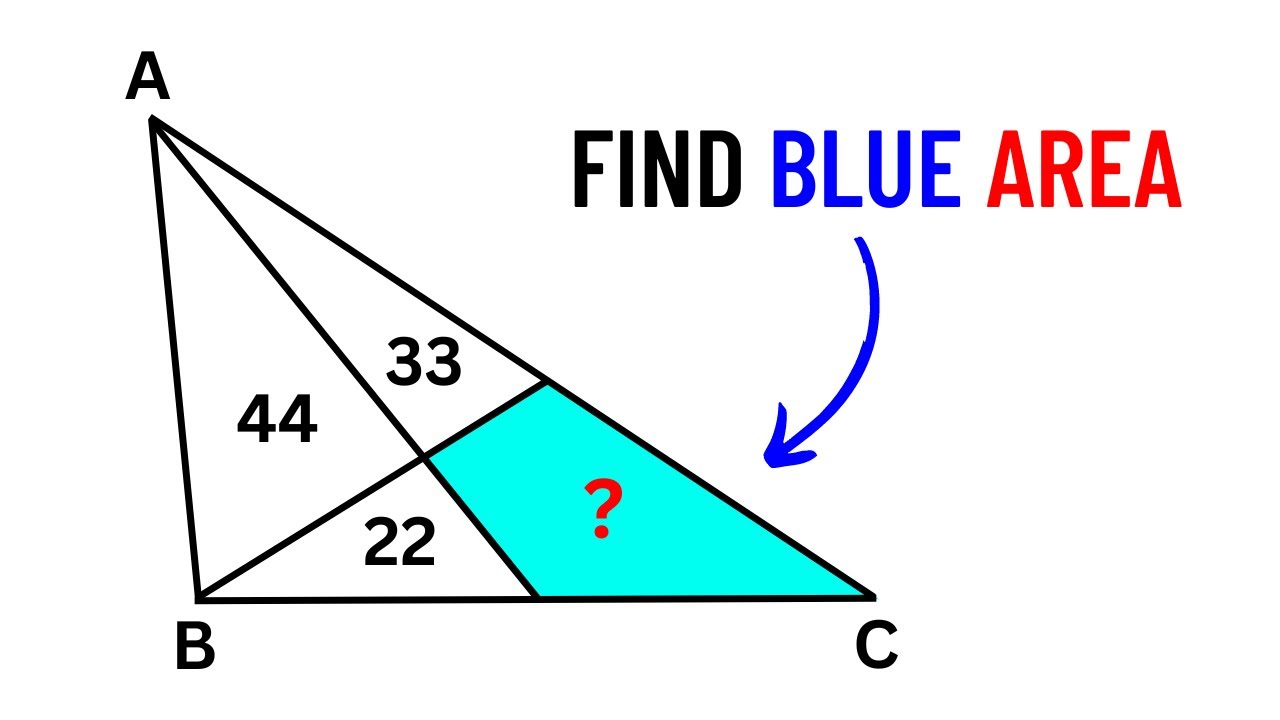
Показать описание

Find the blue shaded area | Geometry Problem | 2 Different Methods | Very Important Geometry Skills

Join this channel to get access to perks:

MEMBERS OF THIS CHANNEL
••••••••••••••••••••••••••••••••••••••••
Mr. Gnome
Sambasivam Sathyamoorthy
ஆத்தங்கரை சண்முகம்
Εκπαιδευτήρια Καντά
AR Knowledge
堤修一
Sidnei Medeiros Vicente
Mark Ludington
Saunak Swar
Grace Cooper
Nicholas Garcia
Ben Stone
Karlo Karbs
Debashis Kesh

Рекомендации по теме

Комментарии

If you rotate this triangle, you can apply the ladder theorem which relates the areas:
1/(AEF+AFB) + 1/(ADB+AFB) = 1/ABC + 1/AFB.
So 1/(33+44) + 1/(22+44) = 1/T + 1/44.
T is the total area of the triangle, and we find T=184.8. The area of CDFE= T-(22+33+44)= 184.8-99=85.8

brandonk

If you know Mass Points, you can solve as follows. Let G be the point where AD and BE meet. Since AG:GF=44:22=2:1, assign weights A=n, D=2n. Similarly assign B=3m, E=4m. The weight of C is 2n-3m along BDC and 4m-n along AEC. Then 2n-3m=4m-n, so 3n=7m. If we set n=7, m=3 then the weights of A and C are 7 and 5. Thus the area of BEC is (7/5)*77, or 107.8. Now subtract 22 to yield the blue area of 85.8.

davidellis

It can be very easily solved by using area lemma and menalaus theorem
Let required area is x
In triangle ABE =>AF :FE= 4:3.
In traingle ABD and DAC=> BD:DC=66:33+x
In triangle ABE and BEC => CE:EA=22+x:77
Using menalaus theorem in triangle BEC, 66/33+x * 99+x/77 * 3/4=1
Which gives x as 85.8 💁👀

SumitVerma-lgqh

Hello, I'm from Brazil and I'm really enjoying your channel. I would like you to prove the following:

(a - 1)^a < a^(a - 1), where "a" is a positive real number greater than 1,

gabrielfreitas

you could just use Menelaus in the ACD triangle

mice

Find the blue shaded area | Geometry Problem | 2 Different Methods | Very Important Geometry Skills

Find the blue shaded area | Geometry Problem | Important Geometry Skills explained

Find the blue shaded area | Geometry Problem | Important Geometry Skills Explained

Find the blue shaded area | Geometry Problem | Important Geometry and Algebra Skills Explained

Geometry Problem: The Value of the Blue Shaded Area?

Find the blue shaded area | Geometry Problem | Important Geometry Skills Explained

Find the blue shaded area of the square | A Nice Geometry Problem

Find the blue shaded area | Given a rectangle and a square | Important Geometry Skills Explained

Find the Area of the Blue Shaded Region | Step-by-Step Tutorial

Find the blue shaded area | Math Olympiad Geometry Problem | Important Geometry and Algebra Skills

Calculate the Blue Shaded Area. Geometry problem/

Find the blue shaded area | Geometry Problem | 2 Different Methods | Very Important Geometry Skills

Find the blue shaded area | Geometry Problem | 2 Different Methods to Solve

Can you find the area of the Blue shaded region? | (Important Geometry skills explained) #math

Find area of blue shaded region in a circle | Chord lengths are 4 and 8 | Important skills explained

Can You Find The Blue Shaded Area | Improve your Geometry Skill

Find The Area of The Blue Shaded Region in The Square | Geometry | Math | 2 Methods

Find The Blue Shaded Area | 3 squares inside a triangle | Important Geometry Skills Explained

Find The Blue Shaded Area in The Quadrilateral | Geometry | Math

Find the blue shaded area | Semicircle inside a quarter circle | Calculus | Area under the curve

Can you find area of the Blue shaded region? | (Think outside the Box) | #math #maths | #geometry

Find the blue shaded area | Geometry Problem | Important Geometry and Algebra Skills Explained

How to Find The Blue Shaded Area Using Euclid Theorem | Improve your Geometry Skill

Find the blue shaded area | Given a semicircle inside a quarter circle | Geometry Problem | 2 Method

Calculate the blue shaded area | Geometry Problem | 2 Different Methods