Before You Start On Quantum Mechanics, Learn This

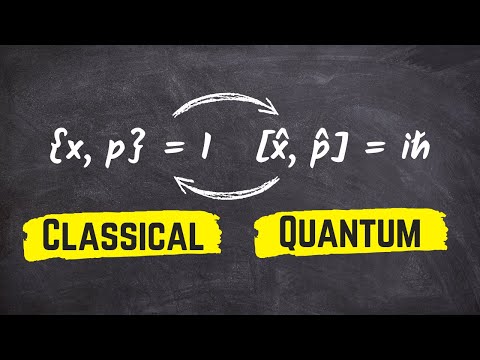
You can't derive quantum mechanics from classical laws like F = ma, but there are close parallels between many classical and quantum equations. Many fundamental quantum equations are expressed as a commutator of operators, such as the canonical commutation relation and the Heisenberg equation of motion. These equations have classical parallels where the quantum commutator is replaced by a classical operation called the Poisson bracket, up to a factor of i hbar. I'll show how Poisson brackets work, and how they mirror these key quantum equations.

About physics mini lessons:
In these intermediate-level physics lessons, I'll try to give you a self-contained introduction to some fascinating physics topics. If you're just getting started on your physics journey, you might not understand every single detail in every video---that's totally fine! What I'm really hoping is that you'll be inspired to go off and keep learning more on your own.

About me:

[pianophiliarmonic]

Your videos are crystal-clear, beautifully laid out and follow a precise progression, treating not so easy topics that are usually not well understood or explained with a lot of confusion.
I think your videos are among the very top quality materials on physics divulgation, and I'm sure that more and more people will join. Keep up the excellent work! <3

[eigenchris]

I remember finding this connection between QM and CM really intriguing when I first learned it, but I'm also a bit sad that I've never learned a deeper reason for why the "replace Poisson brackets with commutators" rule makes sense. Do you know of any deeper algebraic or physical reason why this connection exists?

[bartpastoor]

Again very good. Yes I would appreciate to see Noethers Theorem worked out in Hamiltonian formalism.

[shutupimlearning]

i love how your able to stay focused to the topic at hand to avoiding long tangents. It makes the videos so much easier to digest.

[bartpastoor]

The quality and level of the videos is just too good and provide much insight.
They seduce you to pick up a pen and paper to do the calculations yourself.
I could not resist and am proud to have become one of your patreons just now.
Keep up the good work!

[musamoloi]

This is where the concept of spin (a form of angular momentum) gets weird as it has no classical counterpart that you can relate it to😍. I love physics.

[nagygergely]

As someone who is going to take QM in my next semester, thanks for the help! Thankfully we covered a lot of what you said in CM, but the video is a cool summary and refresher on the topic. Especially necessary when you have so many other subjects too.

[volcanic]

My classical mechanics course glossed over hamiltonian mechanics, but your video was still very clear. Gonna go read up on hamiltonian mechanics now

[WildGamez]

PLEASE EXPLAIN. You have no idea how much researching I have done just to understand quantum mechanics. You are an absolute genius science educator. Keep up the good work man!!!!

[APaleDot]

Wow, criminally underrated. I had to stop and absorb some of the mathematics at times because it moved so fast, but I understood everything.
I really feel like my understanding of math and physics is leveling up.

[erichaag]

Yes, please do the more advanced explanation. If you have any experience with Bessel functions, I would like to see something on that. Really enjoy these videos!

[orangeguy]

From a mathematical perspective the commutator is famous in the context of Lie Algebras, an example of which is first order differential operators. Who knew that math was useful in physics?

[TheFreckCo]

As I'm going through my physics degree, this video is helping me a lot to better understand my classical dynamics course, thank you very much for these videos, I will follow up on your very useful video uploads.

[Eta_Carinae__]

Coming back to this video after learning a little more Hamiltonian mechanics, the Poisson bracket being 1 is the exact same as the condition for a coordinate transform to be canonical - that is, for the Jacobian of the transform M, and the canonical symplectic matrix J (as in, the one that spins everything 90 degrees anticlockwise), the Poisson bracket comes out of the condition that MJM^T = J. And this essentially comes from restricting your coordinate transforms to only the ones where Hamilton's equations of motion are satisfied (i.e. if you have some old coordinates q, p and some new coord.s Q, P, then \del H / \del Q = dP/dt and \del H / \del P = -dQ/dt).

In short: the Poisson bracket is basically the condition for a change of coordinates that allows the same Hamiltonian to describe what's going on in the new phase space.

EDIT: correcting one of the Hamiltonian EoMs.

[mgvlddu]

Ya boi literally explained this in what took two weeks of lectures from my graduate classical mechanics professor. Very nice!

[rlativ]

Definitely interested in the Hamiltonian version of Noether's theorem.

[jaimeduncan]

Men this videos are a jewel. You have a knack for explaining physics and it's clear that you put the effort into understanding the concepts and a lot of effort into this videos. Thanks for sharing. I hope the channel grows.

[vivekpanchal]

This is really amazing,
Watching your videos is really beneficial for students who wants to explore the theories,

Really great work 👏👏🙏🏻

[Mysoi]

Thank you!
very helpful on such an abstract topic.

[lengocchinh]

From Vietnam with love, thank you so much for clear and easy-to-understand video.