filmov
tv
Math 391 Lecture 10 - The Wronskian and what it tells us about solutions to SOLDEs

Показать описание
In this lecture we explore the Wronskain. We motivate its definition and show how it can determine whether two solutions form a fundamental set of solutions to a second order linear differential equation (SOLDE), and in what context it guarantees the existence of a unique solution. We also prove Abel's theorem--a theorem that determines, up to a multiplicative constant, the value of the Wronksian without knowing what the fundamental solutions are.
Math 391 1XC Lecture 10 - Particular Solutions: Variation of Parameters & Undetermined Coefficie...
Math 391 Lecture 10 - The Wronskian and what it tells us about solutions to SOLDEs
Math 391 1XC Lecture 5 - Continuous Compounding; Newton's Cooling Law; Exact Differential Equat...
Math 391 Lecture 11 - Complex roots - Repeated roots - Reduction of order
Math 391 1XC Lecture 4 - Modeling with First Order ODEs: Mixture Problems and Continuous Compounding
Math 391 Lecture 5 - Modeling with linear equations continued - Mixture Problems
Math 391 1XC Lecture 13 - Why are there n solutions to an nth order LODE; More techniques for HOLDEs
Math 391 Lecture 1 - Intro and a very brief (and incomplete) overview of ODEs
Complete Math Revision For SSC CGL 2024 | Top 250 Questions | SSC CGL Maths Previous Year Questions
Math 391 Lecture 23 - Separation of Variables for PDEs and the Heat Equation
Math 391 Lecture 7 - Chapter 2 HW review - part 1
Math 391 1XC Lecture 21 - Final Exam Review
Math 391 1XC Lecture 20 - Sturm-Liouville Theory; The Eigenvalue Problem; and The Heat Equation
Math 391 1XC Lecture 2 - Population Models and First Order Linear Differential Equations
Intro to Continuum Mechanics Lecture 10 | Balance of Linear and Angular Momentum
Math 391 1XC Lecture 19 - Fourier Series
Math 391 Lecture 22 - Fourier Series
Math 391 Lecture 2 - Intro to differential equations - definitions and separation of variables
Math 391 Lecture 15 - Short review of chapter 3 - Test 2 preparation
Math 391 Lecture 8 - Some miscellaneous first order ODEs and Intro to Second order linear ODEs
Math 391 1XC Lecture 18 - The Laplace Transform and using it to solve linear ODEs
Math 391 Lecture 9 - SOLDEs with constant coefficients and the Existence and Uniqueness Theorem
Math 391 Lecture 14 - Mechanical Vibrations - Motion of a mass at the end of a vibrating spring
Math 391 1XC Lecture 3 - Homogeneous First Order Differential Equations
Комментарии
 1:55:46
1:55:46
 0:48:19
0:48:19
 1:38:26
1:38:26
 0:46:47
0:46:47
 1:37:14
1:37:14
 0:52:39
0:52:39
 1:58:02
1:58:02
 0:48:45
0:48:45
 2:53:41
2:53:41
 1:01:53
1:01:53
 0:40:47
0:40:47
 1:51:41
1:51:41
 1:52:46
1:52:46
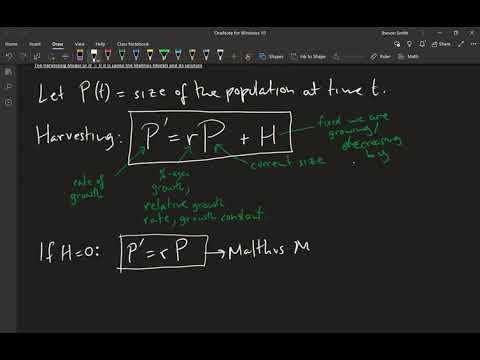 1:37:23
1:37:23
 1:14:18
1:14:18
 1:53:51
1:53:51
 1:07:16
1:07:16
 0:48:22
0:48:22
 0:39:17
0:39:17
 0:32:38
0:32:38
 1:48:54
1:48:54
 0:56:27
0:56:27
 0:59:58
0:59:58
 1:16:41
1:16:41