filmov
tv
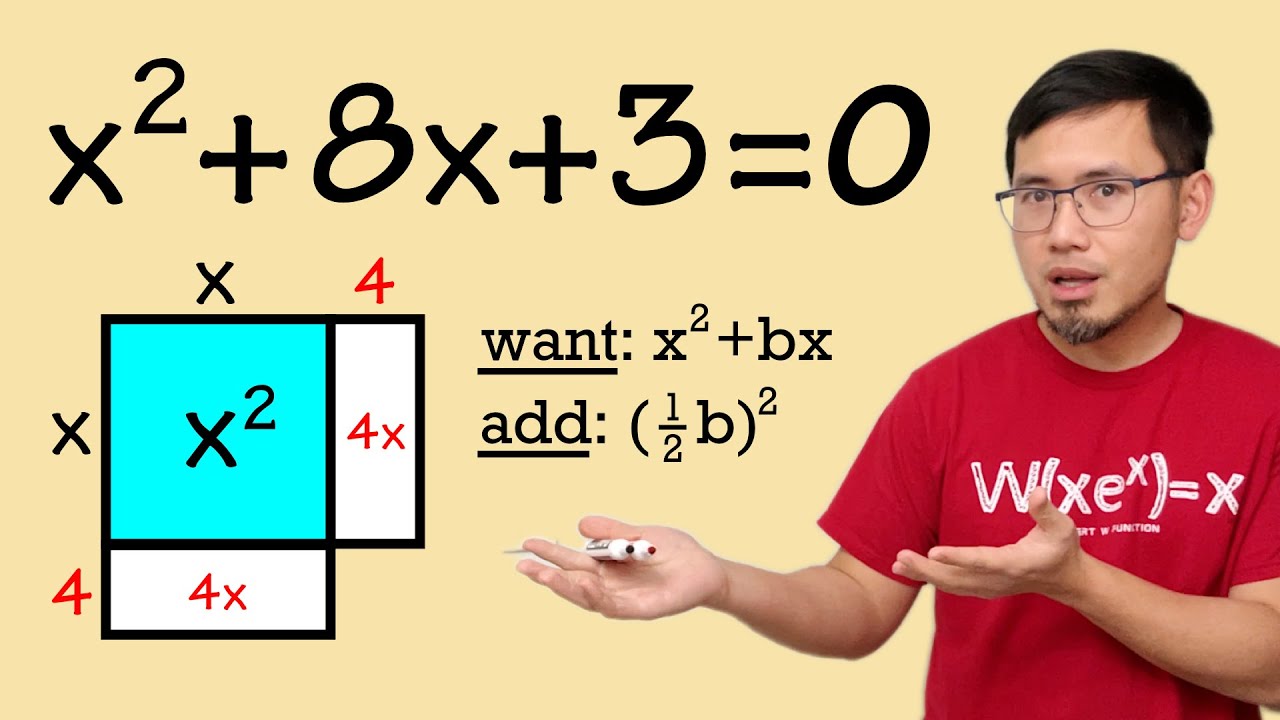
Completing the square (step by step instruction & visual illustration)
Показать описание
-----------------------------
-----------------------------
#math #algebra #mathbasics
Solve by completing the square | Step by Step Technique
Solve by Completing the Square: Step-by-Step Technique
Solving Quadratic Equations By Completing The Square
Completing the Square Visually
Solve quadratic equation by Completing square method
Completing The Square Method | Quadratic Equation | asH maths
GCSE Maths - How to Factorise Quadratics by Completing the Square (Part 1 - Factorise When a=1) #52
Solving a quadratic by completing the square
Visual Reasoning | Part - 2 | Reasoning Topic | CSR Session Day 50
Completing the Square Formula Step-by-Step!
Solve by Completing the Square: Step-by-Step Tutorial
How to Solve By Completing the Square (NancyPi)
How to Solve Quadratic Equations by Completing the Square? Grade 9 Math
Solve Quadratic Equations by Completing the Square: Step-by-Step Tutorial
How to Complete The Square….Step-by-Step….
Solving a quadratic by completing the square
Complete Square Grade 11
Complete the square - FAST and EASY!
How To Prove The Quadratic Formula By Completing The Square
Completing the Square 2 - Corbettmaths
How To Solve Quadratic Equations By Completing The Square
Completing the Square - GCSE Higher Maths
Quick reminder: completing the square
Completing The Square Explained In 1 Minute!
Комментарии
 0:05:20
0:05:20
 0:07:40
0:07:40
 0:07:57
0:07:57
 0:00:54
0:00:54
 0:00:59
0:00:59
 0:00:49
0:00:49
 0:05:14
0:05:14
 0:10:43
0:10:43
 0:30:30
0:30:30
 0:09:58
0:09:58
 0:04:56
0:04:56
 0:17:33
0:17:33
 0:06:32
0:06:32
 0:07:21
0:07:21
 0:17:43
0:17:43
 0:04:10
0:04:10
 0:03:35
0:03:35
 0:04:48
0:04:48
 0:05:41
0:05:41
 0:09:17
0:09:17
 0:13:07
0:13:07
 0:09:46
0:09:46
 0:00:16
0:00:16
 0:00:51
0:00:51