filmov
tv
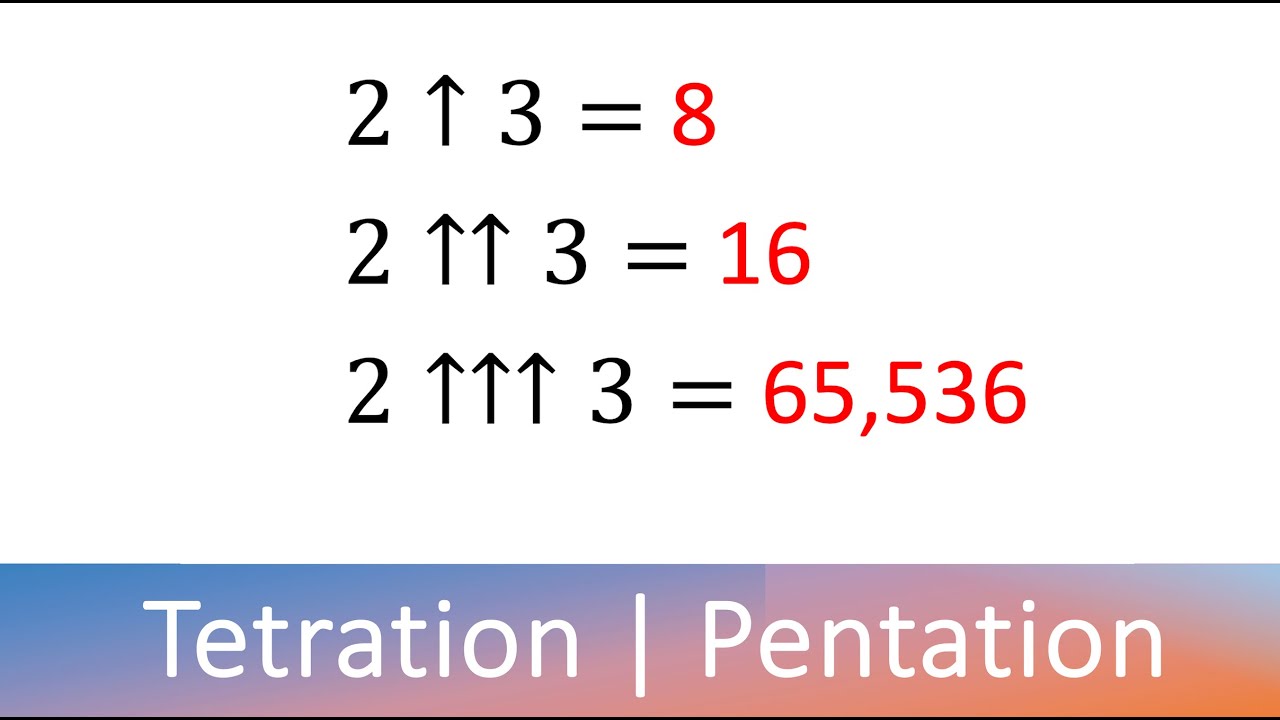
Hyperoperations | Exponentiation | Tetration | Powertowers | Pentation
Показать описание
Hyperoperations | Exponentiation | Tetration | Powertowers | Pentation
The Rare Levels Beyond Exponents
Numbers too big to imagine
Why you didn't learn tetration in school[Tetration]
Have You Heard of Tetration, Pentation, and Hexation? #shorts
What is a pentation? (repeated tetration)
Why use tetration? #shorts
Beyond Exponentiation: A Tetration Investigation
hyperoperation🤯| Pentation | Titration | Exponentiation | Multiplication | Addition | Easy examples...
Hyperoperation Evaluation
1 ^ ∞, It's Not What You Think
Tetration: The operation you were (probably) never taught
Multiplication, Exponentiation, Tetration, Pentation
Tetration, Iterated Exponentiation, Power Towers - the next #math operation
Tetration equation
Addition, Multiplication, Exponentiation... what comes next? (Tetration!)
Exponentiation 2⁴ (2↑4) Vs Tetration ⁴2 (2↑↑4) Vs Pentation (2↑↑↑4) #maths
Hyperoperations and even bigger numbers | Data structures in Mathematics Math Foundations 179
What is Tetration? Going beyond Exponential Growth!
Hyperoperations
WHAT COMES AFTER EXPONENTS? Tetration examples and extensions | ND
Hyperoperation
Luxembourg - Math Olympiad Question | You should know this trick
Graham's Number - Numberphile
Комментарии
 0:02:14
0:02:14
 0:14:39
0:14:39
 0:08:02
0:08:02
 0:06:23
0:06:23
 0:00:27
0:00:27
 0:00:59
0:00:59
 0:00:40
0:00:40
 0:24:46
0:24:46
 0:00:20
0:00:20
 0:00:38
0:00:38
 0:04:28
0:04:28
 0:06:01
0:06:01
 0:00:30
0:00:30
 0:07:05
0:07:05
 0:10:26
0:10:26
 0:17:01
0:17:01
 0:00:16
0:00:16
 0:22:26
0:22:26
 0:02:58
0:02:58
 0:19:56
0:19:56
 0:16:24
0:16:24
 0:08:27
0:08:27
 0:02:51
0:02:51
 0:09:16
0:09:16