filmov
tv
Physics 62 Special Relativity (43 of 43) Relative Velocity - Example 3

Показать описание
In this video I will find the velocity of an object as seen by a stationary observer A.
First video in the Special Relativity series can be seen at:
Physics 62 Special Relativity (30 of 43) The Relativistic Triangle
Physics 62 Special Relativity (18 of 43) A Relativistic Time Experiment
Physics 62 Special Relativity (21 of 43) The Lorentz Transformation Equations: Time
Physics 62 Special Relativity (32 of 43) The Lorentz Factor Close-Up
Physics 62 Special Relativity (19 of 43) A Relativistic Length Experiment
Physics 62 Special Relativity (20 of 43) The Lorentz Transformation Equations: Length
Physics 62 Special Relativity (27 of 43) The Relativistic Units of Energy
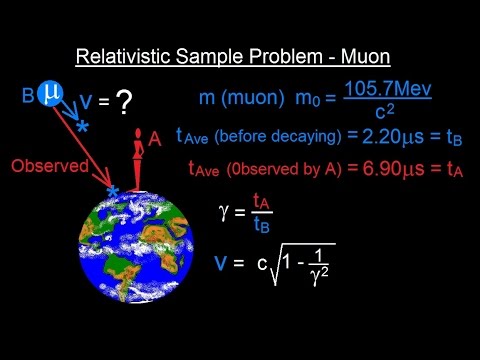
Physics 62 Special Relativity (39 of 43) Relativistic Sample Problem - Muon
Physics 62 Special Relativity (40 of 43) Relativity- Effects of Melting Ice
Physics 62 Special Relativity (17 of 43) Galilean Transformation
Physics 62 Special Relativity (26 of 43) The Relativistic Doppler Effect for Light
Physics 62 Special Relativity (38 of 43) Relativistic Sample Problem - Cosmic Radiation
Physics 62 Special Relativity (12 of 43) Momentum of a Photon
Physics 62 Special Relativity (37 of 43) Relativistic Sample Problem - Velocity
Physics 62 Special Relativity (31 of 43) The Relativistic Triangle Revisited
Physics 62 Special Relativity (35 of 43) Relativistic Sample Problem - Time
Physics 62 Special Relativity (23 of 43) The Lorentz Transformation Equations: Velocity (v')
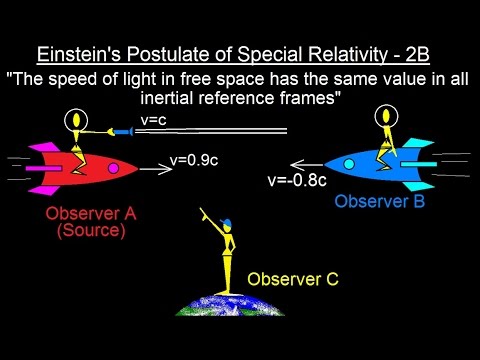
Physics 62 Special Relativity (15 of 43) Einstein's Postulate of Special Relativity - 2B
Physics 62 Special Relativity (24 of 43) The Lorentz Transformation Equations: Velocity (v)
Physics 62 Special Relativity (34 of 43) Relativistic Sample Problem - Length
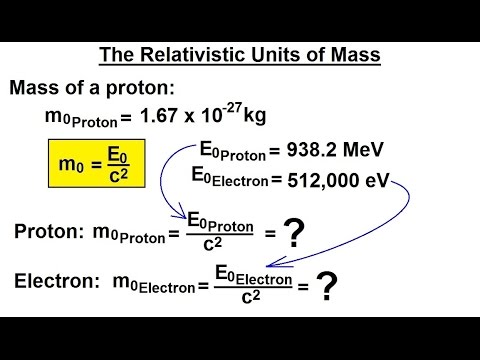
Physics 62 Special Relativity (28 of 43) The Relativistic Units of Mass
Physics 62 Special Relativity (33 of 43) The Lorentz Factor Close-Up 2
Physics 62 Special Relativity (29 of 43) The Relativistic Units of Momentum
Physics 62 Special Relativity (43 of 43) Relative Velocity - Example 3
Комментарии
 0:07:34
0:07:34
 0:11:29
0:11:29
 0:07:00
0:07:00
 0:07:19
0:07:19
 0:15:32
0:15:32
 0:03:23
0:03:23
 0:08:25
0:08:25
 0:03:22
0:03:22
 0:04:10
0:04:10
 0:06:20
0:06:20
 0:09:16
0:09:16
 0:11:28
0:11:28
 0:06:34
0:06:34
 0:05:10
0:05:10
 0:02:25
0:02:25
 0:07:52
0:07:52
 0:04:21
0:04:21
 0:04:12
0:04:12
 0:03:12
0:03:12
 0:07:45
0:07:45
 0:03:36
0:03:36
 0:04:47
0:04:47
 0:07:17
0:07:17
 0:03:08
0:03:08