filmov
tv
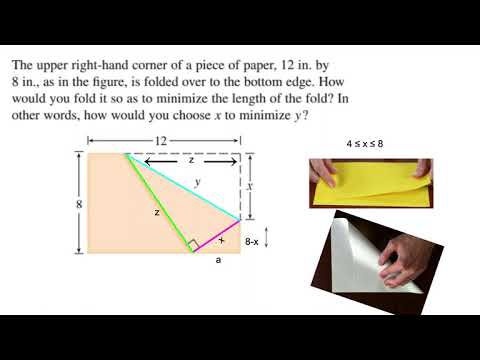
Calculus Minimum Edge Length of Paper Fold from a Corner

Показать описание
Optimization of Time Application: Find the critical numbers and analyses for optimum value
A unique series developed for the students preparing for GCSE Level A and equivalent examination globally. Anil Kumar has shared his knowledge with students who are preparing for GCSE Level A so that they can understand and perform much better.
Absolute Maximum and Absolute minimum value for any function continuous in closed interval [a, b] will always exist at the critical numbers or at the end points.
#optimization_Calculus #Increasing_Decreasing_Interval #IBSL_Calculus #IBSL_exponential_derivatives #Higher_Mathematics_Differentiation #anilkumarmath #globalmathinstitute #mcv4u
A unique series developed for the students preparing for GCSE Level A and equivalent examination globally. Anil Kumar has shared his knowledge with students who are preparing for GCSE Level A so that they can understand and perform much better.
Absolute Maximum and Absolute minimum value for any function continuous in closed interval [a, b] will always exist at the critical numbers or at the end points.
#optimization_Calculus #Increasing_Decreasing_Interval #IBSL_Calculus #IBSL_exponential_derivatives #Higher_Mathematics_Differentiation #anilkumarmath #globalmathinstitute #mcv4u
Calculus Minimum Edge Length of Paper Fold from a Corner
Calculus | Minimum Length of Crease | Revised
Optimization Problem: Minimize the printing area of the poster
Calculus | Minimum Length of the Crease| Raysurian Method
Calculus | Minimum of Crease | Ratio Method
Related Rate Problems - The Cube - Volume, Surface Area & Diagonal Length
Calculus | Minimum of Crease | Area Method
Calculus | Minimum of Crease | Trigonometric Method
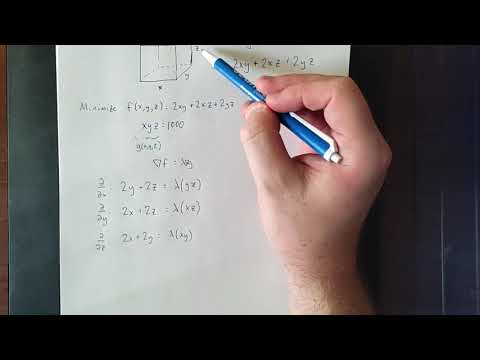
Lagrange Multipliers, example 3
Solving Maximum/Minimum Problems Using Calculus
Calculus | Minimum of Crease |Calculate the Derivative
Maximum edge length of a cuboid.【Fun Math, 9th grade – 11】
Absolute Maximum and Minimum Values of Multivariable Functions - Calculus 3
Optimization Question - Corner Cut to form a Box
Calculus - Minimum Surface Area of an Open Cylindrical Can
Optimization Problem
Calculus Optimization Problem 04
Maximize Volume of an Open Top Box (Optimization) | Calculus 1 Exercises
Calculus | Minimum of Crease | Pythagorean Method
Calculus - Another Applied Max/Min Example
Optimization Problems | Calculus Lesson 27 - JK Math
Salsa Night in IIT Bombay #shorts #salsa #dance #iit #iitbombay #motivation #trending #viral #jee
Find the maximum area enclosed by 80 m fence with one side wall
How to eat Roti #SSB #SSB Preparation #Defence #Army #Best Defence Academy #OLQ
Комментарии
 0:15:04
0:15:04
 0:19:15
0:19:15
 0:05:53
0:05:53
 0:09:44
0:09:44
 0:11:46
0:11:46
 0:12:23
0:12:23
 0:12:34
0:12:34
 0:09:13
0:09:13
 0:08:49
0:08:49
 0:05:02
0:05:02
 0:09:02
0:09:02
 0:03:32
0:03:32
 0:11:24
0:11:24
 0:11:12
0:11:12
 0:07:21
0:07:21
 0:14:56
0:14:56
 0:07:33
0:07:33
 0:10:33
0:10:33
 0:11:05
0:11:05
 0:09:15
0:09:15
 0:19:49
0:19:49
 0:00:14
0:00:14
 0:03:51
0:03:51
 0:00:16
0:00:16