Related Rate Problems - The Cube - Volume, Surface Area & Diagonal Length

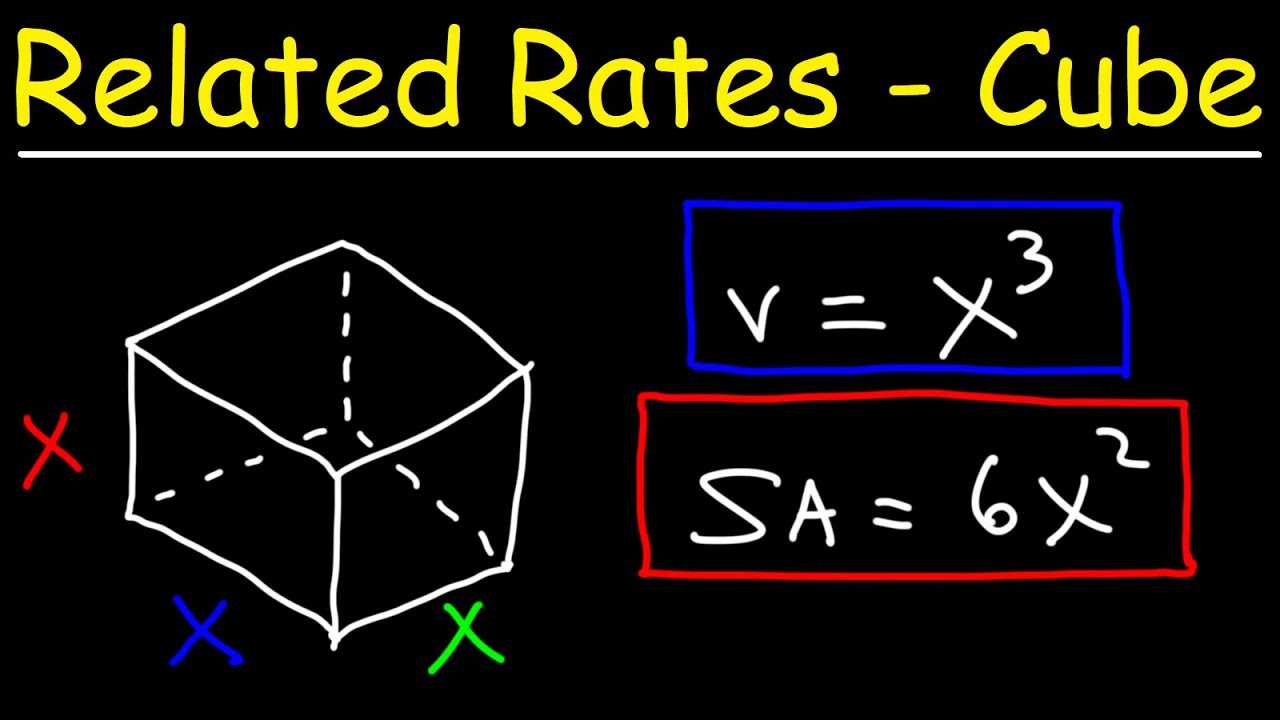
This calculus video tutorial explains how to solve related rate problems with the cube. It explains how to find the rate at which the volume of a cube is changing with respect to the time. Other topics include the surface area of a cube as well as the diagonal length of a cube.

Introduction to Limits:

Derivatives - Fast Review:

Introduction to Related Rates:

Derivative Notations:

Related Rates - The Cube:

__________________________________
Inflated Balloon & Melting Snowball:

Gravel Dumped Into Conical Tank:

Related Rates - Area of a Triangle:

Related Rates - The Ladder Problem:

Related Rates - The Distance Problem:

____________________________________
Related Rates - Airplane Problems:

Related Rates - The Shadow Problem:

Related Rates - The Baseball Diamond Problem:

Related Rates - The Angle of Elevation Problem:

Related Rates - More Practice Problems:

_________________________________
Final Exams and Video Playlists:

Full-Length Videos and Worksheets:

[georgesadler]

MR. Organic Chemistry Tutor, thank you for an incredible video/lecture on Related Rates problems in Calculus. Related Rates problems can be highly problematic. This is an error free video/lecture on YouTube TV with the Organic Chemistry Tutor.

[nickwright]

“I know my drawings aren’t that great….” Proceeds to draw a badass cube 😭

[xHannaHx]

This is so beautifully explained 😍 thank u!

[a.pd.p]

Can we just use the diagonal formula that is root 3 times x?

[elisaguazon]

In the last problem would it be easier to relate using the formula for the diagonal of a cube D= 3^1/2(a) ? Many thanks

[zubair]

how are you supposed to just remember 9:00 where the two triangles come out of know where

[mrsnowflake]

How do we find each side if we only have a volume? E.g: Cube of a volume of 20cm(cubed), How long is each side of the cube

[chriswilliams]

For part c since the question asks "how fast is the length of the diagonal" how do we know to solve for z and not l when they are both a diagonal in the cube? Is it because z covers all the entire edge lengths?

[yeakin]

I know my drawings is not that great...who cares? You literally know undergrad physics, economics, maths, chemistry and what not.

[twa]

how do you find the rate of change of the edges given the rate of change of the volume

[lovaf]

ok but why in the part c, he didn't write z=x(root)3 instead of z^2=3x^2, wouldn't it make more sense?

[marklenardraz]

I have a question about the surface area, what is the difference of it compared to totasurface area?

[WhateverIwannaupload]

Wtf am I watching? I don't get it. You start at 5x5x5= 125m^3, you add 1000m^3/hr. What does the initial value of x in x^3 matter? What are we even looking for if the problem is saying that the rate of change is 10m/hr and the rate of volume change is 1000m^3??