Optimization Problems | Calculus Lesson 27 - JK Math

How to Solve Optimization Problems (Calculus Lesson 27)

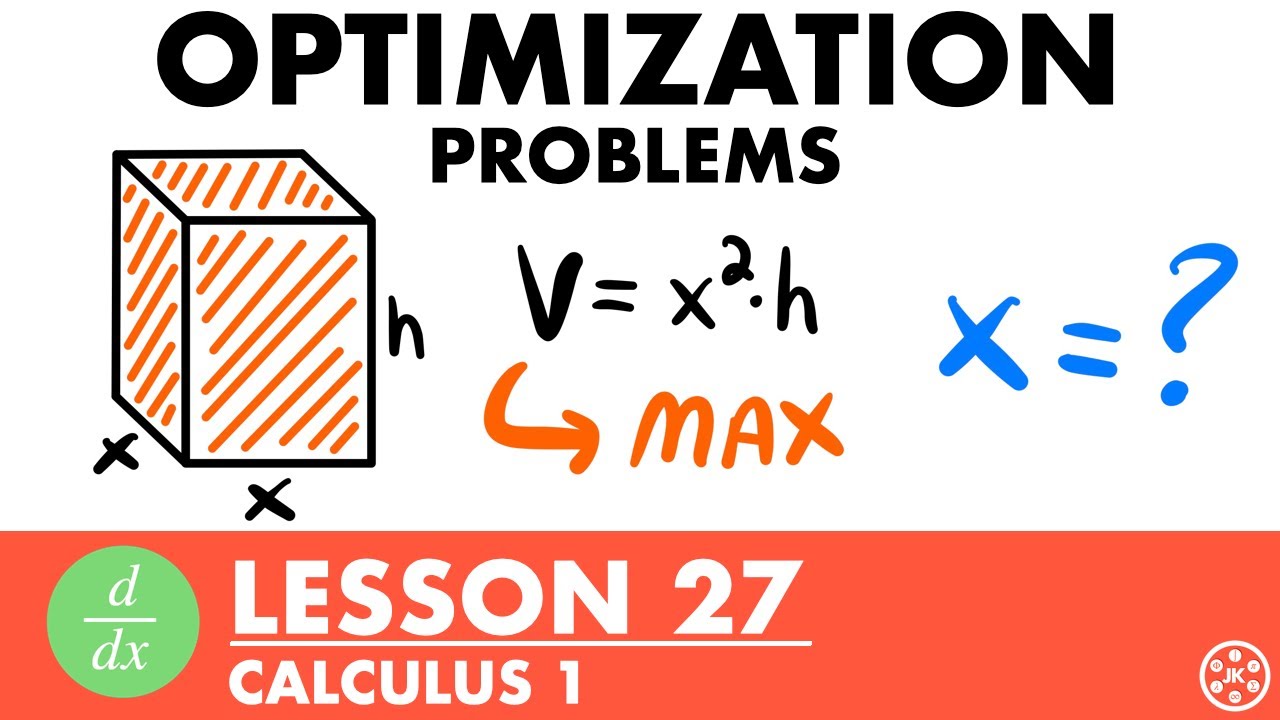
In this lesson we learn how to solve optimization problems. Optimization problems involve the process of either maximizing or minimizing a particular value that may be of interest in a real-world application. This includes the concepts of greatest volume, the least amount of area, etc. We look at the general guidelines to solving optimization problems and then go through various examples.

This course is designed to help students understand the concepts of calculus at a grounded level. No long, boring, and unnecessary explanations, just what you need to know at a reasonable and digestible pace, with each lesson under 20 minutes!

Calculus requires a solid understanding of precalculus and algebra concepts and techniques including factoring, equation manipulation, trigonometric equations, logarithms, finding slope, graphing, and many more. If you are not familiar with these prerequisite topics, be sure to learn them first!

Video Chapters:
0:00 What is an Optimization Problem?
0:56 Example - Maximum Product of Two Numbers
6:10 Example - Minimum Perimeter
12:33 Guidelines to Solving Optimization Problems
12:51 Example - Maximum Volume

⚡️Math Products I Recommend⚡️

⚡️Textbooks I Use⚡️

⚡️My Recording Equipment⚡️

(Commissions earned on qualifying purchases)

Find me on social media:
Instagram: @jk_mathematics

Found this video to be helpful? Consider giving this video a like and subscribing to the channel!

Thanks for watching! Any questions? Feedback? Leave a comment!

-Josh from JK Math

#calculus

Disclaimer: Please note that some of the links associated with the videos on my channel may generate affiliate commissions on my behalf. As an amazon associate, I earn from qualifying purchases that you may make through such affiliate links.

[aniketgharpure]

If we set the derivative equal to 0, we can either get a maxima or a minima. How do we know whether the answer we get from that step is a max or a min? Do we need an additional step of finding a second derivative to be sure?