Maximize Volume of an Open Top Box (Optimization) | Calculus 1 Exercises

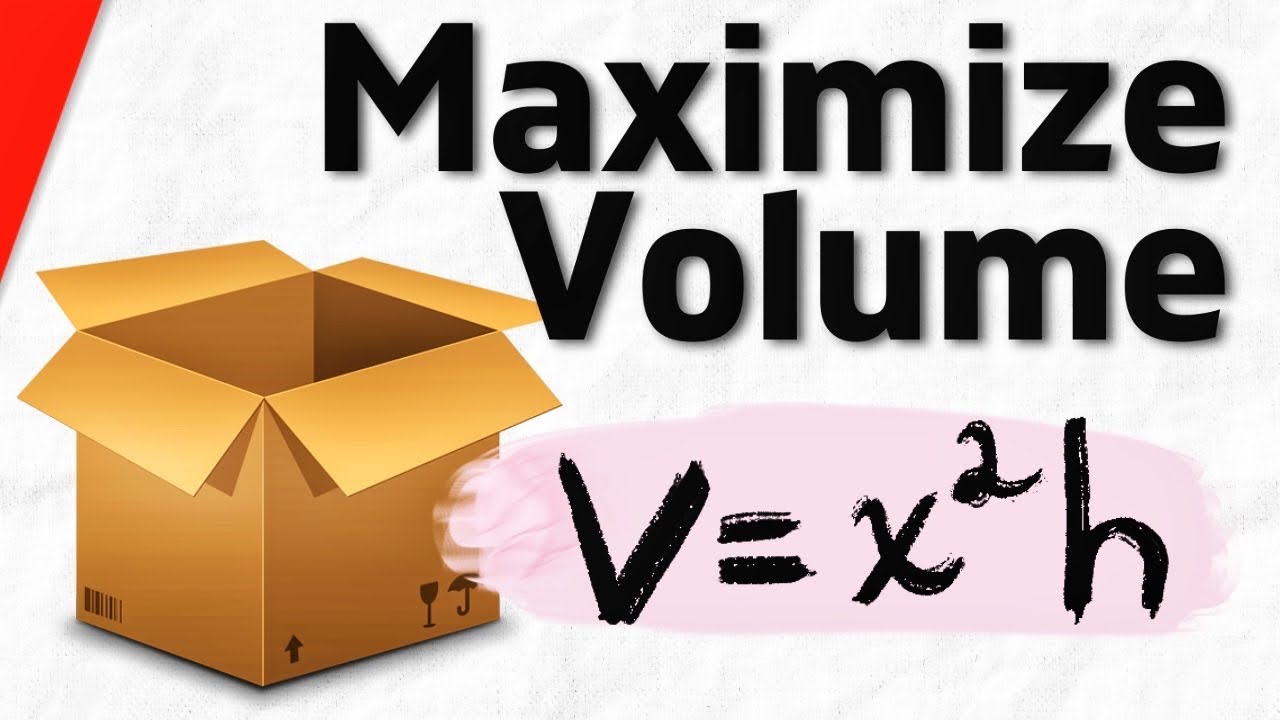
preview_player
Показать описание
We solve a common type of optimization problem where we are asked to find the dimensions that maximize the volume of an open top box with a square base and a fixed surface area. To do this we begin with a volume equation then use the surface area restriction to rewrite the volume in terms of a single variable, at which point we can use our usual strategy of taking the first derivative, finding critical points, then using the first or second derivative test to classify these critical points. #Calculus1 #apcalculus

◉Textbooks I Like◉

★DONATE★

Thanks to Petar, dric, Rolf Waefler, Robert Rennie, Barbara Sharrock, Joshua Gray, Karl Kristiansen, Katy, Mohamad Nossier, and Shadow Master for their generous support on Patreon!

Follow Wrath of Math on...

  1. Wrath of Math
  2. wrath of math
  3. math lessons
  4. math
  5. education
  6. math video
Рекомендации по теме
Maximize Volume of 0:10:33

Maximize Volume of an Open Top Box (Optimization) | Calculus 1 Exercises

Volume of Open 0:05:47

Volume of Open Box Made From Rectangle with Squares Cut Out

Determine the Maximum 0:07:32

Determine the Maximum Volume of an Open Top Box Using a Graph Only

Optimization - Open 0:11:44

Optimization - Open Box With Max Volume | JK Math

Ex 2: Max 0:07:15

Ex 2: Max / Min Application Problem - Max Volume of a Box

Optimisation Grade 12: 0:05:34

Optimisation Grade 12: Maximum Volume Box

Calculus optimization: given 0:06:18

Calculus optimization: given surface area, want largest volume of an open-top box

Optimization: Minimize Surface 0:06:46

Optimization: Minimize Surface Area of a Box Given the Volume

Volume of Open 0:10:04

Volume of Open Box from Cardboard by Cutting Corners Problem Solving Skill Pre Calculus

Optimization: Find Largest 0:08:05

Optimization: Find Largest Volume of a Box Given Amount of Material

Optimization: Determine the 0:07:38

Optimization: Determine the Maximum Volume of an Open Top Box with a Fixed Surface Area

Stationary Points - 0:15:53

Stationary Points - Maximum Volume of a Box : ExamSolutions Maths Revision

Lagrange Multipliers Maximize 0:12:33

Lagrange Multipliers Maximize Volume of a Box Without Lid

Optimisation Grade 12: 0:03:33

Optimisation Grade 12: Maximum Volume Cylinder

Calc Class: Minimize 0:41:07

Calc Class: Minimize Surface Area Given Volume, Maximize Volume Given Surface Area (Box & Cylind...

Calculus 1: Max-Min 0:06:20

Calculus 1: Max-Min Problems (19 of 30) Maximum Volume of a Closed Box

Find the largest 0:07:14

Find the largest possible volume of box w open top and square base given surface area Optimization

Open-top Box of 0:05:35

Open-top Box of Greatest Volume | Calculus Optimization Problem

101 Maximum Volume 0:10:02

101 Maximum Volume of Cylinder with Surface Area 384 pi Derivatives Calculus

Redmi Phone Automatic 0:02:37

Redmi Phone Automatic Volume UP and DOWN Problem Solved | MIUI BUG | How to solve Volume Bug Redmi

AN OPEN BOX 0:11:47

AN OPEN BOX OF MAXIMUM VOLUME IS TO BE MADE FROM A SQUARE PIECE OF MATERIAL 24CM A SIDE | Harmtedy

Galaxy S22/S22+/Ultra: How 0:01:59

Galaxy S22/S22+/Ultra: How to Set/Remove a Volume Limit Pin

How to increase 0:00:52

How to increase the volume on your iPhone 13 Pro max

Xiaomi/Redmi Phone Automatic 0:03:05

Xiaomi/Redmi Phone Automatic Volume up and down Problem Solved | Fix MIUI Volume Bug

Комментарии
Автор

Follow along with my AP Calculus FRQ Advent Calendar!

WrathofMath
Автор

The way you explained how to solve this is seriously awesome. I was struggling with this type of problem and your teaching made it a cake walk. Thanks

SilentPenguin
Автор

Thank you, very helpful. And having Nicolas Cage do do your voice over was cool.

leighahrens
Автор

If the box were closed the dimensions would be 3sqrt2 in. X3sqrt2in.X3sqrt2in. In addition to working out the math the same way, this makes sense because a perfect cube has the maximum volume for a rectangular box with all closed sides.

larry
Автор

Keep it up man.... excellent work 👌🏻👌🏻

SaurabhMishra
Автор

very helpful, having a AP calc test coming up soon

ooh_netiyiy
Автор

Interesting fact: the volume is 108 in cu. In. Just like the surface area is 108 sq. In.

larry
Автор

Would the surface area equation not be = to 108=x^2 +5xh due to there being a 5th square face on the bottom of the cube ?

christaylor
Автор

can you do the harder extension to this problem where we're not guaranteed that the base is square? so "maximize the volume of a rectangular-based open-top box". I think the proof that the base is square is intuitive, but could you do it set up like a calculus problem with max (xyh) w.r.t. x, y holding h constant?

anthonychuah