Convolution and the Fourier Transform explained visually

Convolution and the Fourier Transform go hand in hand. The Fourier Transform uses convolution to convert a signal from the time domain into the frequency domain. In this video I demonstrate an intuitive way of understanding what convolution is, explain the convolution equation and demonstrate how it is used in the Fourier Transform.

0:00 - Introduction
0:17 - A visual example of convolution
0:52 - Ident
0:57 - Welcome
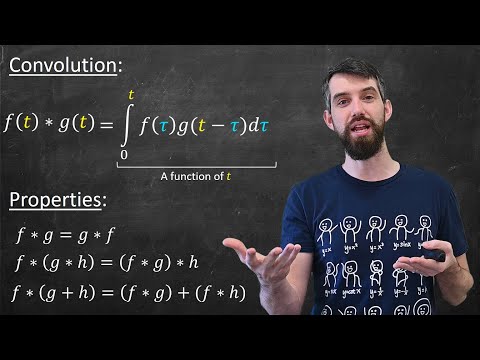
1:19 - The formal definition of convolution
2:24 - The signal being analyzed
2:36 - The test wave
3:00 - The independent variable
3:31 - Stage 1: Sliding the test wave over the signal
4:34 - Stage 2: Multiplying the signals by the test wave
4:51 - Stage 3: Integration (finding the area under the graph)
5:31 - Why convolution is used in the Fourier Transform
7:28 - Challenge

Other works used in this video:
2 Crowd Green Screen and Crowd Talking Sounds
by Creative Film

[MarkNewmanEducation]

At 3:58, for anyone who would like to know why, in general convolution, g(τ) has to be reversed so that it becomes g(-τ), it is because, if it isn't, then the response comes out backwards. For the Fourier Transform, however, as I mention in the video reversing g(τ) when it is a sinusoid has no effect as sinusoids are symmetrical.

[dddderek]

Blew my mind! I left a huge reply on your next video that isn't even out yet!!! This is the teaching I've been waiting my entire life for!!! Thank you so much!!! Love the graphics, too. Boy, convolving the image of yourself with yourself, what a great visual example!!!

[jimmea]

mark is the electrical engineering professor we wish all the rest of them could be

[НиколайАлексапольский]

Это лучшее объяснение свёртки, что я видел. Спасибо!

[arjungoud]

Thank a lot, not been this clear with other videos.

[pradyumnanimbkar]

Mate, how do I learn how to do physics animation and make graphs such as yourself?

[littleKingSolomon]

My search for intuition behind convolution comes to an end with Mark Newman being the game changer. Thanks a ton Mark. Liked and subbed.

[ercocoaz]

What a great explanation! I'm not coming out of college blind after all.

[seahawkers]

Your explanation is a work of art. I could cry. :)

[mutalasuragemohammed]

😅my God! you made me laugh with the bang with which the formula dropped... It's been our nightmare in undergraduate study. Thank you for the succinct explanation.

[rpshukla]

Nice Sir my self Dr RP shukla from India

[OurgasmComrade]

Could you also do a video about the Laplace transform and complex frequency domains? (Including 3D representation of frequency response and how it's affected by poles/zeroes of filters)

[harryhirsch]

Sinusoids are not symmetrical. sin(-tau)=-sin(tau). There are several other problems with this exposition and it intermingles cause and effect.

[SAJAN_ECE]

Actually we are checking the similarity between two signals here. That is called Correlation right? I am confused. Which one are we doing in Fourier Transform? Correlation or Convolution? Please clarify my doubt.

[johana]

Is it possible to get the fourier transform of a sound signal by just using the formulas?

[dancxjo]

I've been looking for this video for 20 years! Acoustics makes so much more sense now. Thanks for explaining the magic!!

[teddyspaw]

Brilliant! Thank you for producing an excellent visual presentation and explanation. I really like the "score" concept!

[ANJA-mjto]

Showing presentation quite different - diverse illustrated than others in the a Fourier transform in case of useful properties as signal is brilliant idea to this important concept that in practical phyhisics can be given by an example like imaging a perfect spectometar and so on represent the Spectral Power Density.
Thanks for fluorescent presentation 👍 and brilliant input to the Convolution Theorem

[keylanoslokj]

Can you please do a comparison between AM and FM modulations?

[fardinfahim]

this has to be the clearest explanation of what convolution is..