Introducing Convolutions: Intuition + Convolution Theorem

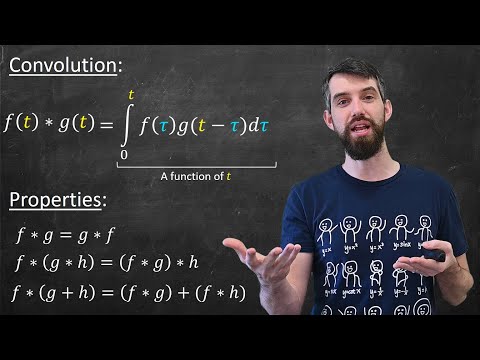
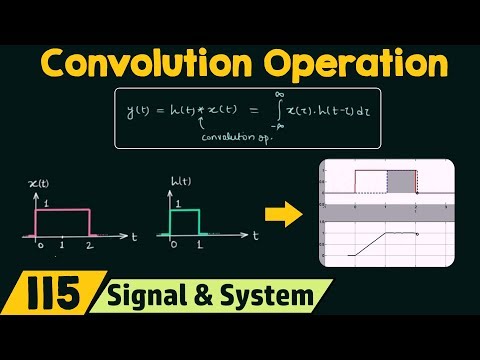
In this lesson, I introduce the convolution integral. I begin by providing intuition behind the convolution integral as a measure of the degree to which two functions overlap while one sweeps across the other. I demonstrate this intuition by showing that the convolution of two box functions is a triangle.

I then move on to proving the Convolution Theorem for Fourier Transforms, and discussing how it compares to the Convolution Theorem for Laplace Transforms. The proof for Fourier Transforms is relatively simple, but the proof for Laplace Transforms is a bit more difficult (if you really want to see the Laplace Transform proof, I can make another video but I've put it off for now).

Questions/requests? Let me know in the comments! Hopefully the intuition I provided was sufficiently clear.

Special thanks to my Patrons for supporting me at the $5 level or higher:
- Jose Lockhart
- Yuan Gao
- Justin Hill
- Marcin Maciejewski
- Jacob Soares
- Yenyo Pal
- Chi
- Lisa Bouchard

[dipankerbaral]

i hereby declare this underrated video the best explanation of convolution in the internet

[riyabansal]

Oh my god, I have been trying to gain an intuition on this topic for so long. So glad I ran into this video! Thank you, sir.

[rockspoon]

I've been through all four calc courses and am on Linear Circuits 2, and this is the first time anyone's written the first part of the definition.

And it makes sense now.

[gutzimmumdo]

finally someone explains concisely what that fucking -t means for fuck sakes, thank you alot best explanation of convolution on the internet.

[pratikahir]

Please continue to make such videos, it serves as a quick refresher for me before exam(VERY HELPFUL).

[duckymomo]

LOL “I hope that the explanation wasn’t too convoluted, haha”

[thevoidzzz]

This is such a MONUMENTALLY important idea in electrical engineering, I don't understand why so many other videos and teachers are so bad at explaining this topic

[davidarredondo]

"I won't be spending the next 18 minutes taking the convolution of sin and cosine in an effort to show you that the convolution of two functions is an actual quantity."

Savage.

I agree though--that Kahn academy video was a waste of time

[simrannahar]

this was extraordinarily well explained

[shine_at_dusk]

Thank you very much) The explanation is so clear I've watched only for about 2 minutes and already got the idea behind the use case

[khaledtaleb]

Thanks god that you made me saw this video in the first month of the semester

[pramod]

Thanks for the explanation... Atlast got a clear visualisation on this topic

[djtoddles]

this is great, please make more of these intuition vids

[rogerz]

Thank you! The concept of convolution is concisely presented.

[leiyplane]

Boundary condition between negative side and positive side can use Laplace Transform too. Fourier Transform is just a special version of Laplace Transform.

[Dontonethefirst]

Getting me through signals and systems man.

[areaxi]

You're actually sweeping across values of tau not t. t is a constant inside the integrand and that is why integrating results in a function of t, y(t).

[LoganStVrain]

Could you do a video about the Fourier transform (definition, purpose and derivation). Also, what is the difference between a Fourier Transform and a Fourier Series. Thanks!

[slashpl]

Awesome, that's what I was looking for

[coz_outline]

The "Ha Ha" in 6:30, lol
Thanks anyway