filmov
tv
Two Methods! Can You Find the Area of the Green Circle? | 2 Easy Methods
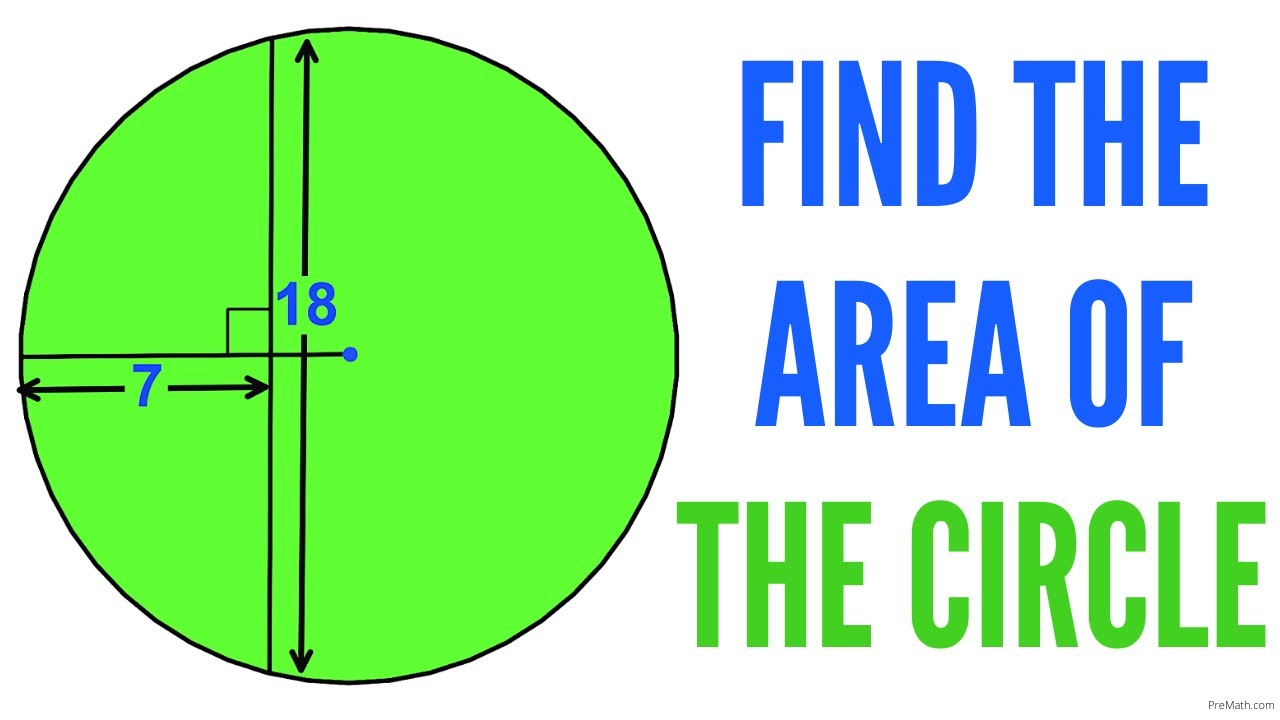
Показать описание
Two Methods! Can You Find the Area of the Green Circle? | 2 Easy Methods
2 + 2 = 5 || Proven with two methods || Can you find the mistake ? 2+2=5 two plus two equals five
2+2=5 || Proven with two methods || Can you find the mistake?2+2=5 two plus two equal 5
How Many Methods Can You Find?
Can you find area of the Yellow shaded Trapezoid? | (Two Methods) | #math #maths | #geometry
Can you find the angle X? | Two Methods | (Step-by-step explanation) | #math #maths #geometry
Can you find the length AB? | (Two Methods) | #math #maths #geometry
The backup boys are starting to go into battle 1259
3+3=7 || Proved with two methods || Can you find the mistake ? Prove 3+3=7 How? -
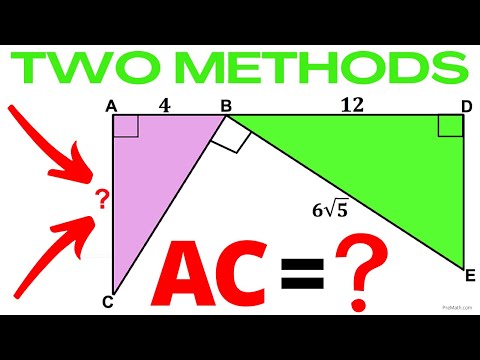
Can you find the Distance AC? | Two Easy Methods | Geometry Challenge
Navigation Challenge! Can You Find Angle X? | Two Simple Methods
Can you find area of the Green shaded Triangle? | (Two Methods) | #math #maths | #geometry
2 + 2 = 5 ॥ Proven with two methods ॥ Can you find the mistake? 2 + 2 equals 5
Two Methods Beautiful Equation Math Tricks Can You Find Value X? EP19 #mathematics
Find The Height 'h' In This Shape | Learn Two Easy Methods
Two Methods | Find the missing side length X | (step-by-step explanation) | #math #maths
Can you find X+Y=? | Two cool methods | (Trigonometry and Geometry) | #math #maths
Can You Find the Radius of the Blue Circle? Two Methods Explained
2 + 2 = 5 || proven with two methods || can you find the error || trikes
Two Methods To Find The Least Common Multiple Of Small Numbers ~ 6th Grade Tutor Me Sempai
Can you find the radius? | (Two Methods) | #math #maths | #geometry
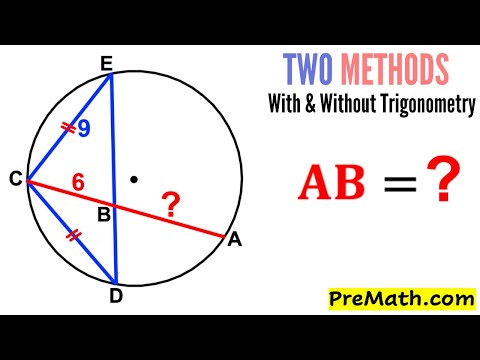
Two Methods: With & Without Trigonometry | Find the Length of AB & AC in this Triangle
Can you find area of the Blue shaded Rectangle? | (Two Methods) | #math #maths | #geometry
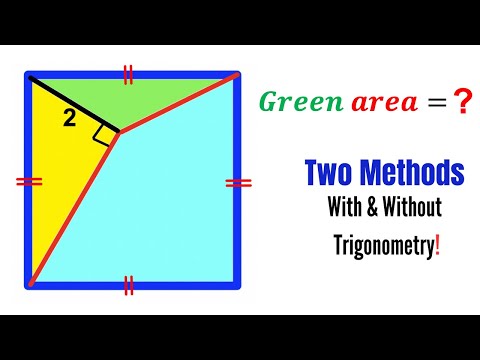
Can you find area of the Green shaded Rectangle? | (Two Methods) | #math #maths | #geometry
Комментарии
 0:09:35
0:09:35
 0:03:51
0:03:51
 0:04:09
0:04:09
 0:08:40
0:08:40
 0:11:48
0:11:48
 0:11:12
0:11:12
 0:14:33
0:14:33
 0:00:11
0:00:11
 0:03:44
0:03:44
 0:09:43
0:09:43
 0:04:58
0:04:58
 0:09:08
0:09:08
 0:02:54
0:02:54
 0:09:05
0:09:05
 0:08:21
0:08:21
 0:08:44
0:08:44
 0:11:44
0:11:44
 0:13:04
0:13:04
 0:04:32
0:04:32
 0:10:40
0:10:40
 0:09:46
0:09:46
 0:14:54
0:14:54
 0:09:59
0:09:59
 0:10:51
0:10:51