filmov
tv
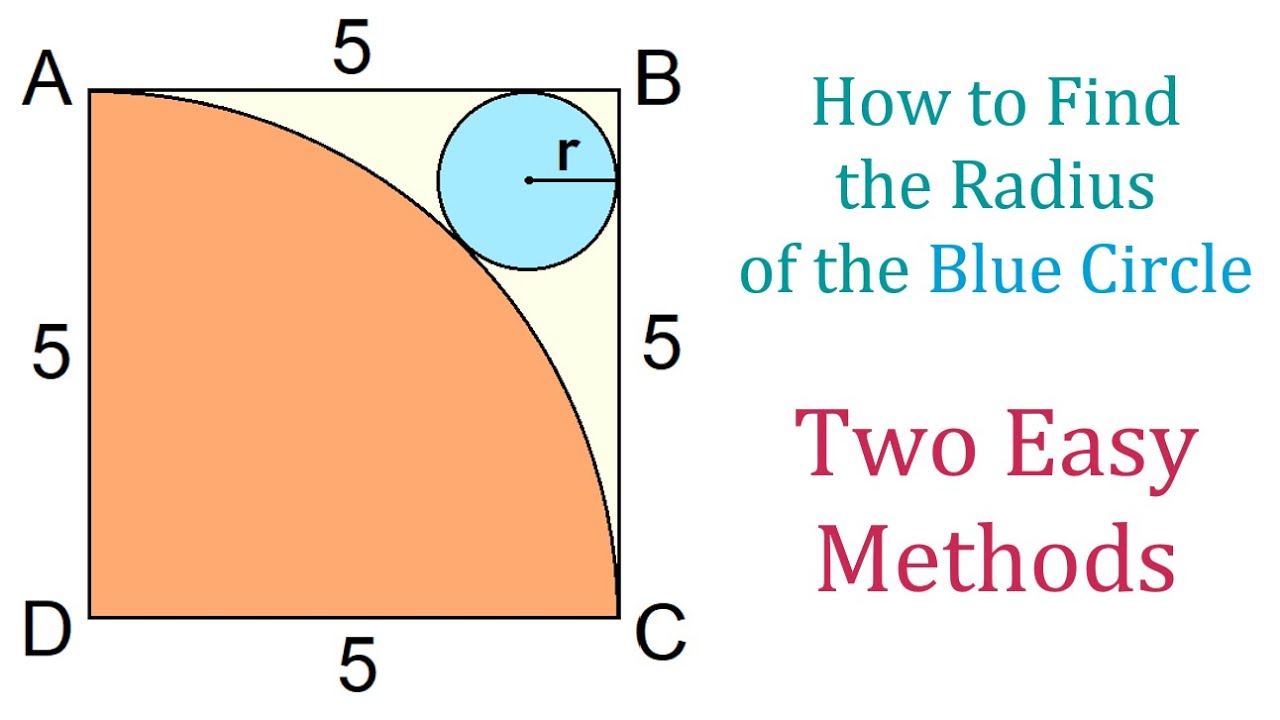
Can You Find the Radius of the Blue Circle? Two Methods Explained
Показать описание
The two methods involve the Pythagorean Theorem, Quadratic Formula, and rationalizing the denominator using the conjugate.
Finding the Radius of a Circle Given the Diameter | Math with Mr. J
How to Find Radius of Circle from Area | Geometry
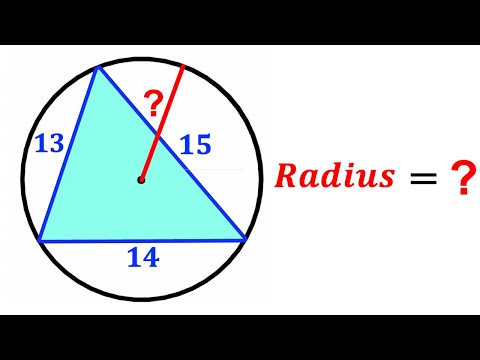
Can you find the Radius of the circle? | (Triangle inscribed in a circle) | #math #maths
Find the Radius
How to Find The Radius Of Circle
Can You Find the Radius of the Circle? | Quick & Simple Tutorial
Can you find the Radius of the Circle? | Step-by-Step Explanation
Finding the radius when given the circumference
How to find an expression for the area between the circles? #shorts #maths
Can You Find the Radius of the Small Purple Circle? | Quick & Easy Explanation
What is the Radius of a Circle? | What is the Diameter of a Circle? | Radius and Diameter Explained
Can you apply three methods to find the Radius of the circle? | Important Geometry skills explained
Given the area of a circle find the radius
Learn 3 Different Methods to Find the Radius of a Circle | In-Depth Explanation
Finding the radius of a circle
How to Find the Radius of a Circle (Circumference Given)
Can you find the radius of this inscribed circle??
How to Find the Radius of a Circle given the Area
Key Skill - Find the radius of a circle given its area.
Finding the Diameter of a Circle Given the Radius | Math with Mr. J
Can you find the Radius of the circle? | (Scalene triangle) | #math #maths | #geometry
How to find the center and radius of a circle in standard form
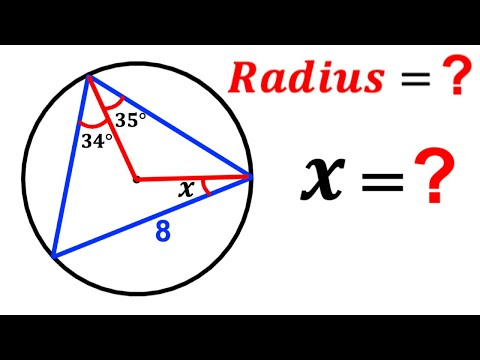
Can you find the Radius and the angle X? | (Inscribed triangle) | #math #maths | #geometry
How to calculate the RADIUS, DIAMETER and the CIRCUMFERENCE of a circle
Комментарии
 0:02:56
0:02:56
 0:05:03
0:05:03
 0:10:25
0:10:25
 0:03:25
0:03:25
 0:02:05
0:02:05
 0:09:15
0:09:15
 0:06:45
0:06:45
 0:01:51
0:01:51
 0:01:58
0:01:58
 0:06:32
0:06:32
 0:03:52
0:03:52
 0:11:12
0:11:12
 0:02:20
0:02:20
 0:17:28
0:17:28
 0:01:40
0:01:40
 0:01:02
0:01:02
 0:15:45
0:15:45
 0:02:54
0:02:54
 0:03:03
0:03:03
 0:03:08
0:03:08
 0:09:29
0:09:29
 0:05:06
0:05:06
 0:08:51
0:08:51
 0:08:14
0:08:14