Completing the Square - Solving Quadratic Equations │Algebra

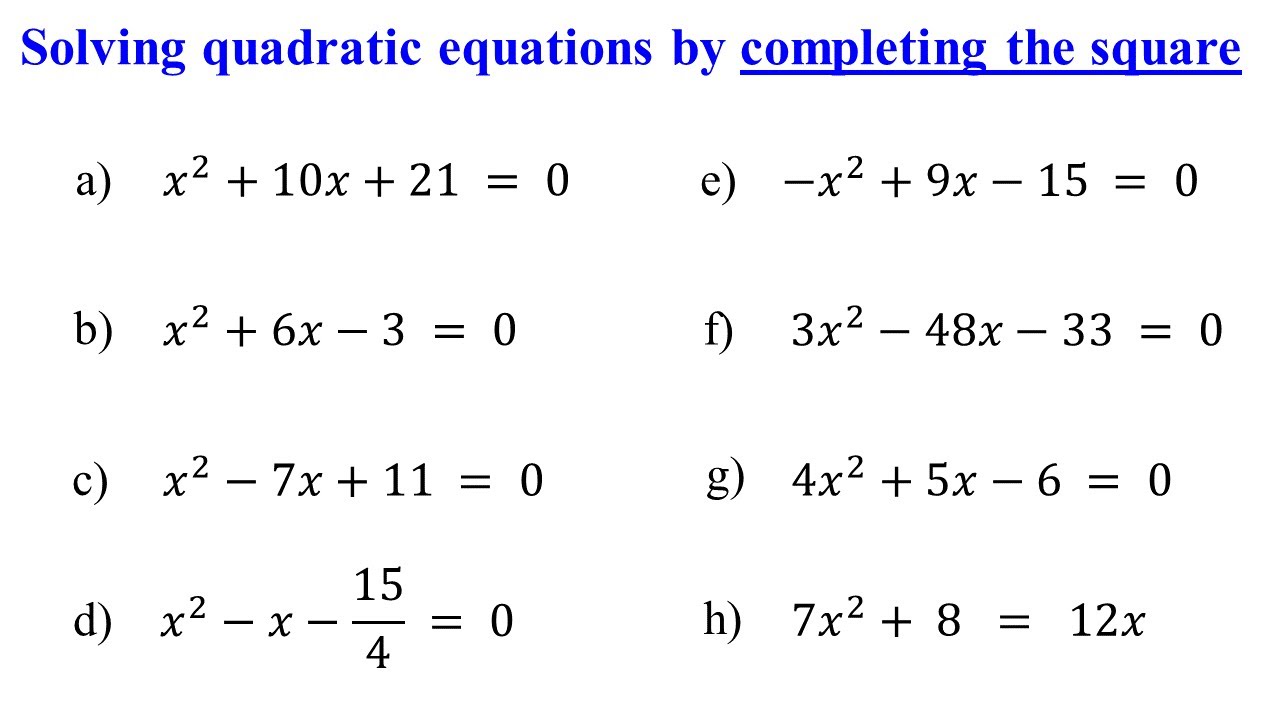
This algebra math tutorial explains how to solve quadratic equations by completing the square. It covers examples with leading coefficient of one, leading coefficient different from one, non-standard form equations and equations with no real solutions or complex/imaginary solutions. It also shows how to verify/check the solutions.

quadratic equations, completing the square, solving quadratic equations by completing the square, how to solve quadratic equations by completing the square, quadratic equations by completing the square , solving quadratic equations with a leading coefficient of one, solving quadratic equations with a leading coefficient different from one, solving quadratic equations with negative leading coefficient, how to solve quadratic equations , solving quadratic by completing the square, solving quadratic equations by completing the square with checking, solving quadratic equations by completing the square step by step, solving quadratic equations using completing the square, how to find the roots of a quadratic equation, how to find the zeros of a quadratic equation, algebra , gotutor math ,

[scottcooper]

Awesome detailed explanation of completing the square! For me, I like to move C to the right side of equation as my 1st step and then make A = 1 by dividing by A. If C is on the right side 1st, then when you divide all of the terms by A, you can eliminate dividing 0/A on the right side. I Really liked the animations for solving the problems. Probably the best video I have seen for solving quadratic equations. Most videos show solving quadratic equations by using the quadratic formula. That really does not explain what is going on. After all, the quadratic formula is derived by completing the square. Excellent Work!

[mabormalualmalual]

I'm absolutely pleased with the way you solve your problems, very fast, accurate and giving a variety of examples to make the learners understand the concept more better.THANKS

[Hokiebird]

Just a heads up, any time you don’t end up with a square root in the answer, the original equation could have been factored. While the point of the exercises was completing the square, part g can also be solved by factoring. This equation just so happens to be a good example of a little trick called “British Factoring” which can be used when the leading coefficient is not 1. In ax^2+bx+c, multiply a by c. Then write your factored form starting with 4x in both sets of parentheses.

(4x + #)(4x + #)=0

Now treat c as if it is -24, not -6. 8* -3 = -24, and 8-3=5

Enter

(4x+8)(4x-3)=0

And now remove a factor of 4 from 4x+8. Basically, you put an extra factor of 4 in when you wrote 4x for both factors, so you need to take a factor of 4 out. This trick is nice when it works, because it takes the guesswork out of whether you should have 4 and 1 as your coefficients, or 2 and 2.

Now you get (x+2)(4x-3)=0

Which of course gives the same answer as given at 12:41, just in a different way.

[cnxcnx]

Fantastique and clear explanation thank u

[shahidsamim]

Grate. It's a very informative and easy method. Thanks a lot.

[bkp_s]

Your both instructions gladly followed by me (liking and subscribing )without any delay.

[paola-lvor]

This really helped me tysm I didn’t find a video that explained the same way as my teacher

[PriscillaAyen-gn]

Thanks you are the best teacher but please be slow

[shannonmcdonald]

I remember when these were difficult... now i do them in my head.

[thokozanilukhele]

(x+9)(x-8)=0...
If A•B = 0.then.A/B = 0
x=-9 x=8

[londekadlamini]

I didn't understand the last part. Where did you get _5 and _2

[christophermalex]

Slov this. ײ +× - 72 =0. Solve in factorization method please